JEE Advanced Electrostatics (Non-Conductors) 2
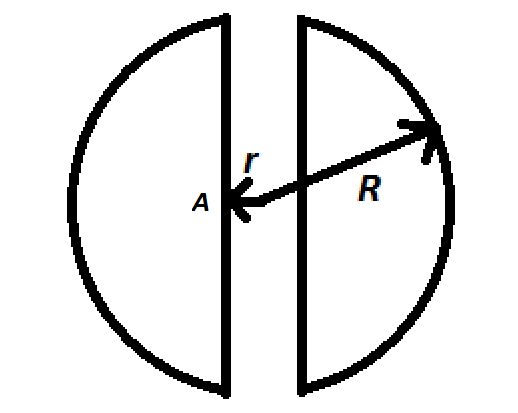 A cylindrical portion of radius
is removed from a solid sphere of radius
and uniform volume charge density
in such a way that the axis of the hollow cylinder coincides with one of the diameters of the sphere.
. Then the electric field at point
is
A cylindrical portion of radius
is removed from a solid sphere of radius
and uniform volume charge density
in such a way that the axis of the hollow cylinder coincides with one of the diameters of the sphere.
. Then the electric field at point
is
Assumptions
- is a unit vector to the right
This is a part of My Picks for JEE Advanced 2
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Simple Super Position Principle !
Well Pranjal I suggest You To mention X-Y direction's .