Just A

Logic Level 1

A A A A A A + A 1 0 0 \begin{array} { l l l l } & A & A \\ & A & A \\ & & A \\ & & A \\ + & & A \\ \hline 1 & 0 & 0 \end{array}

What is A A ?

2 4 6 8 0

View wiki
Chung Kevin by Chung Kevin , 45, Australia

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

15 solutions

Venkatachalam J
Mar 27, 2017

43 Helpful 0 Interesting 0 Brilliant 0 Confused

why 10? in the beginning the only thing in my mind was A times A = A square so 2(a)^2 + 3 a = 100 but got confused a is 10 a how did you find that

Joanna Demee - 4 years, 2 months ago

Log in to reply

Why 10 in the beginning?

Alshaimaa Elgenbihy - 4 years, 2 months ago

Log in to reply

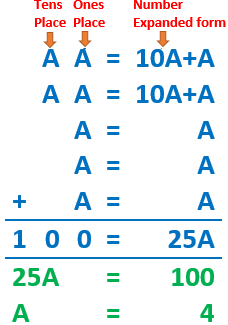
Because it is in the tens place. AA can be written as = (A x10) +A =10A + A in the expanded number form using base 10. – Venkatachalam J

Venkatachalam J - 4 years, 2 months ago

It is in the number expanded form. "A" is a single digit. In "AA" the left most "A" is in tens place and right most is in the once place. Therefore it is written as "10A+A". If you check with any two digit number you will get it quickly. For example the number "27" can be written in the expanded form (2x10)+7=20+7.

Venkatachalam J - 4 years, 2 months ago

Nice. I had once seen a similar cryptogram where there were 8 8's that made a 1000.

Agnishom Chattopadhyay - 4 years, 2 months ago

Based on the comments I updated the required modification. I hope, it will clear and understandable.

Venkatachalam J - 4 years, 2 months ago
Zee Ell
Mar 22, 2017

( 10 A + A ) + ( 10 A + A ) + A + A + A = 25 A (10A+A) +(10A+A) + A+A + A = 25A

25 A = 100 25A = 100

A = 4 A = \boxed {4}

40 Helpful 0 Interesting 0 Brilliant 0 Confused

25 plus 4 equals 100

Prabhbir sohi - 4 years, 2 months ago

Log in to reply

😂 25 + 4 = 29

25 × 4 = 100

Zee Ell - 4 years, 2 months ago
Ahmed Amir
Mar 27, 2017

5A + 20A = 100

A = 4

10 Helpful 0 Interesting 0 Brilliant 0 Confused

@Agnishom Chattopadhyay You can represent numbers like 55, 31, 72, etc... as 5 * 10+5, 3 * 10+1 and 7 * 10+2 respectively. Try and think how that fact applies to this problem.

Ebuka Akubilo - 4 years, 2 months ago

Could you explain how you got the 5 and 20 in 5A + 20A?

Agnishom Chattopadhyay - 4 years, 2 months ago

To a novice, there is nothing obvious about this solution. No idea what the different columns represent, how to interpret them, or how they interact. Back to 2+2=4, my favorite.

Carson Foard - 4 years, 2 months ago
Mervyn Rice
Mar 29, 2017

44+44+4+4+4= 100

3 Helpful 0 Interesting 0 Brilliant 0 Confused

Yes you have shown that A=4 is a solution, but is it possible that there are other possible solutions as well? Why or why not?

Pi Han Goh - 4 years, 2 months ago
Dominic Dharam
Apr 2, 2017

3A + 11A +11A =100 A (3+11+11) =100 A = 100/25 = 4

2 Helpful 0 Interesting 0 Brilliant 0 Confused
Galen Buhain
Apr 2, 2017

5(a) + 20(a) = 100 a = 4

1 Helpful 0 Interesting 0 Brilliant 0 Confused
Guy-Philippe Goth
Mar 29, 2017

11xA+11xA+3xA=100

25A=100

A=4

1 Helpful 0 Interesting 0 Brilliant 0 Confused
Guillermo Zamudio
Mar 28, 2017

This is assuming that you have a base 10 system. if the base was0, the A could also be zero. which is a silly problem ut points out that a second solution is real, depending on the base used. A=b *2/(2 b+5) b being your base.

1 Helpful 0 Interesting 0 Brilliant 0 Confused

I like your thinking :)

If the base is a positive integer, what other solutions can we have (where A is also an integer)?

Chung Kevin - 4 years, 2 months ago
Avianna Gay
Apr 2, 2017

Just by glancing at it, we know that 0 and 2 are automatically out , because the numbers they yield are TOO SMALL:

0 ----> (00+00=0)

2----> (22+22+2+2+2=44+6<100)

In the same instance, we also know that that 6 and 8 are automatically out due to the fact that the numbers that they yield, are too LARGE to begin with. Doubling the double digit numbers, alone, would yield a number GREATER than 100:

6 ---> (66+66>100)

8 ---> (88+88>100).

Therefore, the only logical answer must be 4:

4 ---> 44+44+4+4+4=88+12= 100

0 Helpful 0 Interesting 0 Brilliant 0 Confused
Dibakar Sunny
Apr 2, 2017

0 Helpful 0 Interesting 0 Brilliant 0 Confused
冠璋 陳
Apr 1, 2017

Why would 11% get this wrong?

0 Helpful 0 Interesting 0 Brilliant 0 Confused
Tarick Hill
Mar 31, 2017

i got the right answer but i did it a completely different way i took it as an a and another a and since they were right next to each other i took the 2 a's and put it as 44 and 44 times 2 is 88 and 88 + 12 = 100

0 Helpful 0 Interesting 0 Brilliant 0 Confused

Sorry, I did not understand what you meant.

Agnishom Chattopadhyay - 4 years, 2 months ago
Gabby Dackers
Mar 30, 2017

A=4 . 6 and 8 are too high a value/s, and 0 just equals 0. This leaves 2 and 4. However, A cannot be 2 because 22+22+2+2+2 will only equal 50. So it makes sense that when you double the value 2 to make 4, you double the result of the AA + AA + A + A + A, and so 50 becomes 100. Also. 2 lots of 44 (= to AA) equal 88, and 3 lots of 4 (= to A) equal 12. 88 + 12 = 100

0 Helpful 0 Interesting 0 Brilliant 0 Confused

Yeah, so out of all the options, A = 2 works, but can A be equal to 1,3,5,7, or 9 as well?

Pi Han Goh - 4 years, 2 months ago
Cassidy McGuire
Mar 29, 2017

I just went through each number. A = 2 isn't it as it would equal fifty A = 6 or 8 aren't it, as they would equal numbers over 100. A = 0 would just be 0 So A = 4

0 Helpful 0 Interesting 0 Brilliant 0 Confused

Yup, A=4 is correct. But is there a more systematic way to solve this without resorting to trial and error?

Pi Han Goh - 4 years, 2 months ago
Emily Lessmiller
Mar 28, 2017

just find the larger two and the last ones become easier. try all the numbers displayed to help. if AA=2, then it would total to 50, not 100. if you took 6, then you would find that wouldnt work, right away. 66+66=132, and thats over 100 already. plus, 0 added to itself makes 0, so thats not the answer. the answer is, AA=44, so 44+44= 88, and A+A+A= 12, (4+4+4=12) and 88+12=100. Its not that complicated. Isnt this supposed to be hard? I could have solved this in sixth grade, and right now I'm in eighth. Take that for a smart kid.

0 Helpful 0 Interesting 0 Brilliant 0 Confused

Yup, you have experimented for all the possible values of A, but is it possible to get the answer without experimenting with all these options?

Pi Han Goh - 4 years, 2 months ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...