Just an Angle!

Extend $PB$ and $AC$ , label the point of intersection as $E$ .

We have $\angle AEP=30^{\circ}$ , $\Rightarrow CE=6, \; AE=8$ , $\Rightarrow AP=\frac{8\sqrt{3}}{3}$ , $\Rightarrow PC=\sqrt{2^{2}+\left ( \frac{8\sqrt{3}}{3} \right )^{2}}=\frac{2\sqrt{57}}{3}=\sqrt{\frac{4\times 57}{9}}=\sqrt{\frac{76}{3}}$ .

Let $PC = x$ and $\angle APC = \theta$ , then using sine rule, we have:

$\begin{aligned} \frac{x}{\sin 90^\circ} = x & = \frac{2}{\sin{\theta}} = \frac{3}{\sin{(60^\circ - \theta)}} \\ \Rightarrow 2(\sin{(60^\circ - \theta)}) & = 3\sin{\theta} \\ 2\left(\frac{\sqrt{3}}{2}\cos \theta - \frac{1}{2} \sin \theta\right) & = 3 \sin \theta \\ \sqrt{3}\cos \theta - \sin \theta & = 3 \sin \theta \\ \sqrt{3}\cos \theta & = 4 \sin \theta \\ 3 \cos^2 \theta & = 16 \sin^2 \theta \\ 3 (1 - \sin^2 \theta ) & = 16 \sin^2 \theta \\ 3 & = 19 \sin^2 \theta \\ \Rightarrow \sin \theta & = \sqrt{\frac{3}{19}} \\ \Rightarrow x & = \frac{2}{\sin \theta} = \frac{2}{\sqrt{\frac{3}{19}}} = \sqrt{\frac{76}{3}} \\ \\ \Rightarrow A + B & = 76 + 3 = \boxed{79} \end{aligned}$

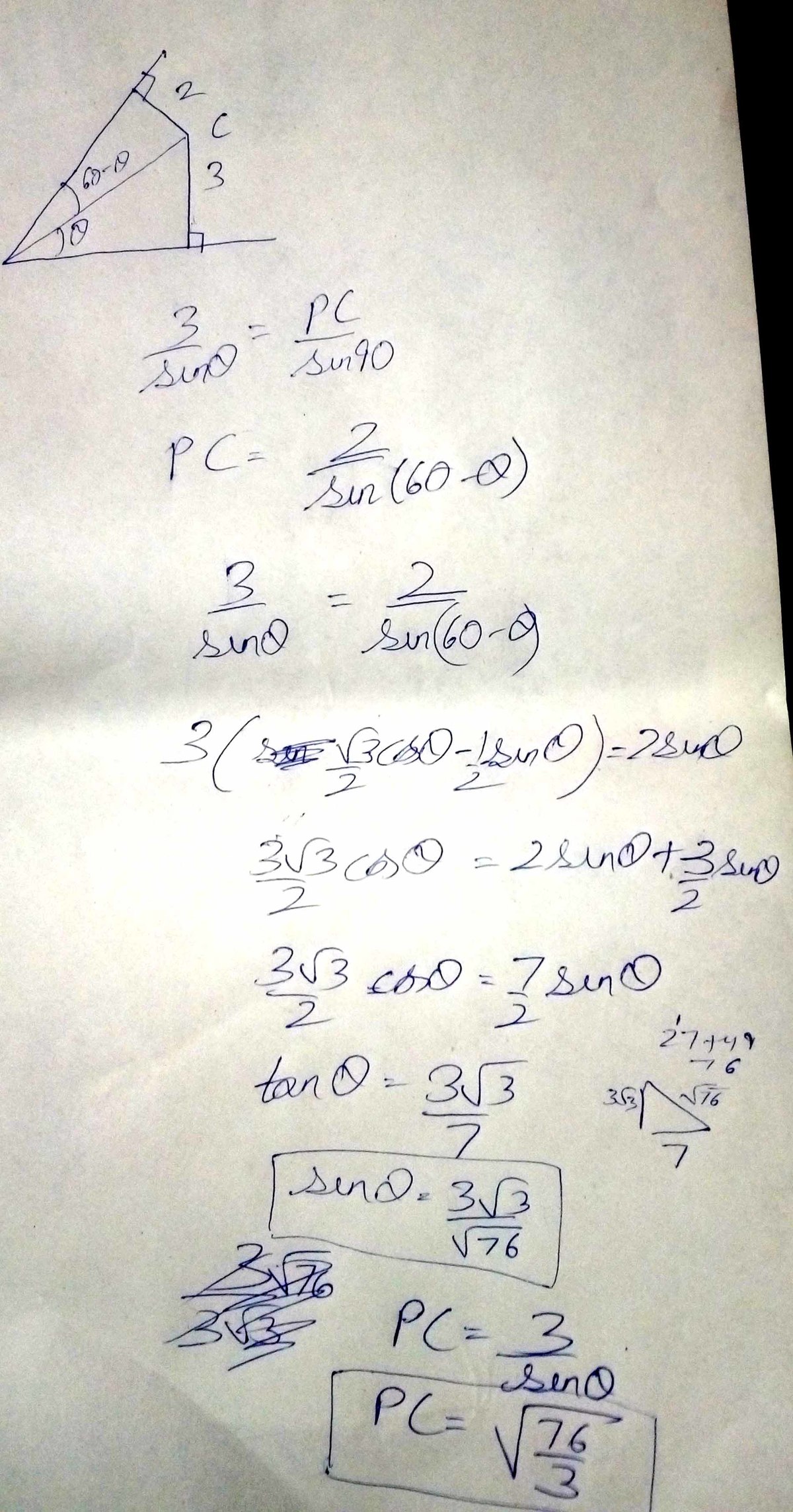
I used sine rule in the very first step

Extend the line BC to intersect extension of line AP at point D.

DAC=30 degrees

CD=2/sin(60)=4

BP=7tan(30)=7/sqrt(3)

CP^2=BP^2+BC^2

CP=sqrt(76/3)

No gimmicks ;)

$P = (0,0)$ $C = (x, 3)$ $AP : y = \tan \frac{\pi}{3} x \implies \sqrt{3}x - y = 0$ $\frac{ |\sqrt{3}x - 3| }{\sqrt{ \sqrt{3}^2 + 1^2} } = \frac{|\sqrt{3}x - 3|}{2} = 2 \implies |\sqrt{3}x - 3| = 4$ $\implies x = \frac{7}{\sqrt{3}} \text{ Other solution is extraneous}$ $CP = \sqrt{x^2 + 9} = \sqrt{\frac{49}{3} + 9} = \sqrt{\frac{76}{3}} \implies 76 + 3 = \boxed{ 79 }$

Let the radius be x then x²+x²-2x.xcos120 = 4 + 9 - 2.2.3.cos120. Then x = sqrt(19/3). PC is diameter so PC = 2x = sqrt(76/3). Sry cant use latex im confused

C can be found as intersection of 2 lines:

1. Line1 parallel with AP at distance 2: y=sqrt(3).x-4
2. Line2 : y=3

So the x coordinate of C is 7/sqrt(3)

-> PC=sqrt(3^2 + (7/sqrt(3))^2)=sqrt(76/3)

Here I present a proof using coordinate geometry.

Let $P= (0,0)$ and $B=(b,0)$ , where $b>0$ . Then $O= (b,3)$ . Now

$Slope OA = \frac{-1}{Slope OB} = \frac{-1}{tan 60^\circ} = - tan 30^\circ$ ,

and $OA=2$ with $A$ to the left of $O$ so the point $A = (b-2cos 30^\circ , 3+ 2 sin 30^\circ )$ $= (b- \sqrt{3} , 4)$ .

Now, the slope of $PA$ is $tan 60^\circ = \sqrt{3}$ , so

$\frac {4}{b-\sqrt{3}} = \sqrt{3}$ , giving $b= \frac{7}{\sqrt{3}}$ .

Therefore $PC= \sqrt {b^{2} + 9} = \sqrt{\frac{49}{3} + 9} = \sqrt {\frac{76}{3}}$ , and so

$A+B = 76+ 3= 79$ .