KVPY #14

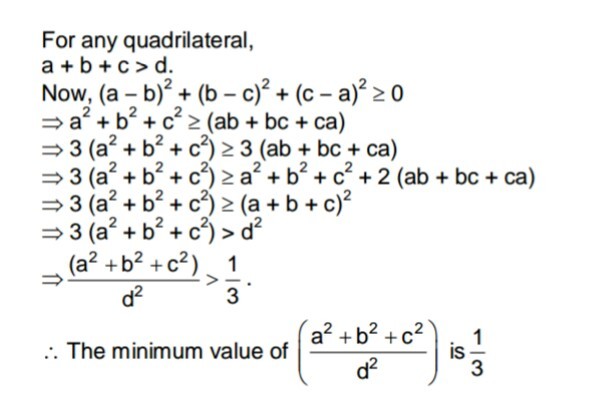
By Titu's Lemma ,

$(1 + 1 + 1)(a^2 + b^2 + c^2) \geq (a + b + c)^2$

$\Rightarrow a^2 + b^2 + c^2 \geq \dfrac{(a + b + c)^2}{3}$

$\Rightarrow \dfrac{a^2 + b^2 + c^2}{d^2} \geq \dfrac{(a + b + c)^2}{3d^2}$ $\quad \quad \cdots (1)$

---

Consider a quadrilateral $ABCD$ .

In $\triangle ABD$ , $AB + AD > BD$

In $\triangle BCD$ , $BD + CD > BC$

Adding the two gives $AB + AD + CD > BC$

This leads to the conclusion that sum of three sides in any quadrilateral is greater than the fourth side.

Following this , we have $a + b + c > d \Rightarrow (a + b + c)^2 > d^2 \Rightarrow \dfrac{(a + b + c)^2}{d^2} > 1$ $\quad \quad \cdots (2)$

---

By $(1) , (2)$ ,

$\dfrac{(a^2 + b^2 + c^2)}{d^2} > \dfrac13$

---

NOTE:

$\dfrac13$ is the infimum value and not the minimum value as this value cannot be attained.