Legebbles

You find some pebbles and arrange them in groups of increasing diamond-shapes as follows:

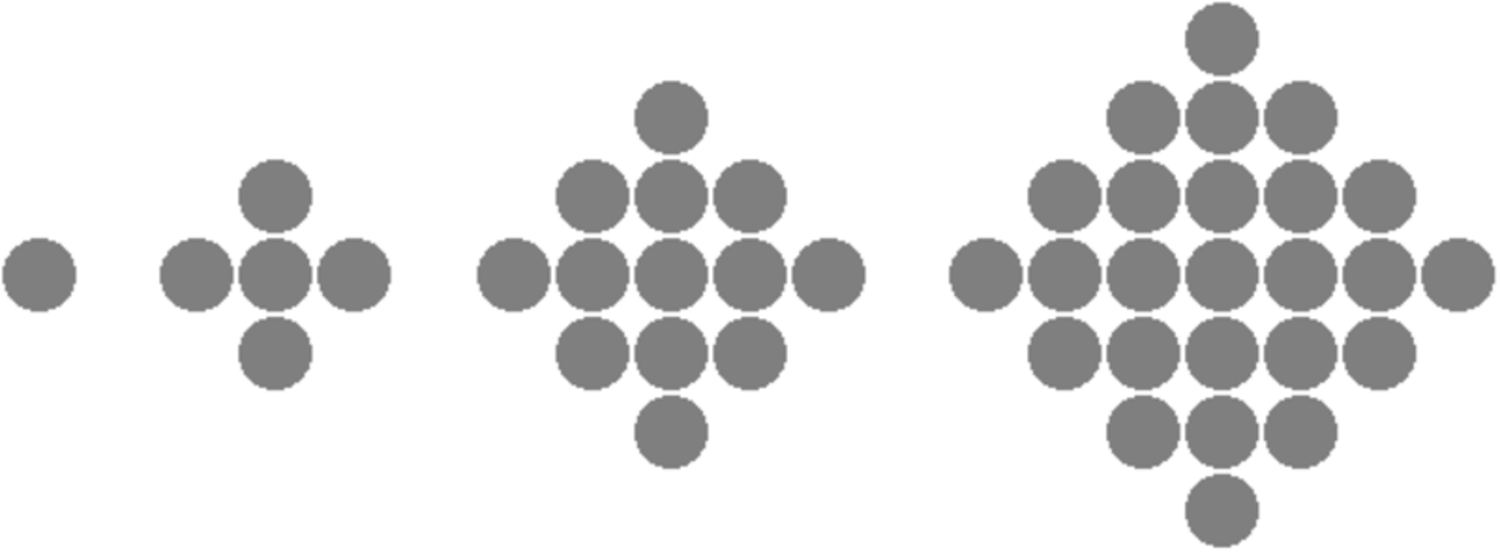
You notice that number of pebbles in the first grouping is a perfect $4^{\text{th}}$ power, ( $1^4=1$ ), but run out of pebbles before you can find another one.

If the pattern continues, how many other groupings would also use a $4^{\text{th}}$ power number of pebbles?

---

Inspiration: $(1)$ , $(2)$

Fun Question. What if we were to generalize this for any positive integer power?