Length of a line
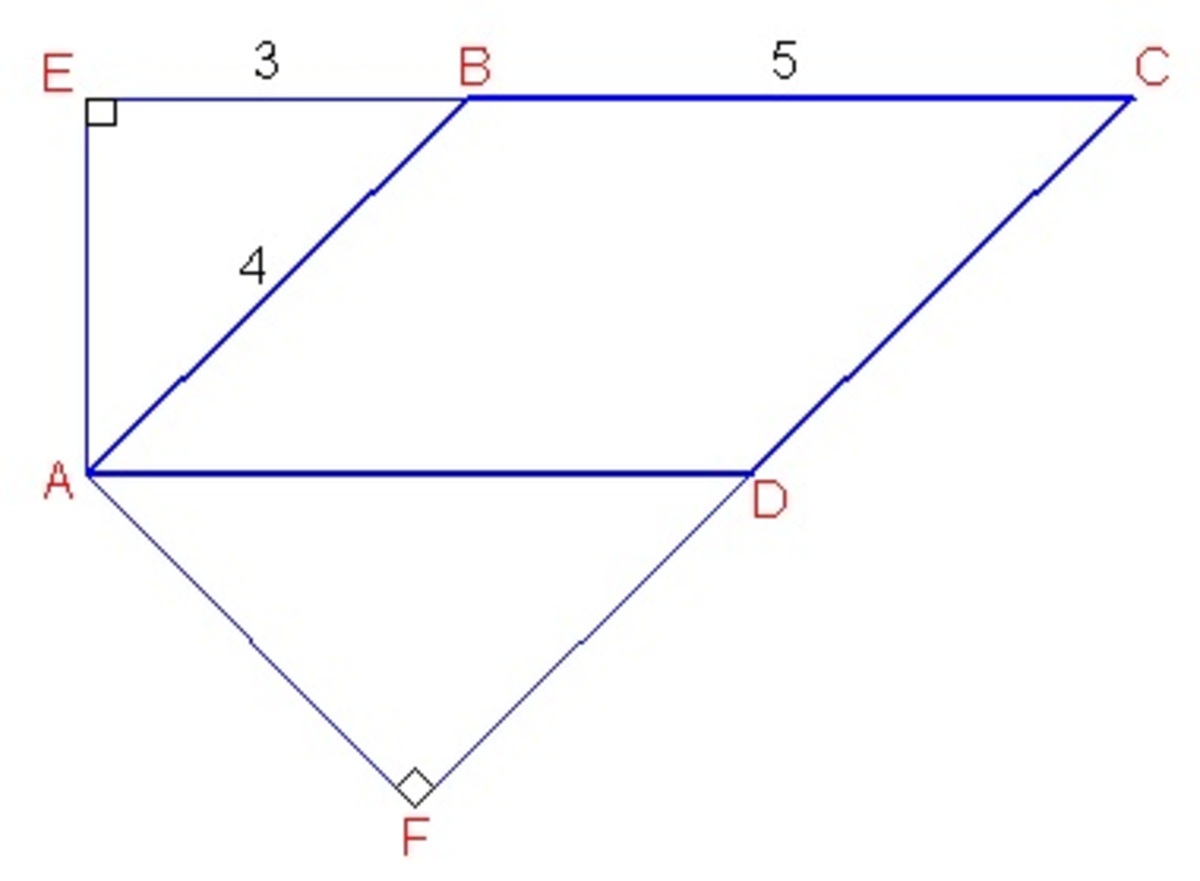 The figure above shows a parallelogram
A
B
C
D
.
C
B
extended meets
A
E
at a
9
0
∘
angle and
C
D
extended meets
A
F
at a
9
0
∘
angle. Given that
E
B
=
3
,
B
C
=
5
and
A
B
=
4
, find the length of
D
F
.
The figure above shows a parallelogram
A
B
C
D
.
C
B
extended meets
A
E
at a
9
0
∘
angle and
C
D
extended meets
A
F
at a
9
0
∘
angle. Given that
E
B
=
3
,
B
C
=
5
and
A
B
=
4
, find the length of
D
F
.
The answer is 3.75.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
For calculating the area if ABCD we have 2 options:
A D ⋅ A E and A B ⋅ A F .
Obviously these give the same result. Using Pythagoras we can find A E = 7 . So we have:
5 ⋅ 7 = 4 ⋅ A F .
So A F = 4 5 7 . Now using Pythagoras in triangle ADF we can calculate D F = 3 . 7 5 .
∠ E B A = 1 8 0 − ∠ A B C and ∠ A D F = 1 8 0 − ∠ A D C
However, ∠ A B C = ∠ A D C . Therefore, ∠ E B A = ∠ A D F .
It follows that, △ E B A ∼ △ A D F ( A . A . ) . So we have
A D D F = A B E B ⟹ 5 D F = 4 3 ⟹ D F = 4 3 ( 5 ) = 3 . 7 5