Let's colour!
Consider a grid of unit squares. Several squares are colored, such that each square has exactly one adjacent square (sharing a common side) that is colored.
What is he maximum number of squares that could be colored?
As an explicit example, if the length of the square's side is 4, we can color 6 squares:
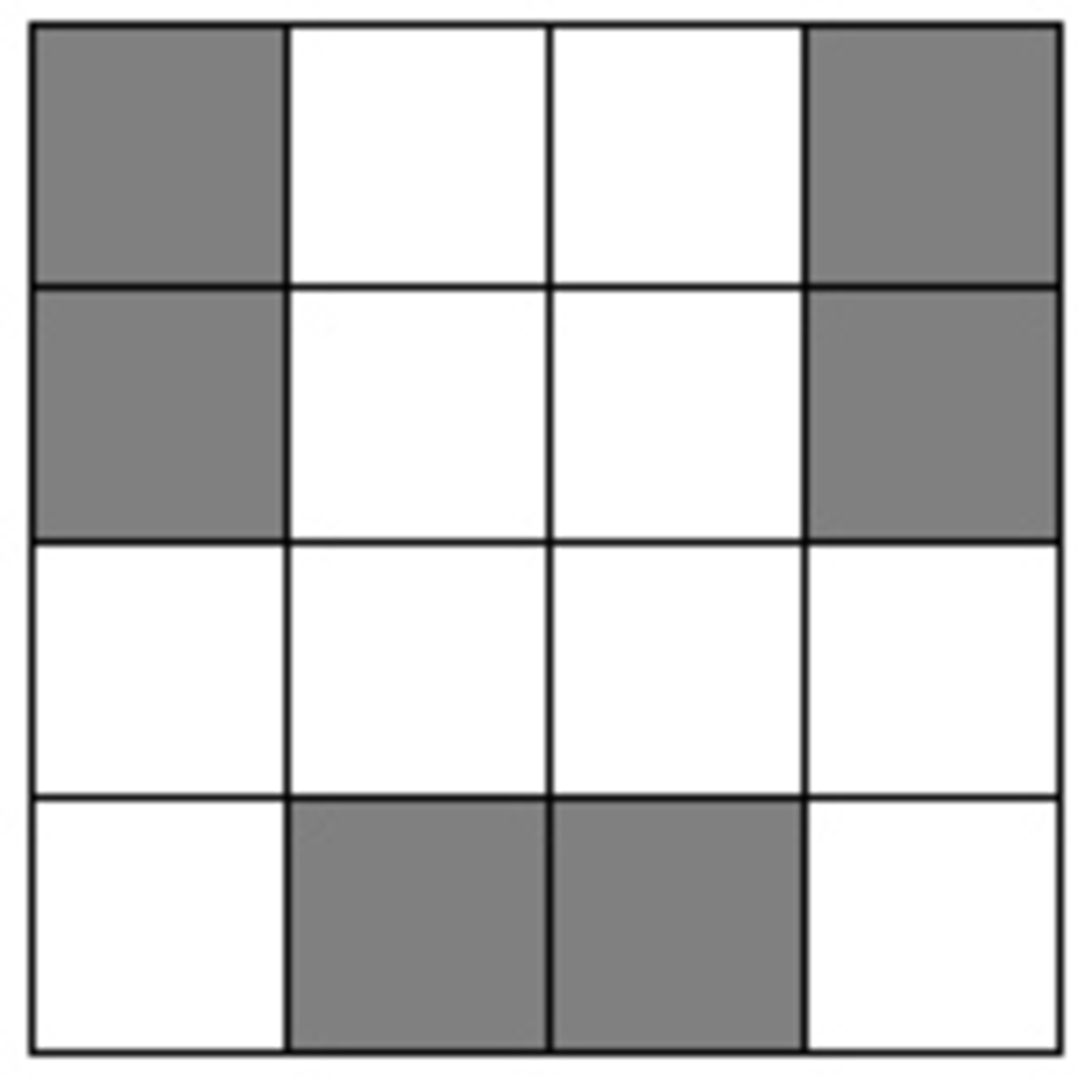
The answer is 1021110.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
Moderator note:
This is not a correct solution.
Consider the following Grid:
B W B W B W ...
B W B W B W ...
W B W B W B ...
W B W B W B ...
B W B W B W ... .................... Following this pattern, we see we can color half of the squares. This will cover a total of 2020*2020/2 = 2040200 squares.
I have proved that it is possible, but I have not proved it is the minimum. Jon Haussmann (https://brilliant.org/profile/jon-13o3qj/) however, has provided this with the following argument: "In any 2 by 2 square, at most two squares can be colored. This immediately gives us an upper bound of 2040200." (Because the grid is composed of 1010 2 by 2 squares, and on each square this argument applies)