Let's count!

A domino is a $2\times1$ or $1\times2$ tile.

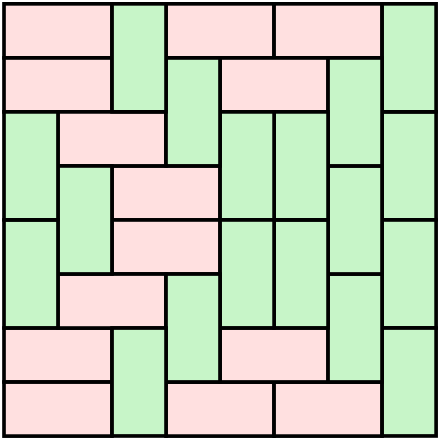
Let $N$ be the number of ways placing $100$ dominoes without overlapping on a $20\times20$ chessboard so that every $2\times2$ square contains at least two uncovered unit squares which lie in the same row or column.

Find the last 4 digits of $N$ .