Let's try some geometry

Geometry Level 3

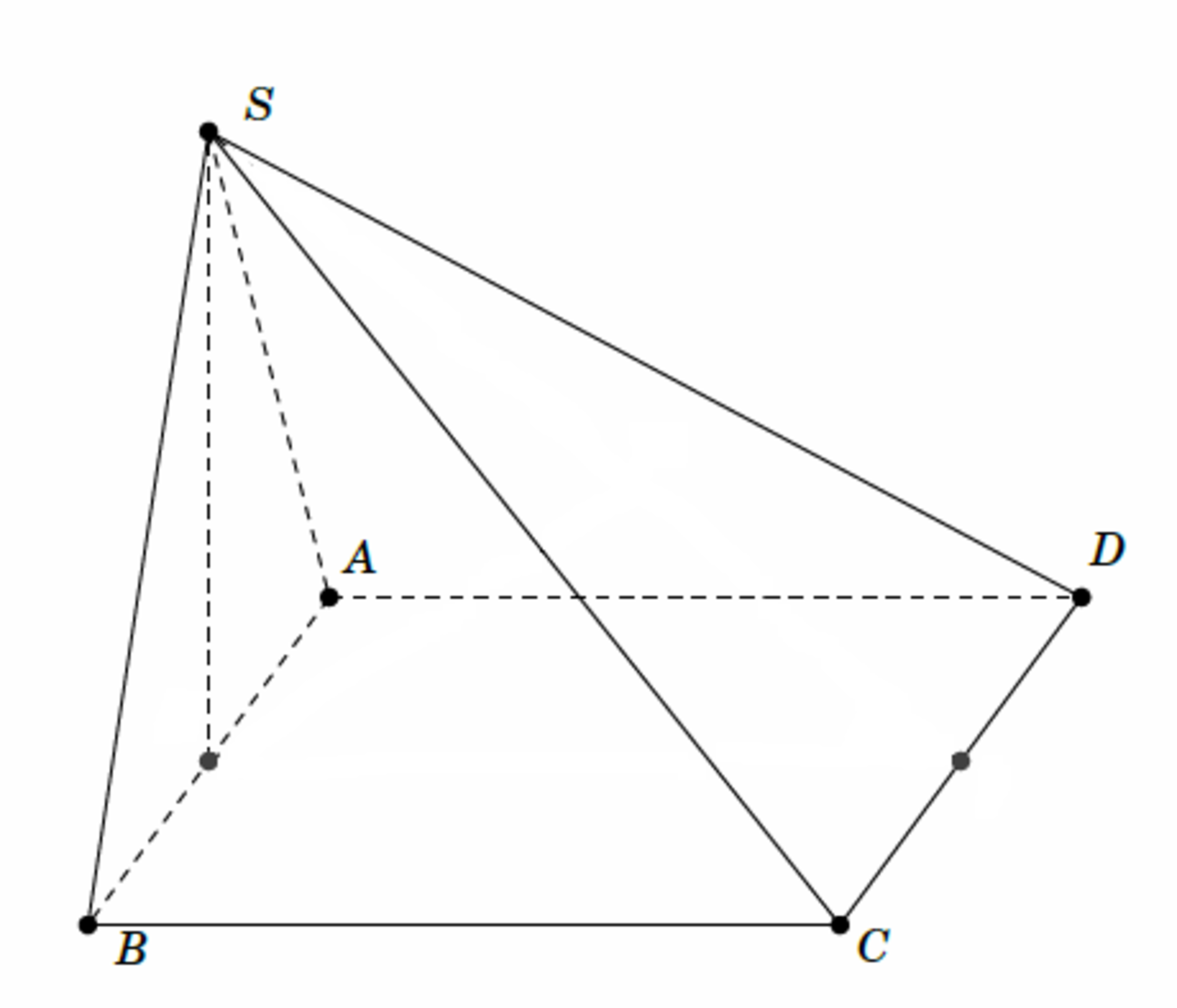
$S.ABCD$ is a pyramid with $S$ is the apex. The base $ABCD$ is a square. The face $SAB$ is an isosceles triangle with $SA=SB$ . $(SAB)\bot (ABCD)$ . The angle between plane $(SCD)$ and plane $(ABCD)$ is ${60}^{o}$ . The distance between plane $(SCD)$ and straight line $AB$ is $3$ . What is the volume of the pyramid $S.ABCD$ ?

The answer is 24.

1 solution

Marta Reece
Aug 20, 2017

If the side of the square is $a$ , then $\frac3a=\cos 30^\circ=\frac{\sqrt3}2$ , so $a=2\sqrt3$ and $a^2=4\times3=12.$

If the height of the shape is $h$ , then $\frac 3h=\sin 30^\circ=\frac12$ , so $h=2\times3=6$ .

Volume $=\frac13a^2h=\frac12\times12\times6=\boxed{24}$

Let's try some geometry

The answer is 24.

1 solution

0 pending reports