Limits!
x → 0 lim ( x 3 8 + x 1 − 2 x 1 )
If the limit above can be expressed as − b a , where a and b are coprime positive integers, find a + b .
The answer is 7.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
good solution.. how's mine?
Log in to reply
I'm not able to read it properly... Maybe you can type it in latex
Log in to reply
wait i'll correct it
check it now
Take x common from denominator and then rationalise the remaining expression to get the answer as − 4 8 1
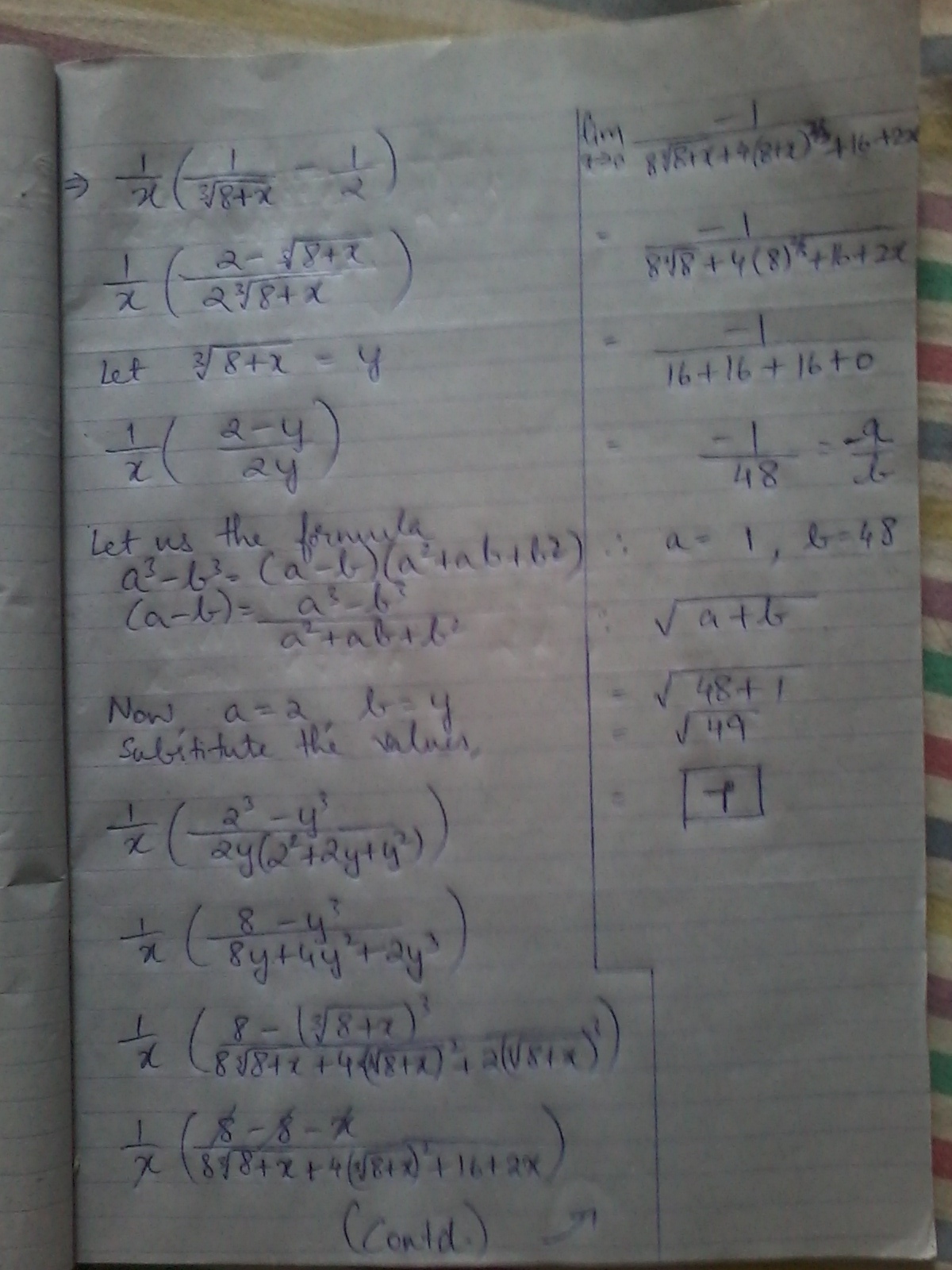
Lets call the limit as F .
F = x → 0 lim 2 x 2 3 8 + x 2 − 3 8 + x = x → 0 lim 2 x 1 − 3 1 + 8 x
Using Binomial approximation 3 1 + 8 x = 1 + 2 4 x as x → 0 and thus F = 4 8 − 1 ∴ 1 + 4 8 = 7