Line in Right Triangle
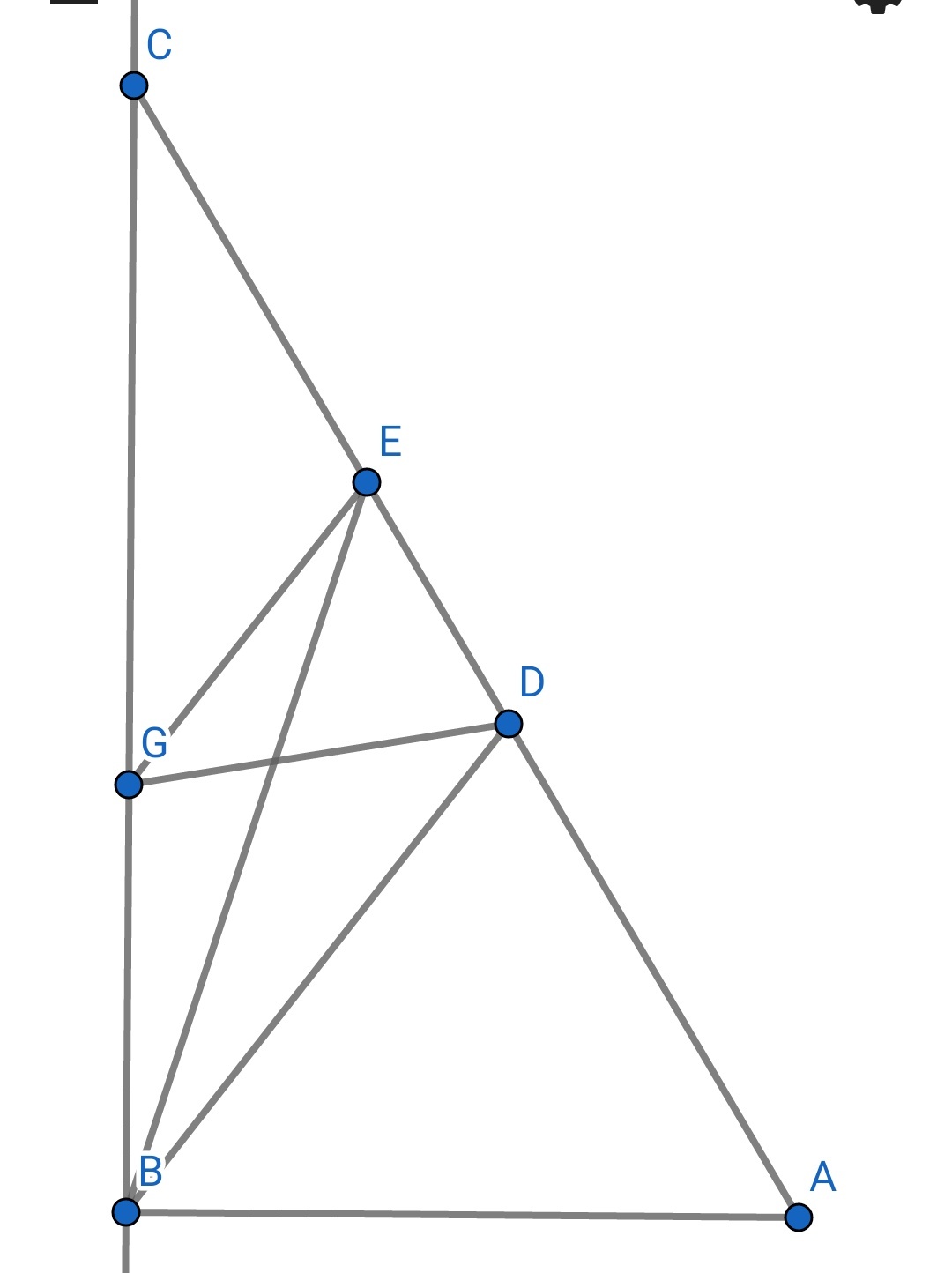 (The diagram is approximately correct but not drawn in scale.)
(The diagram is approximately correct but not drawn in scale.)
As shown in the diagram, there is a right triangle , which . Points lie on line such that . Point lies on line such that . If , find the length of .
The answer is 30.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Consider the circumcircle of triangle B D E as O . As ∠ A E B = ∠ A B D , we can conclude line A B is the tangent of circle O , which tangential point is B . As line B C is perpendicular to line A B at the tangential point B , we can conclude the circumcenter of triangle B D E lies on line B C .
As G E = G D , point G lies on the perpendicular bisector of line D E . As the circumcenter of triangle also must lies on perpendicular bisector of its side, and also G lies on line B C , we can conclude that G is the circumcenter of triangle B D E . Hence, B G = G E = 3 0 .