Locus of the centroid
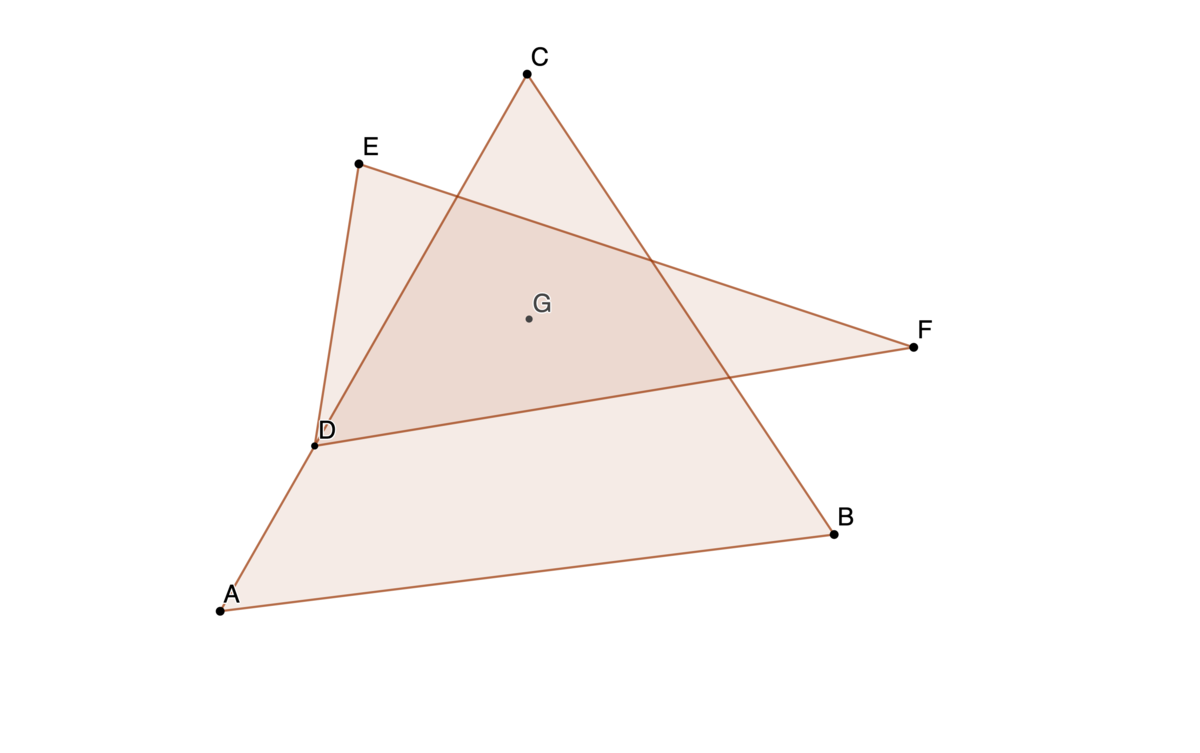 Point
lies on an edge of
. The centroid of
is
. Let
be the area of the locus of
as
traverses the boundary of
and let
be the area of
. If
, where
are coprime positive integers, submit
Point
lies on an edge of
. The centroid of
is
. Let
be the area of the locus of
as
traverses the boundary of
and let
be the area of
. If
, where
are coprime positive integers, submit
The answer is 10.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
With an obvious notation the position vector of the centroid G is g g − 2 1 ( e + f ) = 3 1 ( d + e + f ) = 3 1 [ d − 2 1 ( e + f ) ] so that the homothecy H ( M , 3 1 ) (enlargement with centre M and scale factor 3 1 ), where M is the midpoint of E F , maps D to G . Thus the locus of G is the image of the triangle A B C under the homothecy H ( M , 3 1 ) , and hence is a triangle similar to A B C , but of one third the linear dimension. Thus we deduce that L = 9 1 T , and hence T L = 9 1 , making the answer 1 + 9 = 1 0 .