Log and graphs
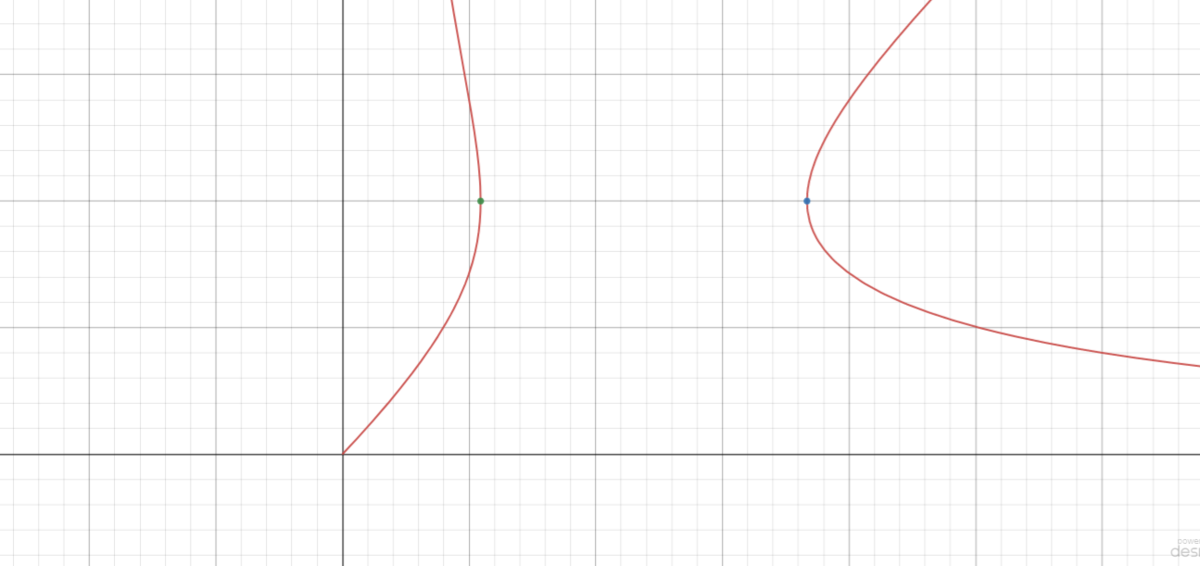 The graph above is of
, the equation is not graphable in between the two colored points.
The graph above is of
, the equation is not graphable in between the two colored points.
If length of the line segment joining the points is find
The answer is -0.5.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Rearranging, ln 2 ( y ) = ln 2 ( x ) − e 1 ≥ 0 , so ln 2 ( x ) ≥ e 1 , which means ln ( x ) ≤ − e 1 and ln ( x ) ≥ e 1 , or in other words, x ≤ e − e 1 and x ≥ e e 1 , so the x -coordinates of the two colored points are x 1 = e − e 1 and x 2 = e e 1 .
Substituting either of these x values back into ln 2 ( y ) = ln 2 ( x ) − e 1 solves to y = 1 , so the distance between the two colored points is e e 1 − e − e 1 .
Therefore, a = e 1 , and ln a = − 2 1 = − 0 . 5 .