Lossless Power Transfer
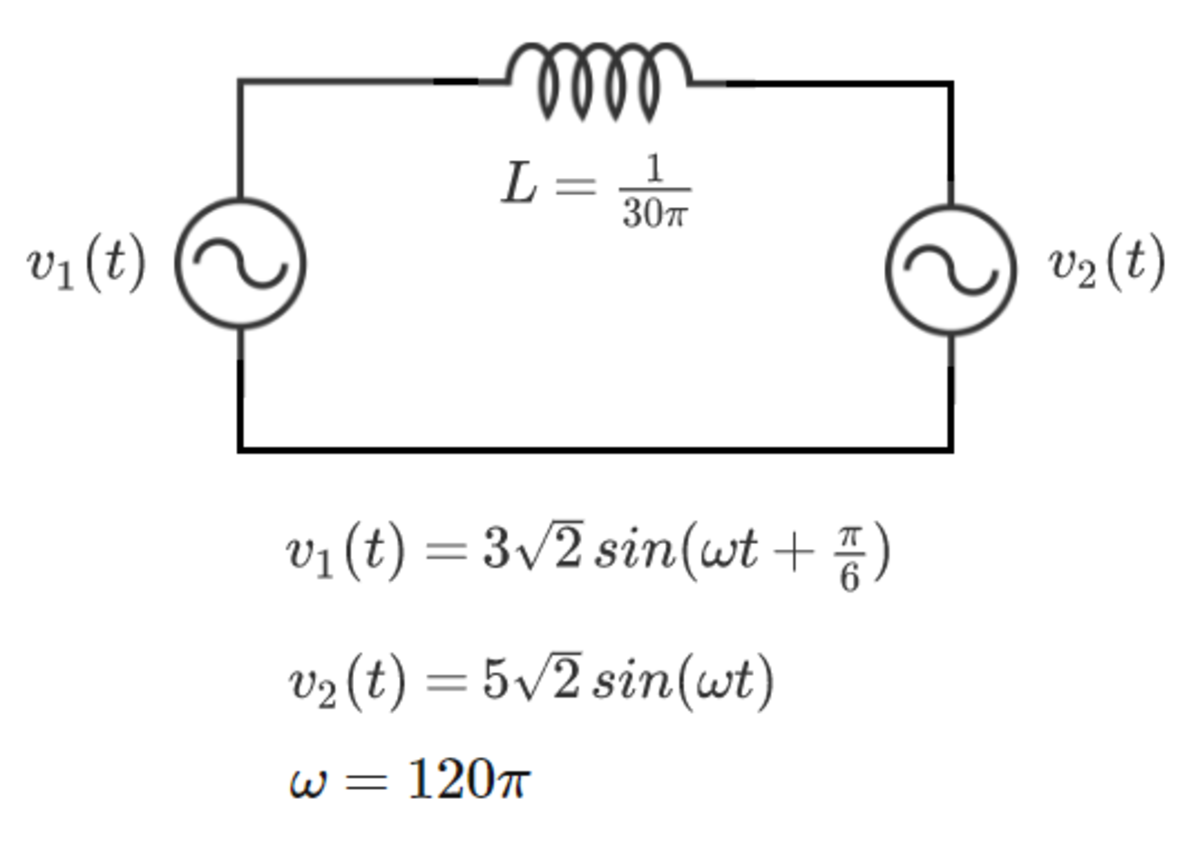
What is the magnitude of the average power transferred from Source 1 to Source 2?
Note: The averaging period is an integer number of sinusoidal cycles. Assume no DC content in the circuit. Assume standard physical units for all quantities.
The answer is 1.875.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
A very important equation in electric power system analysis is the so-called "power angle equation". It relates the power flow across a largely reactive transmission line to the RMS voltage magnitudes at the two ends, the voltage angles at the two ends, and the inductive reactance in between. Suppose the voltage at one end of the line is V 1 ∠ θ 1 , the voltage at the other end is V 2 ∠ θ 2 , and the inductive reactance is X . The power flow is:
P = X V 1 V 2 s i n ( θ 1 − θ 2 )
Plugging in numbers from this problem:
P = 4 3 × 5 s i n ( π / 6 − 0 ) = 8 1 5 = 1 . 8 7 5
We can also simulate the circuit in the time-domain and integrate the instantaneous power over an integer number of cycles, and then divide by the same number of cycles. This yields the same result, but is somewhat more involved.