Low-Speed Lorentzian Physics
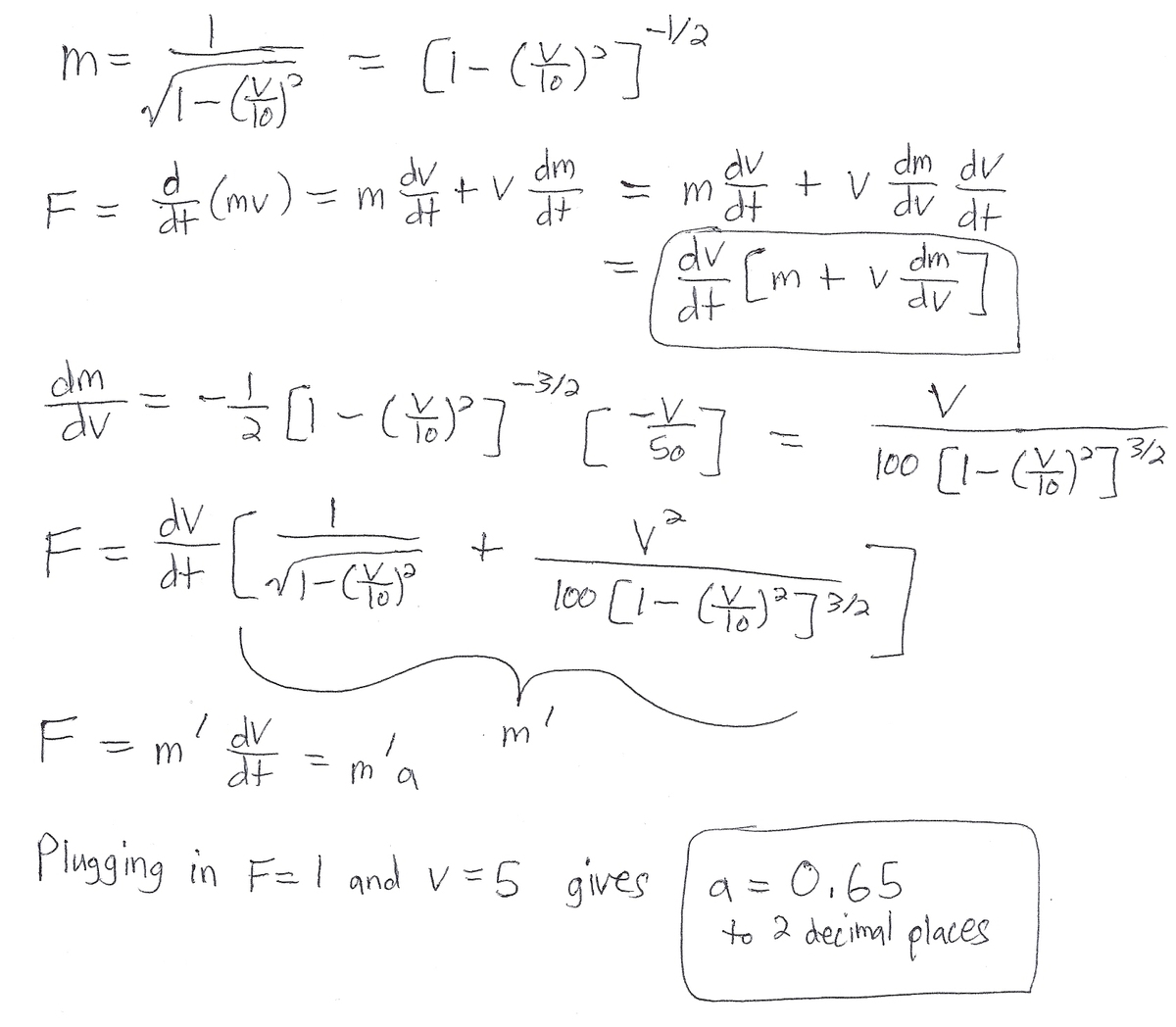
Consider a particle with rest-mass ( m 0 = 1 k g ) and velocity v . Suppose we're in an alternate universe in which the "cosmic speed limit" is only 1 0 m / s , and that the particle's mass varies with its velocity in the following way:
m = 1 − ( 1 0 v ) 2 m 0
In this framework, Newton's Second Law takes a more general form ( F denotes the net force applied to the particle):
F = d t d ( m v )
The particle is initially at rest before a constant 1 N force is applied to it. At the instant at which v = 5 m / s , what is the particle's acceleration (in m / s 2 ) (to 2 decimal places)?
Note: All physical quantities are in standard SI units.
The answer is 0.65.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Let c = 1 0 m / s
F = m 0 d v d ( 1 − c 2 v 2 v ) d t d v =
m 0 ∗ ( c 2 ∗ ( 1 − c 2 v 2 ) 2 3 v 2 + ( 1 − c 2 v 2 ) 2 1 1 ) d t d v =
( 1 − c 2 v 2 ) 2 3 m 0 d t d v ⟹
d t d v = m 0 F ∗ ( 1 − c 2 v 2 ) 2 3
Using v = 5 m / s , F = 1 N , and m 0 = 1 K g with c = 1 0 m / s ⟹
d t d v = 8 3 3 = . 6 5 m / s 2 to two decimal places.
We are given that mass of an object varies with its velocity as: m = 1 − v 2 / c 2 m 0 Also we know that force is rate of change of momentum i.e. F = d t d m v So we write force as: F = d t d 1 − v 2 / c 2 m 0 v = ( 1 − v 2 / c 2 ) 3 / 2 m 0 d t d v replacing d t d v = a ,We get a = m 0 ( 1 − v 2 / c 2 ) 3 / 2 × F Substituting, v = 5 m s − 1 F = 1 N m = 1 k g c = 1 0 m s − 1
We get a = 0 . 6 5 m / s 2