Lune comes with fun

Geometry Level 3

To circles with center O , C O,C are drawn with radius 5 , 3 5,3 respectively such that they intersects at points A , B A,B and A B ‾ \overline{AB} becomes the diameter of the smaller circle as shown in the figure.

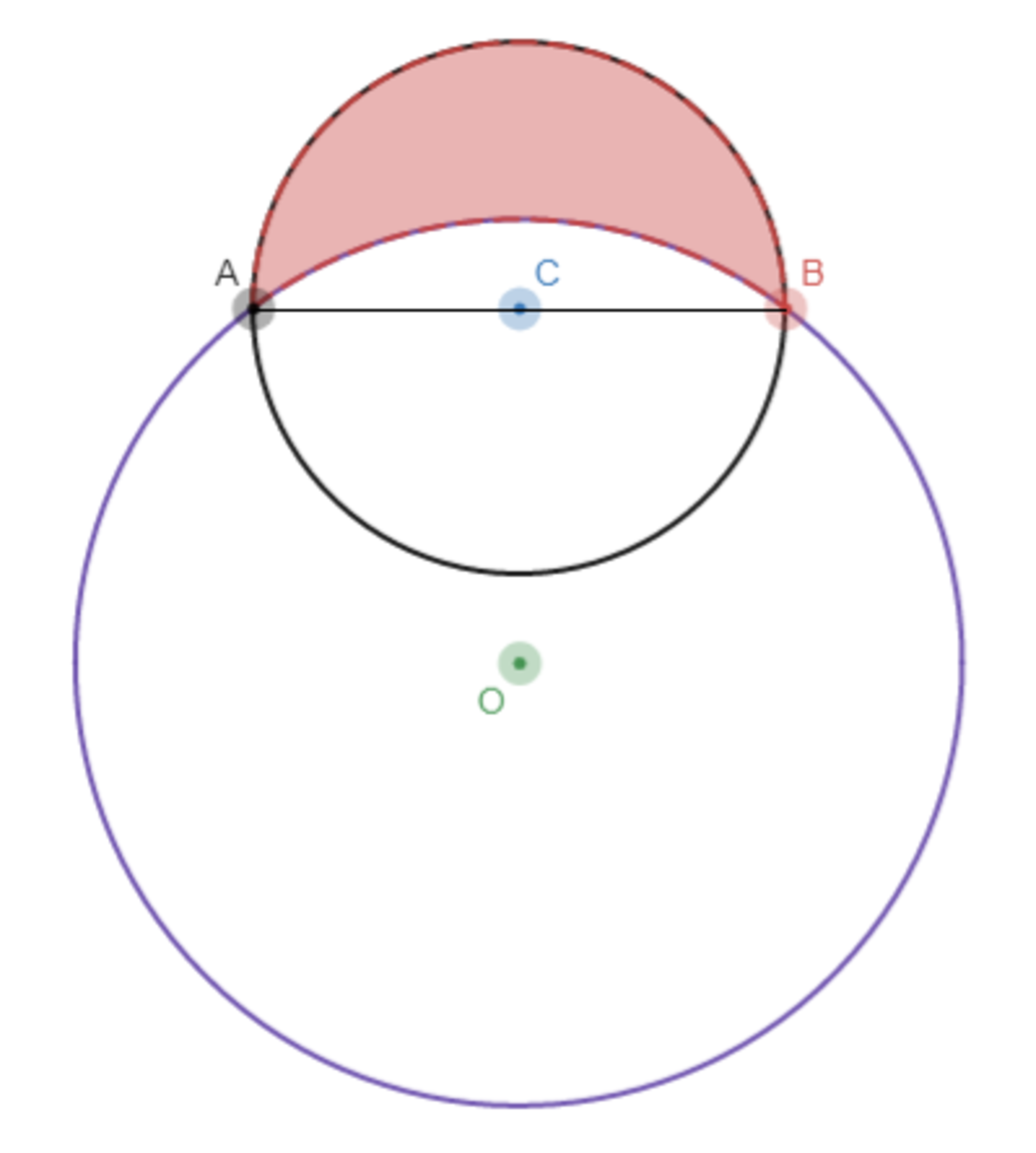
Find the a r e a o f t h e s h a d e d r e g i o n . \red{area\space of \space the \space shaded\space region}.

Bonus : Do it for general circles with radius r 1 , r 2 r_1,r_2 and A B ‾ = d \overline{AB}=d

Inspiration


The answer is 10.049.

Zakir Husain by Zakir Husain , 41, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Saya Suka
Apr 19, 2021

Answer
= Small semicircle area + isosceles triangle area – Big circle's sector
= π(3²)/2 + (1/2)(3+3)√(5²–3²) – π(5²)(2×arcsin{3/5} / 360°)
= 10.049 unit²


1 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...