Make the complex simple

Level 2

Let m m be a line in the complex plane defined by

( 1 − i ) z + ( 1 + i ) z ‾ = 4. (1-i)z+(1+i)\overline{z} =4.

Let z 1 = 2 + 2 i z_1=2+2i be a point in the complex plane.

If the reflection of z 1 z_1 in m m is z 2 z_{2} , then compute the value of

z 1 ‾ ( 1 + i ) + z 2 ( 1 − i ) . \overline{z_{1}}(1+i)+z_{2}(1-i).


The answer is 4.

Sayan Chaudhuri by Sayan Chaudhuri , 23, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Andy Hayes
Sep 8, 2016

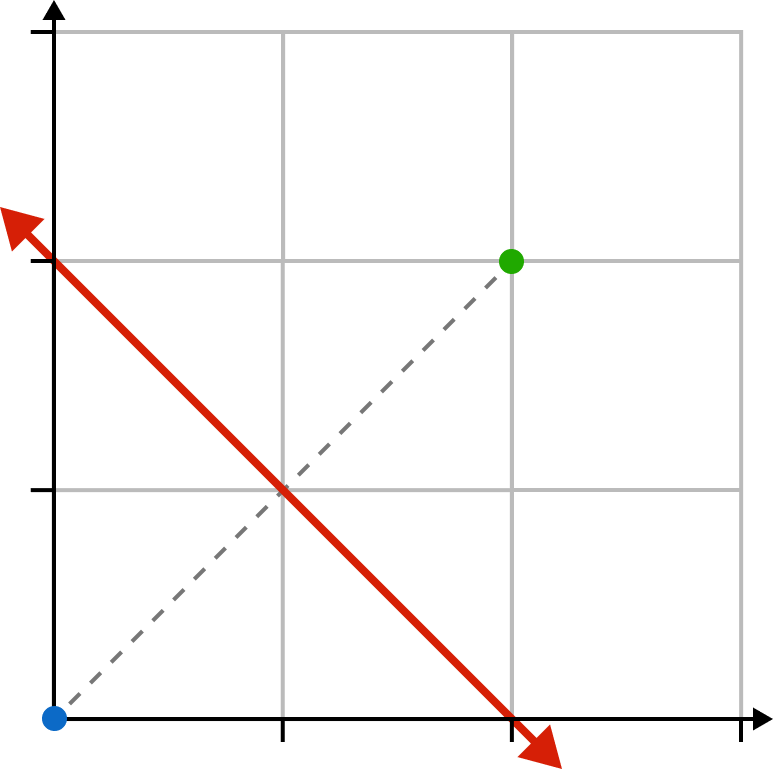
Let z = x + y i z=x+yi . Then the line m m can be written as:

( 1 − i ) ( x + y i ) + ( 1 + i ) ( x − y i ) = 4 (1-i)(x+yi)+(1+i)(x-yi)=4

Solving this equation for y y gives:

y = − x + 2 y=-x+2

By graphing this line and z 1 z_1 , it becomes clear that z 2 = 0 z_2=0 .

Thus, the value of the requested expression is:

( 2 − 2 i ) ( 1 + i ) + 0 ( 1 − i ) = 4 (2-2i)(1+i)+0(1-i)=\boxed{4}

4 Helpful 2 Interesting 0 Brilliant 4 Confused

This is incorrect. We shouldn’t plug in the coordinates of the reflected point in the equation of the line. That point does not lie on the given line. Instead, we must plug in mid-point of (2,2) and (x,y) into the equation of the given line. On solving... We get x+y=0. Comparing distances, we get 2-2i as the other complex number. Answer should be 4(1-i). Please correct me if I am wrong.

Prahlad Verma - 3 years, 3 months ago

Log in to reply

I didn't plug in the coordinates into the equation of the line. I graphed both the line and the point, and by inspection, it can be seen that the reflection of the point about the line is the point ( 0 , 0 ) , (0,0), or simply 0 0 as a complex number.

Andy Hayes - 3 years, 3 months ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...