Map Theory
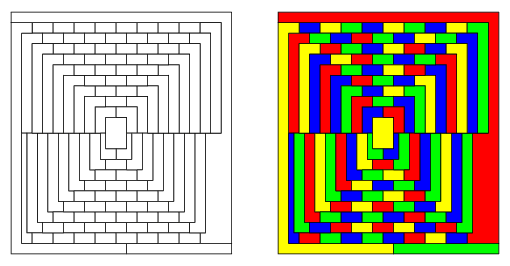What is the least number of different colours necessary to colour a map such that no region shares a border with another region of the same colour?
Think in terms of connected nodes and regional topology. Note that modern proofs are computer-aided, so most likely you'll reason or you've heard of this before.
The answer is 4.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
The map kind of gives it away.
This question is quite amazing, its gone over a century without a proof.