Math in Origami

Without loss of generality, suppose the side of the square has length 4 with A(0, 4), B(4, 4), C(4, 0), D(0, 0). Let E(2, 0) be the midpoint of CD. The fold is the line over which B will be reflected to obtain E. This line is the perpendicular bisector of BE. To construct the perpendicular bisector of BE, we need the midpoint of BE:

$\text{Midpoint of BE: } \left(\frac{2 + 4}{2}, \frac{0 + 4}{2}\right) = (3, 2)$

Furthermore, we need the slope perpendicular to BE. Since the slope of BE is $\frac{4 - 0}{4 - 2} = 2$ , the perpendicular slope is $-\frac{1}{2}.$ Now we can construct the line that forms the crease.

$y - 2 = -\frac{1}{2}(x - 3)$

Our interest is X, the point where this line intersects the side BC. Since BC is concurrent with the line $x = 4,$ we substitute $x = 4$ into the equation of the crease like so:

$y - 2 = -\frac{1}{2}(4 - 3) \longrightarrow y = \frac{3}{2}.$

Because BC has length 4, point X splits BC into two segments CX which has length $\frac{3}{2}$ and BX which has length $\frac{5}{2}.$ The ratio of these lengths is $\frac{3/2}{5/2} = \frac{3}{5} = \boxed{0.6}.$

@Dipannita Guhathakurta, This problem has occurred in the RMO 1990 paper, problem 3, and it is just a one line application of Pythagoras'. 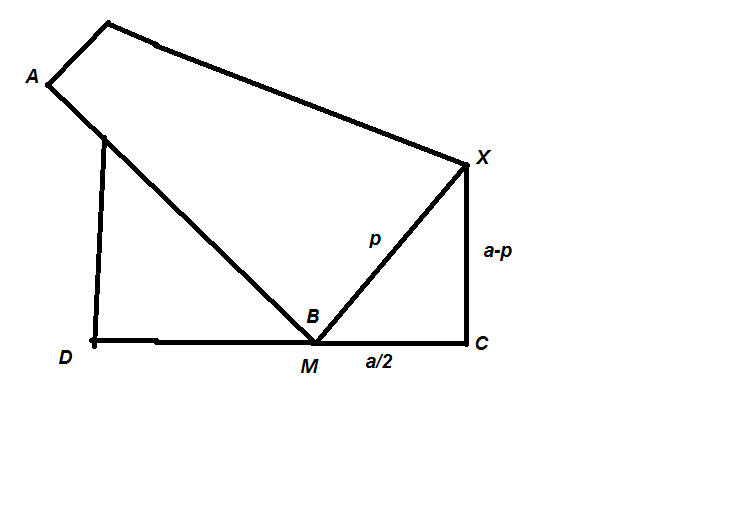In the figure, apply Pythagoras' in triangle BXC, where we have taken the side of the triangle to be a units long, we find that ${p/(a-p)}$ =5/3 , So if we consider the directed segment BC, the answer would be 5/3, and it would be 3/5 if we consider the directed segment to be CB. So I would request the OP writer to edit the problem.

P.S. I think I know you.

P.P.S The geometry problem you have posted is a cult problem, and I know the answer to it. I am not telling it here. If you want to talk about it, feel free to mail at soumavapal@gmail.com, because I think you have given a wrong answer to it. Just verify once by construction with a protractor and a ruler.

it's easy practically....

Let XC=s and XB=t. Midpoint of CD be M. Therefore the hypotenuse MX=t , leg MC= $\dfrac{s+t}2$ and the other leg XC=s.
$\therefore ~ t^2=\left (\dfrac{s+t}2 \right )^2 +s^2 , ~~\implies ~5(\dfrac s t)^2+2 \dfrac s t -3=0.\\ Solving ~quadratic ~equation, ~\dfrac {CX}{XB}=~\dfrac s t=0.6,~ taking ~ ~ only ~ the ~positive ~value.$ .

We will take the midpoint of $\overline{CD}$ to be the point $M$ and take the point where the created fold intersects $\overline{CB}$ to be the point $P$ .

WLOG, take $\overline{AB}=1$ . (Be sure you know why this is retains generality!)

We will consider the general problem of what happens to $\overline{CB}$ when $CM=\alpha$ . In this problem, we are looking to solve the case where $\alpha=\frac{1}{2}$ .

Let the length $CP=x$ .

Knowing $\angle C$ to be right, we apply the Pythagorean Theorem to $\bigtriangleup CMP$ , giving $\alpha^2+x^2=(1-x)^2$ .

Expanding and solving, the quadratic term drops out and we get $x=\frac{1-\alpha^2}{2}$ . (Be sure to see this through for yourself!)

Therefore $\overline{CB}$ is partitioned in a ratio of $\frac{\frac{1-\alpha^2}{2}}{1-\frac{1-\alpha^2}{2}}$ . Rewriting, we have a ratio of $\frac{1-\alpha^2}{1+\alpha^2}$ . (Again, be sure to see this step through!)

In this case, we have $\alpha=\frac{1}{2}$ , so the desired ratio evaluates to $\frac{3}{5}=\boxed{0.6}$ .