Misshapen Brownie
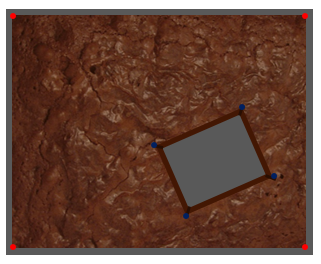 I came back from letting my brownies cool to find that some crazy individual had cut themselves a huge rectangular piece out of the middle of my brownie. The piece does not even align with the tray. Is it still possible to use a single straight cut, that is perpendicular to this surface of the brownie, to cut the remaining brownie area into two pieces of equal area?
I came back from letting my brownies cool to find that some crazy individual had cut themselves a huge rectangular piece out of the middle of my brownie. The piece does not even align with the tray. Is it still possible to use a single straight cut, that is perpendicular to this surface of the brownie, to cut the remaining brownie area into two pieces of equal area?
If not, prove why not. If it's possible, how can you do it?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
If two people want equal shares of the remaining brownie, then the line that goes through the middle of the original brownie rectangle and the middle of the hole rectangle will cut the remaining brownie area in half.
Proof: Any line through the middle of a rectangle cuts that rectangle in half, due to rotational symmetry. Therefore, the line through both the middle of the brownie rectangle and the middle of the hole rectangle will result in each person getting half of the original brownie area and half of the hole area. Therefore, each person will get equal amounts of the misshapen brownie area.