Maximizing Area with a Projectile
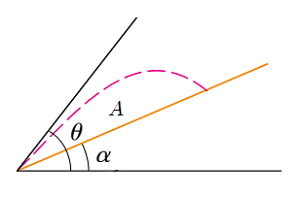
A projectile is fired from the base of a plane that is inclined at an angle , from the horizontal, as shown in the figure, with an initial velocity at an angle of inclination from the horizontal. Determine in degrees such that the area between the trajectory and the inclined plane is maximum.
Details and Assumptions
- .
- Neglect air resistance.
The answer is 75.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
At any time t , the projectile's horizontal and vertical displacement are
x y = v t cos θ , = v t sin θ − 2 1 g t 2 .
If t is eliminated between these two equtions, the following eqution is obtained
y = x tan θ − 2 v 2 cos 2 θ g x 2 .
The projectile hits the slope when
y = x tan α .
Substituting this value into the equation above, we get
x ( tan θ − tan α ) = 2 v 2 cos 2 θ g x 2 .
Solving for x , we have
x = 0 , x = g cos α 2 v 2 sin ( θ − α ) cos θ .
So the area between the trajectory and the inclined plane is
A ( θ ) = ∫ 0 2 v 2 sin ( θ − α ) cos θ / g cos α [ ( x tan θ − 2 v 2 cos 2 θ g x 2 ) − x tan α ] d x = [ 2 1 x 2 ( tan θ − tan α ) − 6 v 2 cos 2 θ g x 3 ] ∣ ∣ ∣ ∣ 0 2 v 2 sin ( θ − α ) cos θ / g cos α = 3 g 2 cos 3 α 2 v 4 sin 3 ( θ − α ) cos θ .
Setting A ′ ( θ ) equal to zero gives
0 ⟹ 0 ⟹ 0 ⟹ 0 = 3 cos ( θ − α ) cos θ − sin ( θ − α ) sin θ = 3 − tan ( θ − α ) tan θ = 3 ( 1 + tan θ tan α ) − ( tan θ − tan α ) tan θ = tan 2 θ − 4 tan α tan θ − 3 .
The physical root of the quadratic equation with respect to tan θ is
tan θ = 2 tan α + 4 tan 2 α + 3 .
It is easy to show that this value of θ maximizes A .
In this case, with tan α = 3 − 1 , we have tan θ = 2 + 3 , and so θ = 7 5 ∘ .