Maximizing Distance

Classical Mechanics Level 2

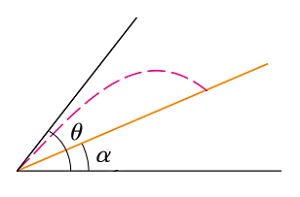
A projectile is fired from the base of a plane that is inclined at an angle α \alpha , from the horizontal, as shown in the figure, with an initial velocity v v at an angle of inclination θ \theta from the horizontal.

What is maximum distance from the projectile to the inclined plane?

Neglect air resistance.

v 2 sin ⁡ 2 ( θ − α ) 2 g cos ⁡ α \frac{v^2\sin^2(\theta-\alpha)}{2g\cos\alpha} v 2 cos ⁡ 2 ( θ − α ) 2 g cos ⁡ α \frac{v^2\cos^2(\theta-\alpha)}{2g\cos\alpha} v 2 sin ⁡ 2 ( θ − α ) 2 g sin ⁡ α \frac{v^2\sin^2(\theta-\alpha)}{2g\sin\alpha} v 2 cos ⁡ 2 ( θ − α ) 2 g sin ⁡ α \frac{v^2\cos^2(\theta-\alpha)}{2g\sin\alpha}

Brian Lie by Brian Lie , 26, China

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Brian Moehring
Aug 4, 2018

The component of the gravitational acceleration orthogonal to the plane is g cos ⁡ α g \cos\alpha , and the component of the initial velocity orthogonal to the plane is v sin ⁡ ( θ − α ) . v\sin(\theta-\alpha).

Then using the equation h = w 2 2 a h = \frac{w^2}{2a} for the maximum height of a projectile with initial vertical velocity of w w and constant downward acceleration a a , we see the maximum distance from the projectile to the inclined plane is ( v sin ⁡ ( θ − α ) ) 2 2 ( g cos ⁡ α ) = v 2 sin ⁡ 2 ( θ − α ) 2 g cos ⁡ α \frac{(v\sin(\theta-\alpha))^2}{2(g\cos\alpha)} = \frac{v^2\sin^2(\theta-\alpha)}{2g\cos\alpha}

4 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...