Maximum number of elements

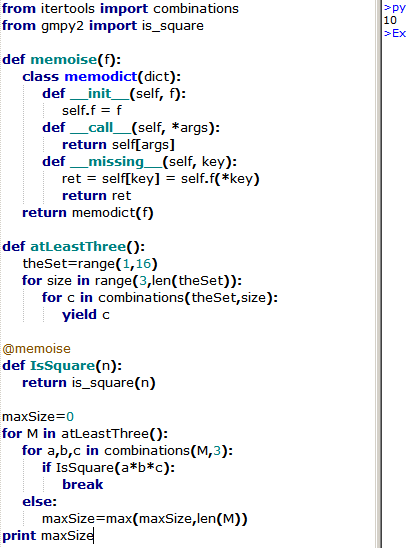
Note that the product of the three elements in each of the sets $\{1,4,9\},\{2,6,12\},\{3,5,15\}$ and $\{7,8,14\}$ is a square.Hence none of these sets is a subset of $M$ . Because they are disjoint, it follows that $M$ has at most $15 - 4 = 11$ elements. Since 10 is not an element of these sets, if $10\notin M$ ,then $M$ have atmost $10$ elements.

Suppose $10 \in M$ .Then none of $\{2, 5\}, \{6, 15\}, \{1, 4, 9\}$ , and $\{7, 8, 14\}$ is a subset of $M$ . If $\{3, 12\} \subset M$ , it follows again that $M$ has at most $10$ elements.

If $\{3, 12\} \subset M$ , then none of $\{1\},\{4\},\{9\},\{2, 6\}, \{5, 15\}$ , and $\{7, 8, 14\}$ is a subset of $M$ , and then $M$ has at most $9$ elements. We conclude that $M$ has at most $10$ elements in any case. Finally, it is easy to verify that the subset $M = \{1, 4, 5, 6, 7, 10, 11, 12, 13, 14\}$ has the desired property. Hence the maximum number of elements in $M$ is $10$ .

Although I did try to write reasonably careful code, I was shocked when this ran in the blink of an eye.