Mechanics |09-06-2021|

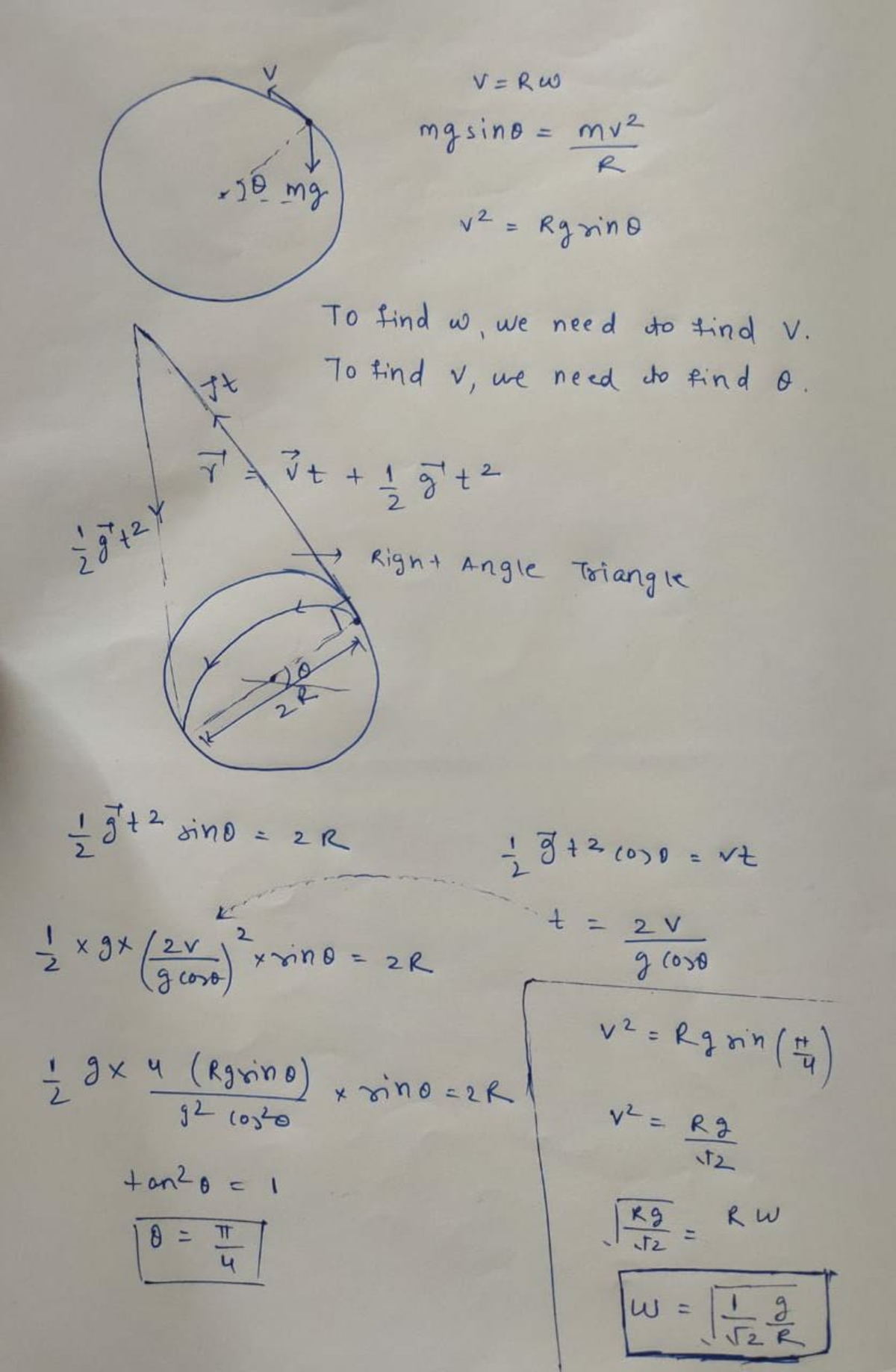
A hollow cylinder is rotating with constant angular velocity about its horizontal axis .A small ball moves in contact with its inner wall ,but leaves contact with it at a position as shown in the figure.

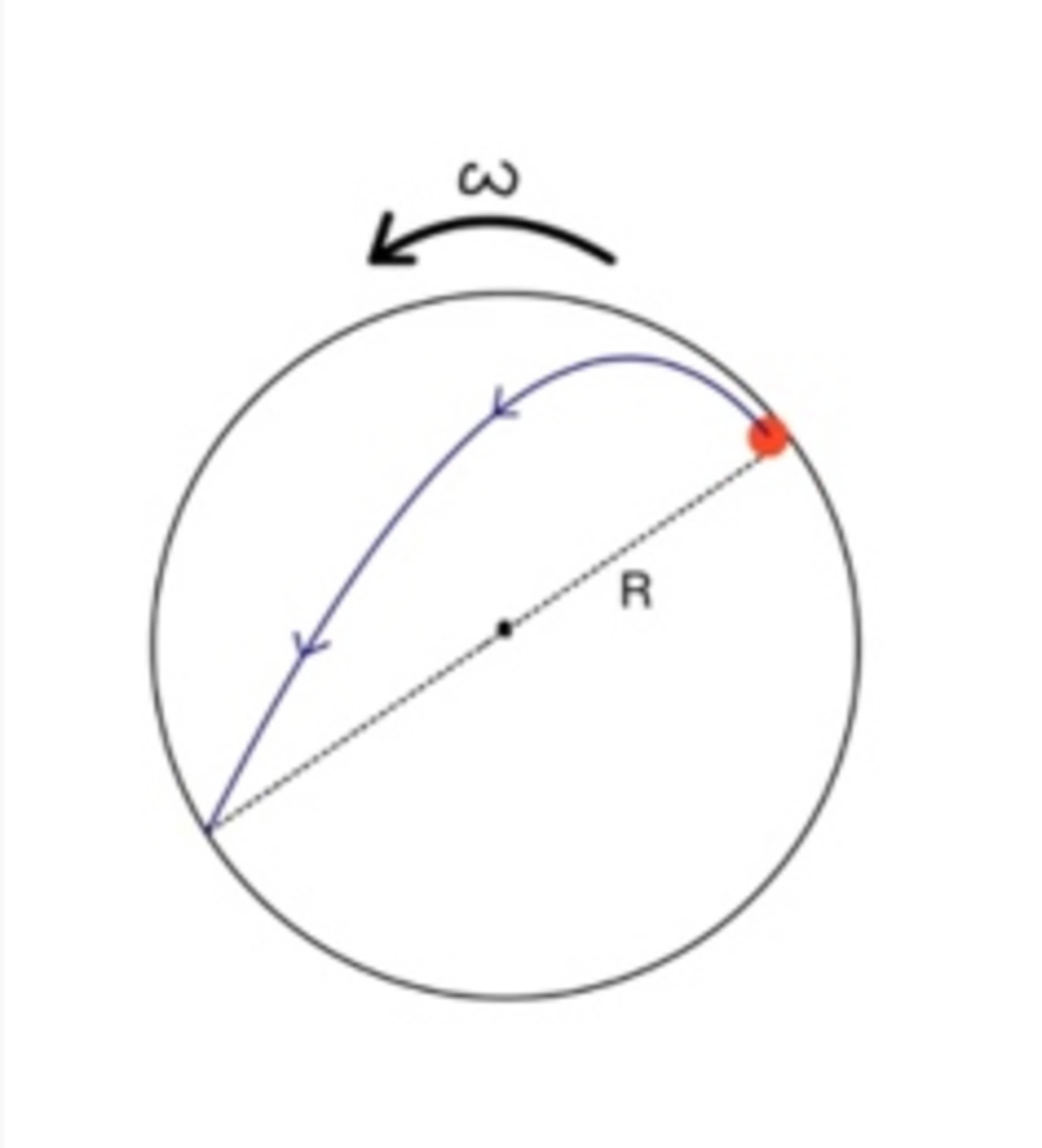
After losing contact,it moves under gravity and hits the diametrically opposite point .
Find the angular velocity of the cylinder .
And it comes in the form of $\omega=\alpha \sqrt{\frac{g}{R}}$
Type $\alpha=?$

Assume friction to be sufficiently high so that it doesn't slide on the cylinder.

Note .
1) $R$ is the radius of cylinder.
2) $g$ is gravitational force acting downwards .
3) While writing the solution also tell where you are migrating from Brilliant website after 2 July 2021.