Minor Arc

Geometry Level pending

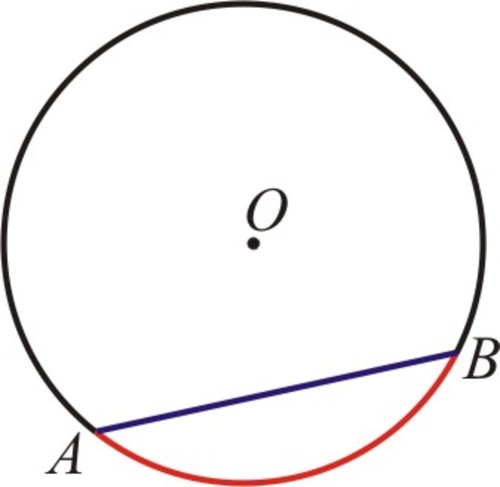In a circle of diameter 40 cm, the length of a chord is 20 cm. Find the length of minor arc of the chord.If the ans. is (x*pi) find x.

22/7 20/3 21/2 23/14

Pratik Raj by Pratik Raj , 22, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

3 solutions

For a given arc the angle subtended at centre is pi/3 as this becomes an equilateral triangle . So the length of arc is given by the product of radius and angle subtended by the arc. Hence it becomes 20 pi /3

2 Helpful 0 Interesting 0 Brilliant 0 Confused
Pradeepa Bk
Jun 20, 2014

The angle A formed by chord at center = 2 arcsin (chord/diameter ) = 2 arcsin(20/40) = 60 degrees.

Arc length S = (A / 180 ) * pi * radius = ( 60/ 180 ) * pi * 20 = (20/3 ) * pi

0 Helpful 0 Interesting 0 Brilliant 0 Confused
Krishna Garg
May 3, 2014

Since diameter of circle is 40 cm ,so radius is 20 cm. Chord length is also 20 cm ,therfore angle at centre for chord is 60 degree. Length of arc = 60/360 X 2 pie X R = 20/3 pia that is X=20/3 Ans

K.K.GARG,India

0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...