Multiple Reflections With Inclined Mirrors

Geometry Level 2

The angle between two mirrors is θ \theta . If the incident ray that is parallel to mirror 1 is reflected 5 times in the setup, and becomes parallel to mirror 1 again. What is the measure (in degrees) of θ \theta ?


The answer is 30.

View wiki
Iit Jee Aspirants by Iit Jee Aspirants , 34, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

5 solutions

Ajit Athle
Jun 6, 2014

Total Deviation = 360 - 5(θ) which in this case =180 + θ or 6θ=180° or θ=30°

6 Helpful 1 Interesting 0 Brilliant 1 Confused

Total deviation is 180+ theta how can it identify

Madhuri Dholakiya - 7 years ago

@Madhuri: I've drawn a diagram which traces the exact path of the ray and explains the solution much better but I do not know how to upload the diagram. If you would write to me at ajitathle@gmail.com, I can forward it to you.

Ajit Athle - 7 years ago

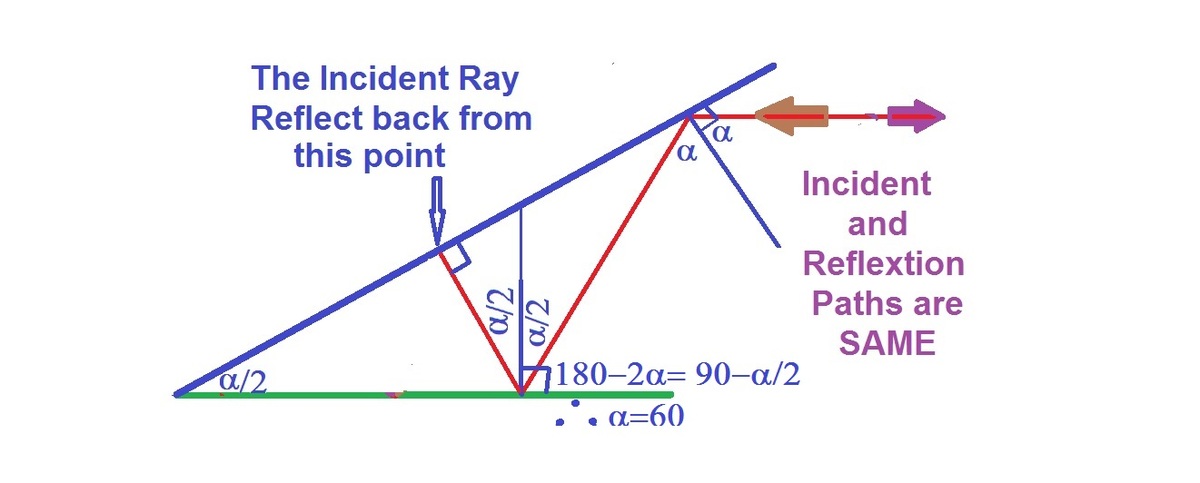
For Incident and Reflection angles to be equal, the condition can only be met for five reflection if there is a symmetry. And that means after third reflation the ray would trance back the same path. That means second reflection on m-2 must be at 90 degrees. If you draw the ray diagram you would see that the other two angles are Alpha/2 and Alpha = 90. Hence Alpha/2 = 30 .

5 Helpful 0 Interesting 0 Brilliant 0 Confused

thus.. there will be no two different parallel rays... the given pic is not realistic.

Ananya Aaniya - 4 years, 9 months ago
Sudipan Mallick
Jun 7, 2014

thita=pi/n+1 (where n is no. of refections) here n=5 so thita = 180/5+1=30.

3 Helpful 0 Interesting 1 Brilliant 0 Confused

Please correct the typo. π n + 1 = 180 5 + 1 = 30. \dfrac\pi {n+1}=\dfrac{180}{5+1}=30.

Niranjan Khanderia - 4 years, 9 months ago

What is this formula about can u elaborate ?

Tarun B - 3 years, 8 months ago
Isaac Browne
Aug 20, 2017

Here is a really nice way of thinking of the reflections.

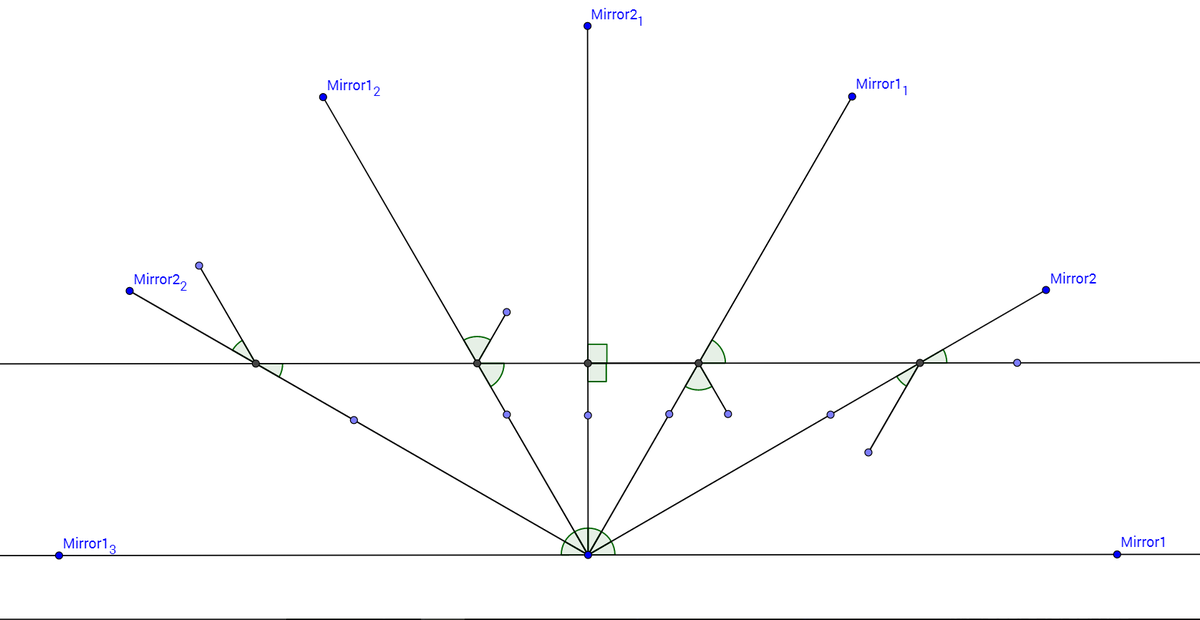
From here it is obvious that θ = 180 / 6 = 3 0 ∘ \theta = 180/6=30^{\circ}

1 Helpful 1 Interesting 0 Brilliant 0 Confused

An explanation I found elsewhere:

https://socratic.org/questions/two-plane-mirrors-are-included-at-an-angle-theta-as-shown-in-the-figure-light-ra

0 Helpful 0 Interesting 0 Brilliant 0 Confused

1 pending report

Vote up reports you agree with

×

Problem Loading...

Note Loading...

Set Loading...