Displacement Isn't Distance!

Classical Mechanics Level 2

A particle is thrown straight up with a velocity of $20 \text{ m/sec}$ . Find the time (in seconds) from the start of motion at which the distance traveled is twice the displacement.

Use $g=10~m/s^{2}$ .

3

2 + \sqrt{ \frac 4 3}

1

None of the given choices

2 + \sqrt{ \frac 3 4}

3 solutions

Nihar Mahajan
Apr 2, 2015

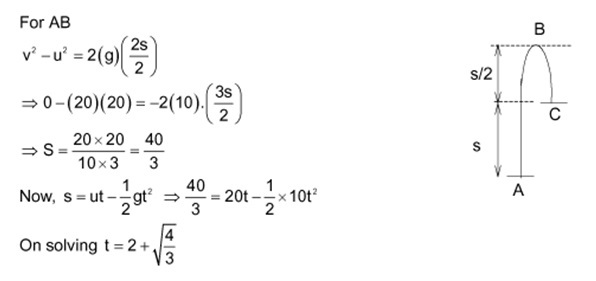
Using $h=\dfrac{u^2}{2g}$ , we easily calculate $h=20m$

According to given condition , assuming that the object at that instant of time has travelled $(x+20)$ distance and has displacement $(20-x)$ ,

$20+x=2(20-x) \Rightarrow x=\dfrac{20}{3}$

Using $s=vt_2+\dfrac{1}{2}at_2^2$ ,

$\Rightarrow \dfrac{20}{3}=(0)(t_2)+\dfrac{1}{2}(10)t_2^2 \Rightarrow t_2 = \sqrt{\dfrac{4}{3}}$

When the object reaches max. height ,

$t_1=\dfrac{u}{g} = \dfrac{20}{10} \Rightarrow t_1=2$ sec

Hence , total time $= t_1+t_2 = \huge\boxed{\color{#3D99F6}{2+\sqrt{\dfrac{4}{3}}}}$

Good job kid but the former is shorter and quicker

Kyle Finch - 6 years, 2 months ago

thank you!

Azalea Satya - 5 years, 1 month ago

Tanishq Varshney
Apr 2, 2015

Nice. Good approach.

Niranjan Khanderia - 6 years, 2 months ago

Sir, kindly mention in your problem that g=10 meter per (second)^2. I had calculated g as 9.8 and got my answer 3.2191 sec which is none of these. Your answer is 3.1547 sec. So, we are facing trouble for not mentioning the "g" as 10. Please Edit the problem.

Rubayet Tusher - 6 years, 1 month ago

I know its quite late, i am replying to u, plz don't call me sir , ironically u r elder than me , just call me Tanishq, $\ddot \smile$

Tanishq Varshney - 6 years ago

Ok, Tanishq. I will do so from now on. Thanks for correcting the problem.

Rubayet Tusher - 6 years ago

Brilliant.. though i am an economics student, i liked d way u approached the problem

Nithin Nithu - 6 years, 2 months ago

Abu Zubair
Apr 4, 2015

Gravity is taken as 10m/s^2

This should be mentioned.

People tend to take g as 9.8m/s^2

You should have filed this as a report. I have reported this error for you. :)

Samuel Li - 6 years, 2 months ago

Technically this is wrong. On the way up the distance traveled equals the displacement. On the way down, he has traveled more distance than and the displacement vector is opposite direction and less. The real answer is at t=4. You're asking a mathematical question that is physically impossible until t=4.

A Former Brilliant Member - 1 year, 3 months ago

Displacement Isn't Distance!

3 solutions

0 pending reports