My name is equation................X Y equation... got it......

Algebra Level 3

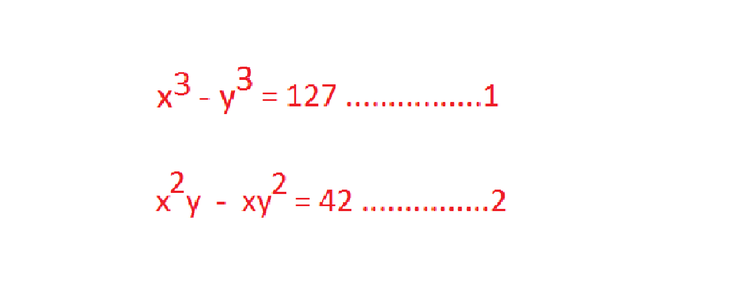find the sum of all the integral solutions of x and y.

2 solutions

Ashutosh Mahapatra
Jun 5, 2014

Check this out $x^3-y^3=127$

$=>x-y=1..................1$

now considering 2nd equation,

$xy[x-y]=42$

$=>xy=42.............2$

Now using 1st equation it is found that the integral solutions are

$x=7,-6 , y=6,-7$

so their sum is $\boxed{0}$

You must mention that 127 is prime

Jayakumar Krishnan - 7 years ago

Moshiur Mission
Apr 3, 2014

(x-y)^3 = 127-3*42 so x-y = 1 or x = 1+y taking integral x^2/2 = y^2/2+y implies x = y =0

Why are you taking integrals? The condition only holds at a set of points, and not necessarily over the entire line / region.

Note that $x = 7, y = 6$ and $x=-6, y=-7$ are the only solutions, which is why they sum to 0.

Calvin Lin Staff - 7 years, 1 month ago

After getting x-y =1 , how did you get the roots?

aditya chandratre - 7 years ago