My school teacher didn't teach me this
Which of the following statements is/are true?
The set of all real numbers in the interval is not countable.
The set of all rational numbers in the interval is not countable.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
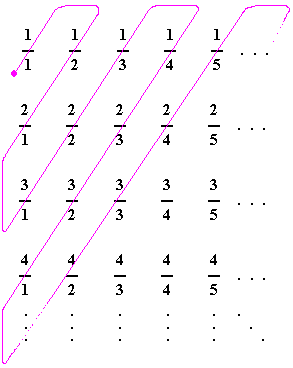
Two of the fundamental concepts of Mathematical Analysis are:
Any interval in R is uncountable.
The set of all rational numbers is a countable set.
However the proof of the hypothesis were not easy though. So finally it was Georg Cantor who published the proofs in 1874 in his papers on infinite sets using Nested Interval Property in [ 0 , 1 ] . Later in 1891, he provided a more elegant proof in form of Diagonalisation Argument. He paints an artistic picture of reals .To me the proof is so simple and aesthetic that it shows why mathematics is one of the Arts. It is the artistic sense of his proof that demands a complete wiki article on it.