Nagel Point Meets Incircle
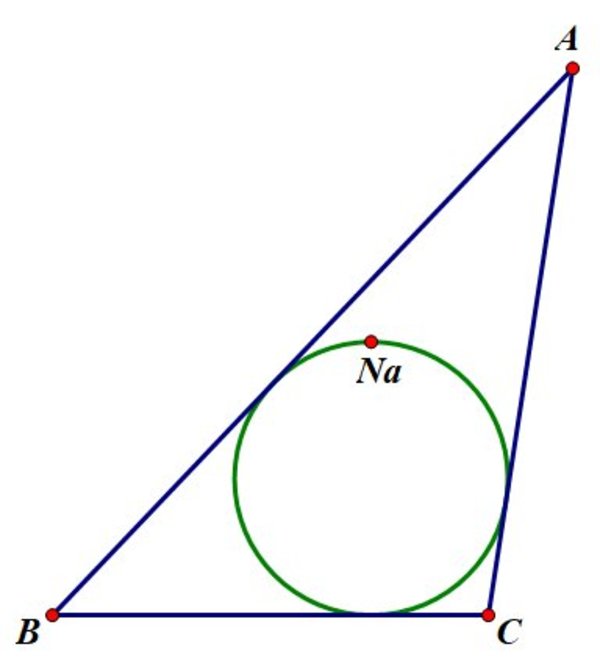 The triangle
A
B
C
is such that its
Nagel point
N
a
lies on its incircle. The triangle has perimeter
2
5
6
. What is the length of its shortest side?
The triangle
A
B
C
is such that its
Nagel point
N
a
lies on its incircle. The triangle has perimeter
2
5
6
. What is the length of its shortest side?
The answer is 64.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Let a = B C , b = A C , and c = A B , and let the coordinates of the vertices be B ( − 2 1 a , 0 ) , C ( 2 1 a , 0 ) , and A ( p , q ) .
The centroid G has coordinates G ( 3 p − 2 1 a + 2 1 a , 3 q + 0 + 0 ) = G ( 3 p , 3 q ) .
The incenter I has coordinates I ( a + b + c a p + 2 1 a b + 2 1 a c , a + b + c a q + 0 + 0 ) = I ( 2 ( a + b + c ) 2 a p + a b + a c , a + b + c a q ) .
Since B C lies along the x -axis, the radius r of the incenter is the same as the y -coordinate of the incenter, so r = a + b + c a q .
The centroid, incenter, and Nagel point all lie on the Nagel line such that 3 G I = r , so:
3 ( 2 ( a + b + c ) 2 a p + a b + a c − 3 p ) 2 + ( a + b + c a q − 3 q ) 2 = a + b + c a q
By the distance formula on A B , c 2 = ( p + 2 1 a ) 2 + q 2 and by the distance formula on A C , b 2 = ( p − 2 1 a ) 2 + q 2 . Solving these two equations for p and q 2 gives p = 2 a c 2 − b 2 and q 2 = 4 a 2 2 a 2 b 2 + 2 a 2 c 2 + 2 b 2 c 2 − a 4 − b 4 − c 4 , and substituting these into the equation above and simplifying we obtain:
( 3 a − b − c ) ( 3 b − a − c ) ( 3 c − a − b ) = 0
Without loss of generality, let 3 a − b − c = 0 , which means b + c = 3 a and c = 3 a − b .
By the triangle inequality theorem , c < a + b , and substituting c = 3 a − b gives 3 a − b < a + b , which solves to a < b . By a similar argument, a < c , so a is the shortest side.
Substituting b + c = 3 a into the the perimeter P = a + b + c and solving gives a = 4 1 P .
Therefore, for a triangle with a perimeter of 2 5 6 and a Nagel point lying on the incenter, the shortest side is a = 4 1 P = 4 1 ⋅ 2 5 6 = 6 4 .
The
Nagel Point
of a triangle is the point of concurrency of its Nagel cevians, which in-turn are the segments joining a triangle's vertex to the point of contact of the excircle corresponding to that vertex with the side opposite to the same vertex.
Property: Let
A
A
1
be the nagel cevian corresponding to vertex
A
and
D
′
be the point diametrically opposite to
D
on
⊙
I
, then
D
′
∈
A
A
1
.
And cyclically for rest three cases. Here, ATQ,
D
′
≡
N
a
.
Using the property on the nagel cevian corresponding to vertex C , one gets ∠ N a F C = 9 0 ∘ and some angle chase would conclude that ∠ D N a C = ∠ 2 B or ∠ B C N a = 9 0 ∘ − ∠ 2 B .
It's easily seen now that, Δ B I A C ≅ Δ C N a B , which implies that Exradius I A A 1 = N a D = 2 × inradius. ∴ A G = 2 × A F ⇒ 2 s = 2 × A F ⇒ A F = 4 s = 6 4 . It's apparent now that B C = 6 4 . That it indeed is the shortest side is also trivially seen.
Just For the sake of synthetic spirit:)
The Nagel point of the triangle has barycentric coordinates s − a : s − b : s − c , while the incentre has barycentric coordinates a : b : c . The distance between two points P , Q with normalized barycentric coordinates p 1 : p 2 : p 3 and q 1 : q 2 : q 3 (so that p 1 + p 2 + p 3 = q 1 + q 2 + q 3 = 1 ) is given by the formula P Q 2 = − a 2 ( p 2 − q 2 ) ( p 3 − q 3 ) − b 2 ( p 1 − q 1 ) ( p 3 − q 3 ) − c 2 ( p 1 − q 1 ) ( p 2 − q 2 )
Thus the distance between the Nagel point and the incentre is given by N a I 2 = − a + b + c a 3 + b 3 + c 3 − 2 ( a 2 b + a b 2 + a 2 c + a c 2 + b 2 c + b c 2 ) + 9 a b c On the other hand the inradius r of A B C has formula r 2 = s ( s − a ) ( s − b ) ( s − c ) = 4 ( a + b + c ) ( b + c − a ) ( a + c − b ) ( a + b − c ) and hence we deduce that N a I 2 − r 2 = 4 ( a + b + c ) ( a + b − 3 c ) ( a + c − 3 b ) ( b + c − 3 a ) Since N a lies on the incircle we know that N a I = r , and hence ( a + b − 3 c ) ( a + c − 3 b ) ( b + c − 3 a ) = 0 Without loss of generality we deduce that b + c = 3 a . Thus we can write b = 2 3 a − d , c = 2 3 a + d for some 0 < d < 2 3 a . It is clear that c is then the largest of the three sides of the triangle. Since a , b , c form the sides of the triangle, we must have 2 3 a + d = c < a + b = a + 2 3 a − d , so that d < 2 1 a . Thus the triangle A B C has sides a < b = 2 3 a − d < c = 2 3 a + d for any 0 < d < 2 1 a . Thus the perimeter of the triangle A B C is 4 a = 2 5 6 , so we deduce that the length of its shortest side is a = 6 4 .