New earth, How does it look like?
 A meteor strikes the earth,well ... as a natural consequence, humanity has vanished as any other kind of life ,the meteor was as big as mercury, after 800 years the new earth started to cool down and take another spherical shape, well not exactly as a perfect sphere. It's shaped as an oblate spheroid.
A meteor strikes the earth,well ... as a natural consequence, humanity has vanished as any other kind of life ,the meteor was as big as mercury, after 800 years the new earth started to cool down and take another spherical shape, well not exactly as a perfect sphere. It's shaped as an oblate spheroid.
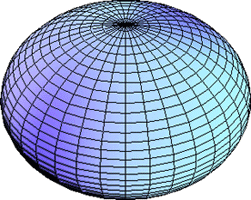
What is the pole to pole diameter of the new earth?
Details And Assumptions :
-The meteor has increased the mass of Earth.
-The meteor-Earth mixed new planet is homogeneous.
-The angular velocity of the new Earth is .
-The new equatorial radius is 7530 km.
-New mass of the earth is .
Hint: What is the condition so masses aren't displaced to another location on the New Earth?
express your answer in Kilometer with three decimal places.
The answer is 15043.03.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
The condition is when all tiny masses on Earth surface are equal in potential, So first I will calculate the gravitational potential then I will calculate the angular rotating potential.
first
U m = ∫ R ∞ R 2 G M E m d r = − R E G M E m
second the Extra potential caused by rotation:
to calculate this we should use "Angular momentum conservation" so the masses when they come from infinity (the angular velocity is zero) to distance R from the center of earth they should gain angular velocity :
L = I ω = c o n s t a n t
L = m r 2 ω → ω m = ω f r 2 ( R s i n θ ) 2
|| spherical coordinates
d U e x t r a = ( ω m ) 2 x d x = ( ω f ) 2 ( x R s i n θ ) 4 x d x
U e x t r a = m ∫ x 0 ∞ ( ω f ) 2 ( x R s i n θ ) 4 x d x
U e x t r a = − m 2 1 ( ω f ) 2 ( R s i n θ ) 2
U = U m + U e x t r a = c o n s t for all the earth surface so
the potential when θ = π / 2 is − R E G M E m − m 2 1 ( ω f ) 2 ( R s i n θ ) 2
this is equal to that one in the pole when θ = 0 so
− R G M E m − m 2 1 ( ω f ) 2 ( R ) 2 = − R p G M E m
we calculate R p = 7 5 2 1 . 5 1 5 k m