New Five Incircles Revolution!

Just for drill, I wanted to see if sympy could solve this.

 1
 2
 3
 4
 5
 6
 7
 8
 9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36

# Find five congruent incircles in the unit square
# See https://www.geogebra.org/classic/eknnqbe2

from sympy import *
from sympy.abc import x

# define the unit square, ABCD
A = Point(0, 0)
B = Point(1, 0)
C = Point(1, 1)
D = Point(0, 1)

E = Point(x, 0)         # let x = AE
F = Point(1, x)         # so x = BF by symmetry
G = Point(1-x, 1)       # so x = GC by symmetry
H = Point(0, 1-x)       # so x = DH by symmetry
M = Point(1/2, 1/2)     # M = centroid ABCD

r1 = Triangle(A, B, F).inradius
r2 = Triangle(C, G, B).inradius
r3 = Triangle(D, H, C).inradius
r4 = Triangle(A, E, D).inradius
r5 = Line(D, E).distance(M)

sol = solve(r1 - r5, x)
for i in sol:
    a = r1.subs(x, i)
    b = r2.subs(x, i)
    c = r3.subs(x, i)
    d = r4.subs(x, i)
    e = r5.subs(x, i)
    print("x = ", simplify(i))
    print("Radius =", N(a))
    print("Checking equality of five radii... ", end=' ')
    print(N(a) == N(b) == N(c) == N(d) == N(e))
    print("Solution: ", floor(N(a) * 10**5))

1
2
3
4

x =  -7/(4*(13 + 16*sqrt(2))**(1/3)) + 1/4 + (13 + 16*sqrt(2))**(1/3)/4
Radius = 0.201964181008339
Checking equality of five radii...  True
Solution:  20196

Label the diagram as follows, and let $AF = ED = k$ :

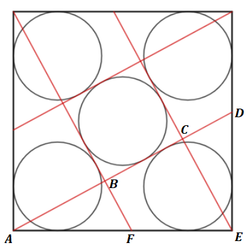
By the Pythagorean Theorem on $\triangle ADE$ , $AD = \sqrt{DE^2 + AE^2} = \sqrt{k^2 + 1}$ .

Since $\triangle ABF \sim \triangle AED$ by AA similarity, $AB = AF \cdot \cfrac{AE}{AD} = k \cdot \cfrac{1}{\sqrt{k^2 + 1}} = \cfrac{k}{\sqrt{k^2 + 1}}$ and $BF = AF \cdot \cfrac{DE}{AD} = k \cdot \cfrac{k}{\sqrt{k^2 + 1}} = \cfrac{k^2}{\sqrt{k^2 + 1}} = CD$ .

Then $BC = AD - AB - CD = \sqrt{k^2 + 1} - \cfrac{k}{\sqrt{k^2 + 1}} - \cfrac{k^2}{\sqrt{k^2 + 1}} = \cfrac{1 - k}{\sqrt{k^2 + 1}}$ .

As an incircle of the red square, $r = \cfrac{1}{2} BC = \cfrac{1}{2} \cdot \cfrac{1 - k}{\sqrt{k^2 + 1}} = \cfrac{1 - k}{2\sqrt{k^2 + 1}}$ .

As an incircle of right $\triangle ADE$ , $r = \cfrac{1}{2}(DE + AE - AD) = \cfrac{1}{2}(k + 1 - \sqrt{k^2 + 1})$ .

So $r = \cfrac{1 - k}{2\sqrt{k^2 + 1}} = \cfrac{1}{2}(k + 1 - \sqrt{k^2 + 1})$ , which solves numerically to $r \approx 0.201964$ and $k \approx 0.540790$ .

Therefore, $\lfloor 10^5 r \rfloor = \boxed{20196}$ .

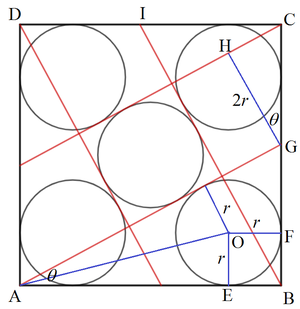
Label the unit square $ABCD$ , red lines $AG$ and $BI$ , the center of the bottom-right circle $O$ . Let $OE$ and $OF$ be perpendicular to $AB$ and $BC$ respectively, and $\angle GAB=\theta$ . We note that

$\begin{aligned} AE + EB & = AB \\ OE \cdot \cot \frac {\angle GAB}2 + OF & = AB \\ r \cot \theta 2 + r & = 1 & \small \blue{\text{Let }t = \tan \frac \theta 2} \\ \frac rt + r & = 1 \\ \implies r & = \frac 1{\frac 1t + 1} = \frac t{1+t} \end{aligned}$

Due to symmetry, the two pairs of parallel red lines are perpendicular to each other. That is $AG || BI$ . Let $GH || BI$ . Then $\angle HGC = \theta$ and:

$\begin{aligned} GH & = 2r \\ CG \cdot \cos \theta & = 2r \\ (BC - BG) \cos \theta & = 2r \\ (1-\tan \theta) \cos \theta & = 2r \\ \cos \theta - \sin \theta & = 2r \\ \frac {1-2t-t^2}{1+t^2} & = \frac {2t}{1+t} \\ 3t^3+3t^2+3t-1 & = 0 & \small \blue{\text{Solving for }t \text{ by numerical method}} \\ \implies t & \approx 0.253076587 \\ r & = \frac t{1+t} \approx 0.201964181 \\ \implies \lfloor 10^5r \rfloor & = \boxed{20196} \end{aligned}$