Newton Raphson Works Great, Until It Doesn't - Part 2
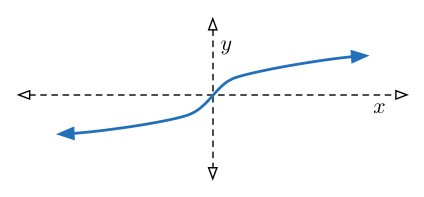 Consider the real valued function given by
f
(
x
)
=
3
x
. If we apply the
Newton Raphson method
with the approximate root
x
0
=
0
.
2
, which root would we converge to?
Consider the real valued function given by
f
(
x
)
=
3
x
. If we apply the
Newton Raphson method
with the approximate root
x
0
=
0
.
2
, which root would we converge to?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Note that as x goes from 0 to ± ∞ , the derivative of 3 x approaches 0 monotonically. Therefore, after doing the Newton Raphson method one time from an arbitrary approximate root x 0 , we end up with the approximate root x 1 such that sgn ( x 1 ) = − sgn ( x 0 ) and ∣ x 1 ∣ > ∣ x 0 ∣ . This repeats to infinity, having x n become larger and larger while switching signs. Therefore, the method will not converge.
This solution is all just my intuition, not really any rigorous math, so apologies for any confusion.
Note that x n =0.2(-2)^{n}. Then the process not converge