OCR A Level: Further Pure 2 - Rational Graphs [June 2012 Q8]
The curve has equation , where and are polynomials of degree 2 and 1 respectively. The asymptotes of the curve are and , and the curve passes through the point .
Express the equation of in the form .
For the curve , find the range of values that can take.
Another curve, , has equation , where and are the polynoimals found in part .
It is given that intersects the line exactly once. Find the coordinates of the point of intersection.
If the coordinates of the point of intersection are , input as your answer.
There are 4 marks available for part (i), 4 marks for part (ii) and 4 marks for part (iii).
In total, this question is worth 16.7% of all available marks in the paper.
This is part of the set OCR A Level Problems .
The answer is 4.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
The mark scheme for this question: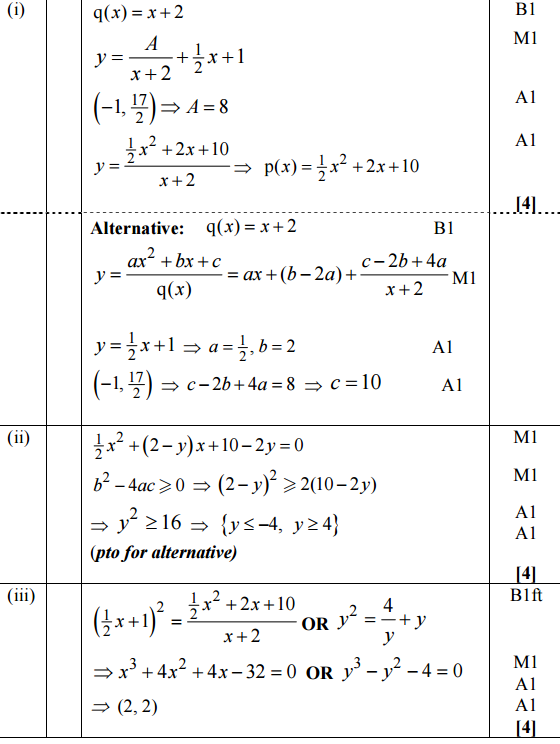 Large Version (Page 1)
Large Version (Page 1)
Large Version (Page 2)