OCR A Level: Mechanics 2 - Centre Of Mass [June 2006 Q3]
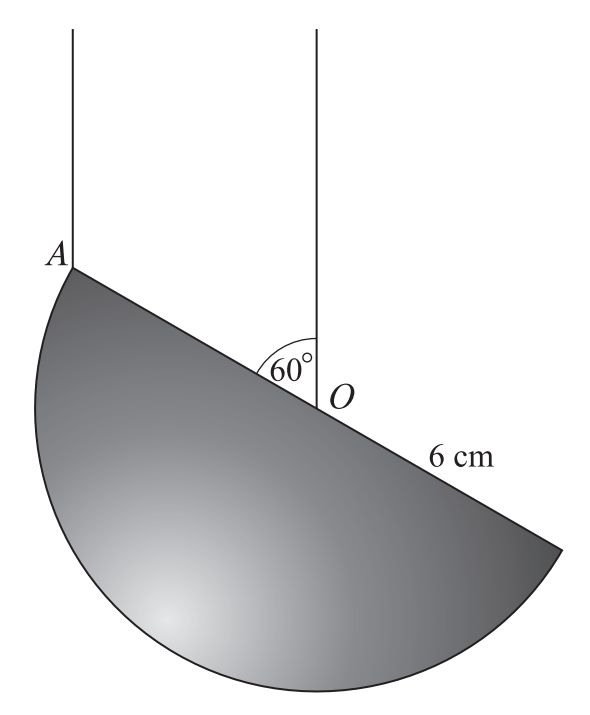 A uniform solid hemisphere of weight
and radius
is suspended by two vertical strings. One string is attached to the point
, the centre of the plane face, and the other string is attached to the point
on the rim of the plane face. The hemisphere hangs in equilibrium and
makes an angle of
with the vertical (see diagram).
A uniform solid hemisphere of weight
and radius
is suspended by two vertical strings. One string is attached to the point
, the centre of the plane face, and the other string is attached to the point
on the rim of the plane face. The hemisphere hangs in equilibrium and
makes an angle of
with the vertical (see diagram).
Find the horizontal distance from the centre of mass of the hemisphere to the vertical through .
Calculate the tensions in the strings (to three significant figures).
Input the product of the two tensions as your answer.
There are 2 marks available for part (i) and 5 marks for part (ii).
In total, this question is worth 9.72% of all available marks in the paper.
This is part of the set OCR A Level Problems .
The answer is 24.44.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
The mark scheme for this question: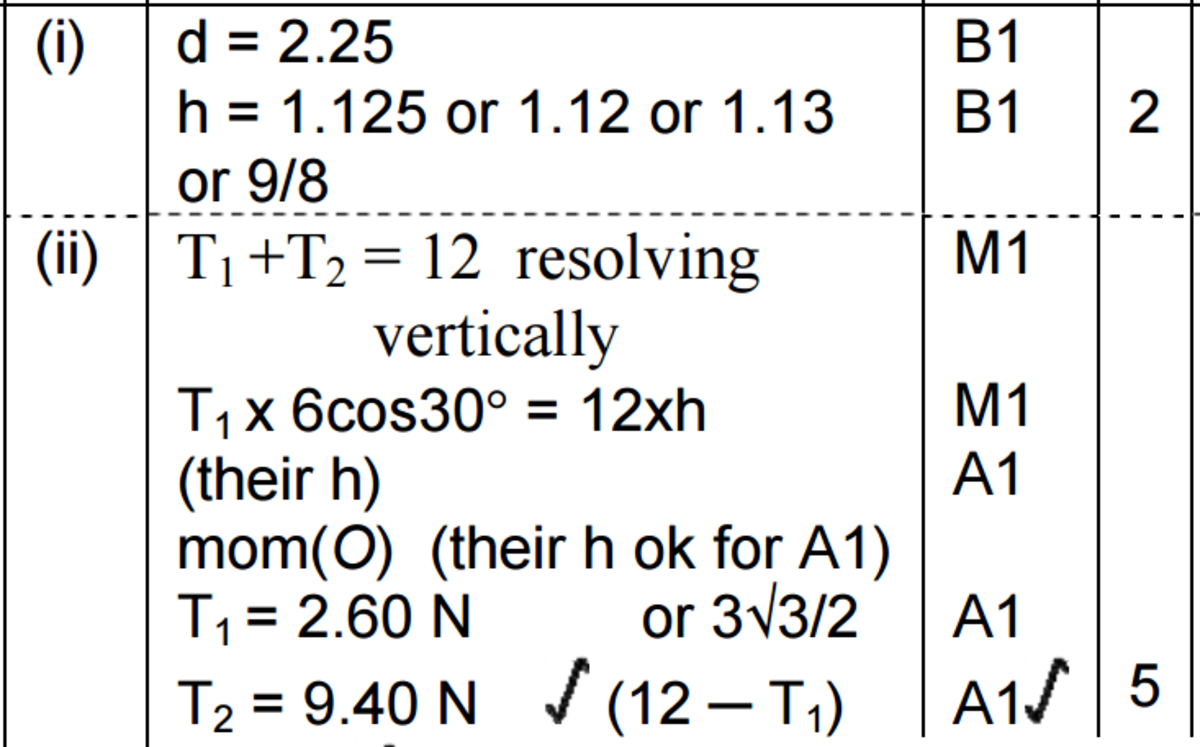 Full Version
Full Version