OCR A Level: Mechanics 2 - Circular Motion [June 2010 Q5]

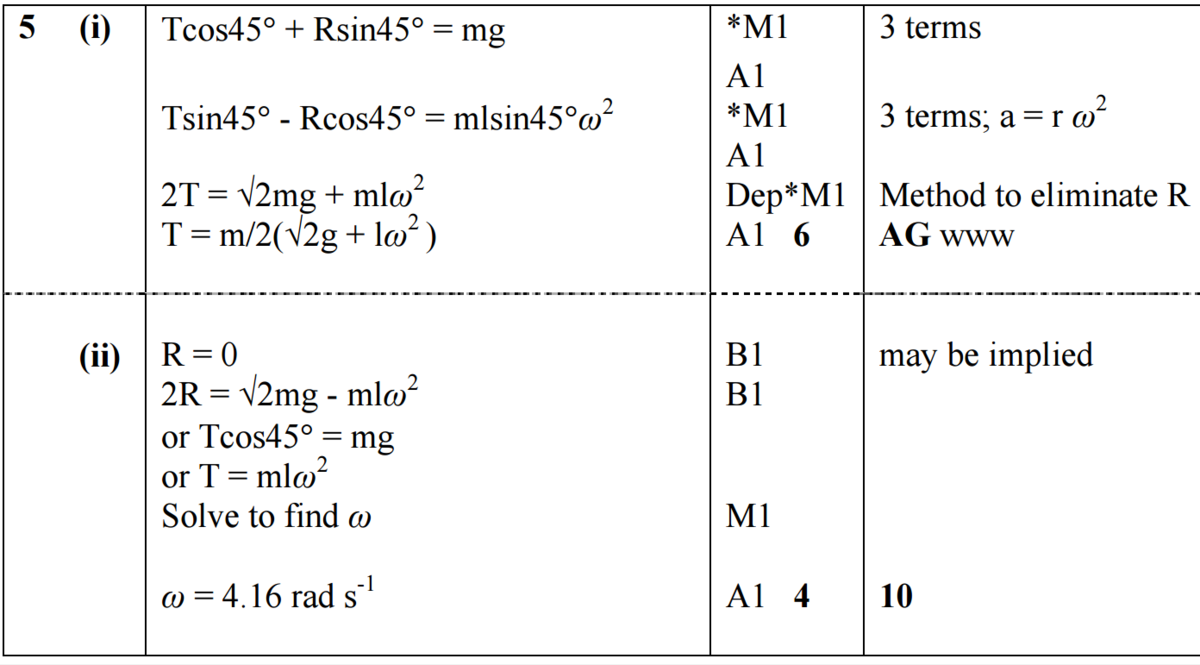
Resolving the forces into horizontal and vertical components:

$||T| \sin \ \frac{\pi}{4} \ - \ |R| \sin \ \frac{\pi}{4} \ = \ \frac{mv^2}{R} \ = \ \frac{mv^2}{l \ \sin \ \frac{\pi}{4}}$ $|T| \sin \ \frac{\pi}{4} \ + \ |R| \sin \ \frac{\pi}{4} \ = \ mg$

Adding the two equations together:

$2|T| \sin \ \frac{pi}{4} \ = \ \frac{mv^2}{l \ \sin \ \frac{\pi}{4}} \ + \ mg \ = \ m(l \ \sin \ \frac{\pi}{4})\omega^2 \ + \ mg \ \Rightarrow \ |T| \ = \ \frac{1}{2} m \ \Big( l \omega^2 \ + \ \frac{g}{\sin \ \frac{\pi}{4}} \Big) \ = \ \frac{1}{2} m \ \Big( l \omega^2 \ + \ \sqrt{2} g \Big)$

Now, to find maximum $omega$ for which the ball stays in contact with the surface, we set $R \ = \ 0$ and use one of the expressions for tension:

$|T| \sin \ \frac{\pi}{4} \ + \ |R| \sin \ \frac{\pi}{4} \ = \ mg \ \Rightarrow \ \Big( \sin \ \frac{\pi}{4} \Big) \ \frac{1}{2} m \ \Big( l \omega^2 \ + \ \sqrt{2} g \Big) \ = \ mg \ \Rightarrow \ \frac{1}{2} \ \Big( \frac{l \omega^2}{\sqrt{2}} \ + \ g \Big) \ = \ g \ \Rightarrow \ \frac{l \omega^2}{\sqrt{2}} \ = \ g \ \Rightarrow \ \omega_{max} \ = \ \sqrt{\frac{\sqrt{2} g}{l}}$

$\omega_{max} \ = \ \sqrt{\frac{\sqrt{2} g}{l}} \ = \ \sqrt{\frac{\sqrt{2} (9.81)}{0.8}} \ = \ 4.16 \ \text{rad}/\text{s}$

The mark scheme for this question: Large Version