Octagon inside a octagon

Let the regular octagon be of unit side length. $FEJG$ is a rhombus, thus, $GJ=FE=1$ .

Consequently, $HGJ$ is an isosceles right triangle, hence, by Pythagorean theorem,

$HJ=\sqrt{H{{G}^{2}}+G{{J}^{2}}}=\sqrt{{{1}^{2}}+{{1}^{2}}}=\sqrt{2}$ $GFKH$ is also a rhombus, thus, $HK=GF=1$ .

Hence, the ratio in question is $\frac{KJ}{GF}=\frac{HJ-HK}{GF}=\frac{\sqrt{2}-1}{1}=\sqrt{2}-1$ For the answer, $a=2$ , $b=1$ , thus, $a+b=\boxed{3}$ .

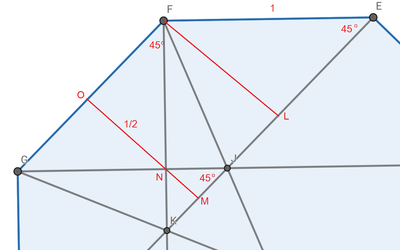
Let the side length of the regular octagon be $1$ , $GJ$ and $FK$ intersect at $N$ , $FL$ and $OM$ , which passes through $N$ , be perpendicular to $HE$ . Note that $\triangle EFL$ is an isosceles right triangle. Hence $FL = OM = \frac 1{\sqrt 2}$ . $\triangle FNO$ is also an isosceles right triangle. This means that $OF = ON = \frac 12$ . $\triangle FGN$ and $\triangle KJN$ are also isosceles right triangles and are hence similar. Then

$\frac {KJ}{GF} = \frac {MN}{ON} = \frac {OM-ON}{ON} = \frac {\frac 1{\sqrt 2}-\frac 12}{\frac 12} = \sqrt 2 - 1$

Therefore $a+b = 2+1 = \boxed 3$ .