Olympiad specials

Geometry Level 4

A right triangle has a hypotenuse of length 100cm and the length of the altitude dropped to the hypotenuse is 60cm . What is this triangle's area?

The question is flawed 30000mm^{2} 30m^{2} 3000cm^{2} All of the above

Kartik Agarwal by Kartik Agarwal , 20, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

Shaun Leong
Jan 16, 2016

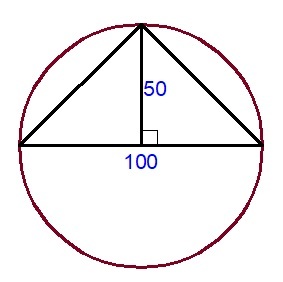
If such a triangle exists, we have 10000 = c 2 = a 2 + b 2 ≥ 2 a b = 4 ( 1 2 a b ) = 12000 10000=c^2=a^2+b^2 \geq 2ab=4 (\frac {1}{2}ab)=12000 This is a contradiction which follows from the AM-GM Inequality.

Hence no such triangle exists.

3 Helpful 0 Interesting 0 Brilliant 0 Confused

Indeed. Since the hypotenuse of a right triangle corresponds to a diameter of its circumcircle, the altitude to the hypotenuse cannot exceed the radius of the circimcircle, i.e., half the length of the hypotenuse.

Brian Charlesworth - 5 years, 5 months ago

A triangle inscribed in a circle where one side is the diameter of the circle is a right triangle. Based from the diagram above, the maximum possible altitude to the hypotenuse is 50 50 .

0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...