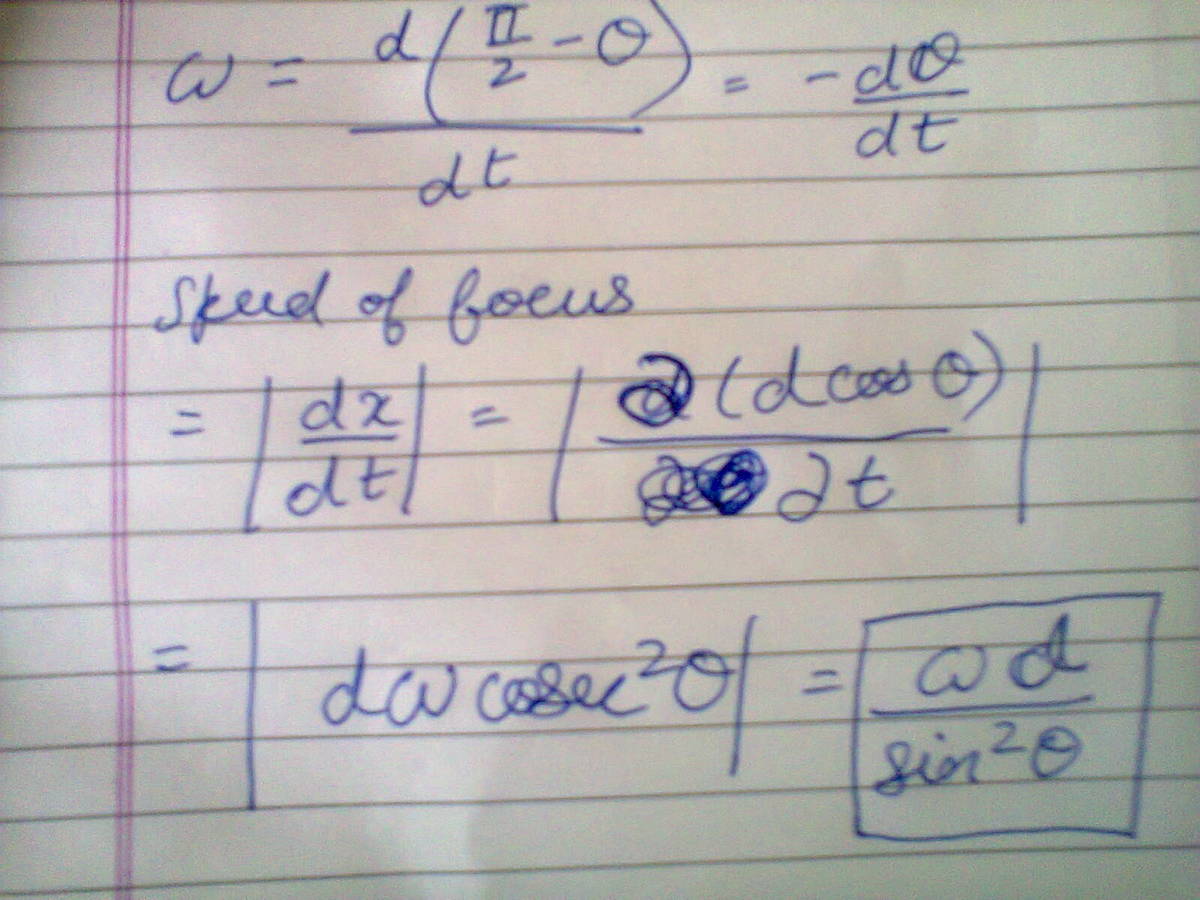Omega Mechanics
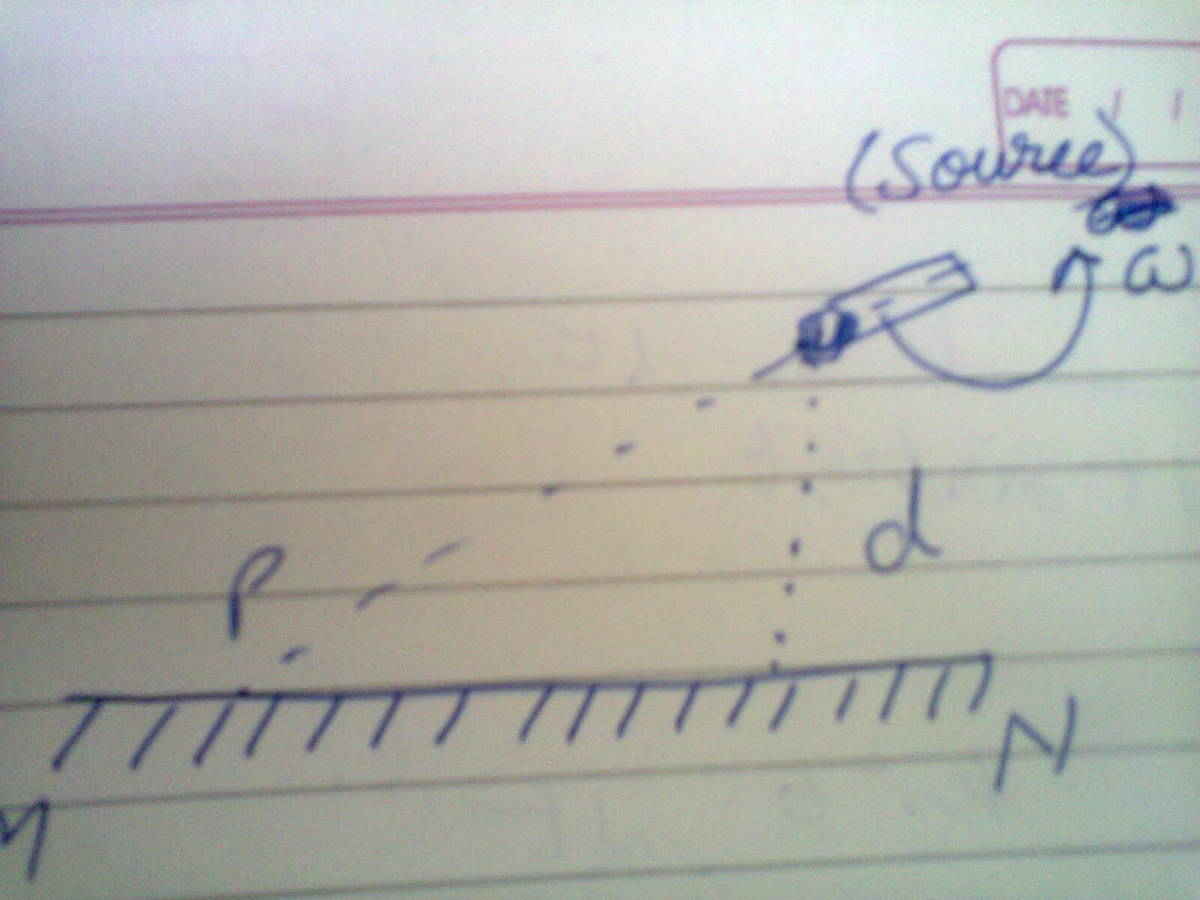
A point source of light is rotating in a horizontal plane at a speed of rads/sec. There is a wall at a distance from the source. At some instant the focus of the light is at P and SPN = . Speed of the focus at this instant in terms of is :
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.