Only Altitudes?
A triangle has altitudes of length 1 5 , 2 1 and 3 5 and area of M 3 square units.
Find the value of M .
The answer is 245.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
good approach..+1
That is a nice result sir! Did not know it, but now see how it works. I had taken the LCM 105 of 15, 21 & 35 to get the sides (a:b:c = 7:5:3) Then used Heron's formula.
Log in to reply
I never thought that way. But your method is far better since it is simple directly from fundamentals as it is normal with you. Congratulations.
Can you please post the solution ? Thanks.
The product of any altitude and the corresponding base is constant because it is twice the area of the triangle. For convenience, this product can be taken as 1 0 5 x as 105 is the LCM of 15, 21 and 35
Yielding an area of A = 2 1 0 5 x . . . (I)
i.e. 1 5 × b 1 = 2 1 × b 2 = 3 5 × b 3 = 1 0 5 x ⇒ ( b 1 , b 2 , b 3 ) = ( 7 x , 5 x , 3 x )
Giving semiperimeter S = ( 7 x + 5 x + 3 x ) / 2 = 1 5 x / 2
From Heron's formula A = S × ( S − 7 x ) × ( S − 5 x ) × ( S − 3 x ) = 4 1 5 3 x 2 . . . . .(II)
Equating (I) and (II) ⇒ x = 3 1 4 3 and area A = 2 4 5 3 ⇒ M = 245
Thank you. Very prompt reply.
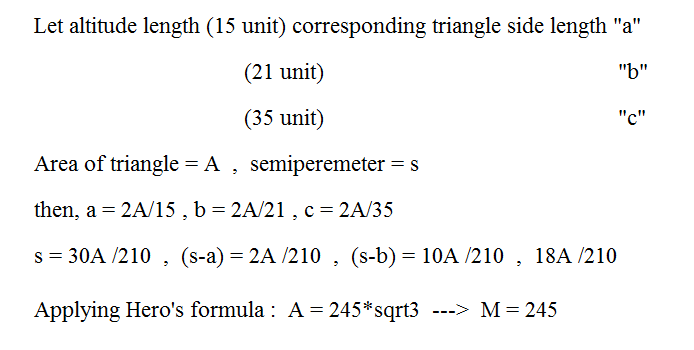
Let x=15, y=21 and z=35 be the altitudes, p reciprocal of x, q of y, and r of z.
Let H = 1/2 * (p + q+ r). It is well known that reciprocal of area= 4 * H ∗ ( H − p ) ∗ ( H − q ) ∗ ( H − r ) .
Putting the values, we get 245 * 3 .
So M = 245.
The approach suggested by Ujjwal Rane, is far better so I am requesting him to post his solution.