Origami Fun 2

Following the labelling shown on the figure and due to symmetry, we have $AH=HF$ and $\triangle ADE=\triangle FDE$ .
Moreover, $\left[ ADE \right]=\left[ FDE \right]={{A}_{1}}+{{A}_{2}}$ and $\left[ ADE \right]+\left[ FDE \right]+{{A}_{1}}+{{A}_{2}}=\left[ ABC \right]$ thus, $\left[ ADE \right]=\frac{1}{3}\left[ ABC \right]$ Let $\triangle ABC$ be of unit side length and denote $AH$ by $x$ .
Then, on $\triangle AMF$ ,

$AF=\frac{AM}{\cos a}\Rightarrow 2x=\frac{\frac{\sqrt{3}}{2}}{\cos a}\Rightarrow {{x}^{2}}=\frac{3}{16}\cdot \frac{1}{{{\cos }^{2}}a}\Rightarrow {{x}^{2}}=\frac{3}{16}\cdot \left( 1+{{\tan }^{2}}a \right)$ Consequently,

$\begin{aligned} \left[ ADE \right] & =\frac{1}{3}\cdot \frac{\sqrt{3}}{4} \\ \frac{1}{2}DE\cdot AH & =\frac{\sqrt{3}}{12} \\ \left( DH+HE \right)\cdot AH & =\frac{\sqrt{3}}{6} \\ \left( x\tan \left( \frac{\pi }{6}+a \right)+x\tan \left( \frac{\pi }{6}-a \right) \right)\cdot x & =\frac{\sqrt{3}}{6} \\ {{x}^{2}}\left( \frac{\tan \frac{\pi }{6}+\tan a}{1-\tan \frac{\pi }{6}\cdot \tan a}+\frac{\tan \frac{\pi }{6}-\tan a}{1+\tan \frac{\pi }{6}\cdot \tan a} \right) & =\frac{\sqrt{3}}{6} \\ \frac{3}{16}\left( 1+{{\tan }^{2}}a \right)\left( 2\cdot \frac{1+{{\tan }^{2}}a}{1-\frac{1}{3}\cdot {{\tan }^{2}}a} \right) & =\frac{\sqrt{3}}{6} \ \ \ \ \ (1)\\ \end{aligned}$ Setting $y={{\tan }^{2}}a$ , $\left( 1 \right)\Leftrightarrow \frac{3}{16}\left( 1+y \right)\left( 2\cdot \frac{1+y}{1-\frac{1}{3}y} \right)=\frac{\sqrt{3}}{6}$ which simplifies to $9{{y}^{2}}+22y-3=0$ Since $y>0$ we get $y=\dfrac{2\sqrt{37}-11}{9}$ Thus, $\tan a=\frac{\sqrt{2\sqrt{37}-11}}{3}\Rightarrow a={{\tan }^{-1}}\left( \frac{\sqrt{2\sqrt{37}-11}}{3} \right)\approx 0.3454360226$ For the answer, $\left\lfloor {{10}^{5}}a \right\rfloor =\boxed{34543}$ .

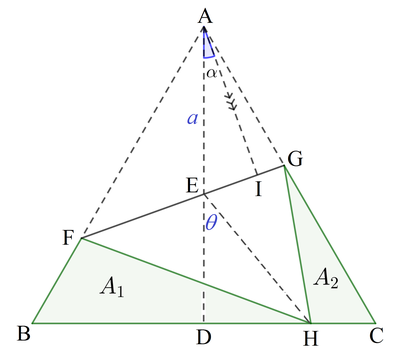
First note that $\triangle AFG$ and $\triangle FGH$ are congruent. Then $3(A_1 + A_2) = A_\triangle$ , where $A_\triangle$ is the area of $\triangle ABC$ . Let the side length of the equilateral triangular paper be $2$ . Then $A_\triangle = \dfrac {2^2 \sin 60^\circ}2 = \sqrt 3$ and $A_1 + A_2 = \dfrac 1{\sqrt 3}$ .

Since $AB=BC=CA=2$ , $AD=\sqrt 3$ . Let $AE = a$ , then $EH=AE=a$ and $ED = \sqrt 3 -a$ . Let $\angle DEH = \theta$ . Then $\angle AEG = \dfrac 12 \angle AEH = 90^\circ - \dfrac \theta 2$ . Since $\angle AEG = 90^\circ - \alpha$ also, then $\theta = 2\alpha$ and $\cos 2\alpha = \dfrac {ED}{EH} = \dfrac {\sqrt 3 -a}a$ .

Note that $\angle AGE = 60^\circ + \alpha$ and $\angle AFE = 60^\circ - \alpha$ . By sine rule , $\dfrac {EF}{\sin 30^\circ} = \dfrac a{\sin (60^\circ-\alpha)}$ and $\dfrac {EG}{\sin 30^\circ} = \dfrac a{\sin (60^\circ+\alpha)}$ . And the area $[FGH]=[AFG]$ is given by:

$\begin{aligned} [AFG] & = \frac {AI \cdot FG}2 \\ A_1 + A_2 & = \frac {a \cos \alpha}2 \cdot (EF+EG) \\ \frac 1{\sqrt 3} & = \frac {a^2 \cos \alpha}4 \left(\frac 1{\sin (60^\circ - \alpha)} + \frac 1{\sin (60^\circ + \alpha)} \right) & \small \blue{\text{As }\cos 2\alpha = \frac {\sqrt 3 -a}a} \\ & = \frac {3(\sin(60^\circ + \alpha) + \sin (60^\circ - \alpha))}{16 \cos^3 \alpha\sin(60^\circ + \alpha)\sin (60^\circ - \alpha)} \\ 8 \cos^3 \alpha (\cos 2\alpha - \cos 120^\circ) & = 9\cos \alpha \\ 4(\cos 2 \alpha + 1) \left(\cos 2\alpha + \frac 12 \right) & = 9 \\ 2(\cos 2\alpha+1)(2\cos 2\alpha + 1) & = 9 \\ 4\cos^2 2\alpha + 6 \cos 2 \alpha - 7 & = 0 \\ \implies \cos 2 \alpha & = \frac {\sqrt{37}-3}4 \\ \alpha & \approx 0.345436023 \\ \implies \lfloor 10^5 \alpha \rfloor & = \boxed{34543} \end{aligned}$

Let the sides of the equilateral triangle be $1$ and label the diagram as follows:

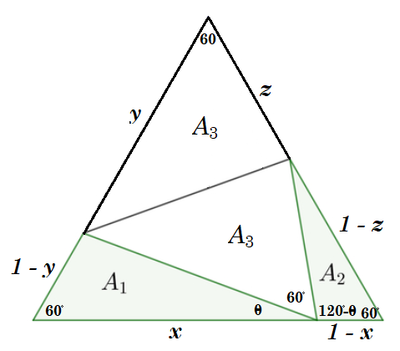
From the area of the equilateral triangle, $A_1 + A_2 + 2A_3 = \cfrac{1}{2} \cdot 1 \cdot 1 \cdot \sin 60° = \cfrac{\sqrt{3}}{4}$ , and from the given information, $A_1 + A_2 = A_3$ . Combining these equations gives $A_3 = \cfrac{\sqrt{3}}{12}$ .

Also,

$A_1 = \cfrac{1}{2} \cdot x \cdot (1 - y) \cdot \sin 60° = \cfrac{\sqrt{3}}{4}x(1 - y)$

$A_2 = \cfrac{1}{2} \cdot (1 - x) \cdot (1 - z) \cdot \sin 60° = \cfrac{\sqrt{3}}{4}x(1 - x)(1 - z)$

$A_3 = \cfrac{1}{2} \cdot y \cdot z \cdot \sin 60° = \cfrac{\sqrt{3}}{4}yz = \cfrac{\sqrt{3}}{12}$

Since $A_1 + A_2 = A_3$ , $\cfrac{\sqrt{3}}{4}x(1 - y) + \cfrac{\sqrt{3}}{4}x(1 - x)(1 - z) = \cfrac{\sqrt{3}}{4}yz = \cfrac{\sqrt{3}}{12}$ , or after simplifying,

$3x(1 - y) + 3(1 - x)(1 - z) = 1$

$3yz = 1$

By the law of sines on $A_1$ , $\cfrac{\sin \theta}{1 -y} = \cfrac{\sin 60°}{y}$ , and if $s = \sin \theta$ , this simplifies to:

$2sy = \sqrt{3}(1 - y)$

By the law of sines on $A_2$ , $\cfrac{\sin (120° - \theta)}{1 -z} = \cfrac{\sin 60°}{z}$ , and since $\sin (120° - \theta) = \sin 120° \cos \theta - \cos 120° \sin \theta = \frac{1}{2}(\sqrt{3} \cos \theta + \sin \theta) = \frac{1}{2}(\sqrt{3(1 - \sin^2 \theta)} + \sin \theta)$ , if $s = \sin \theta$ this simplifies to:

$z\sqrt{3(1 - s^2)} + sz = (1 - z)\sqrt{3}$

The four equations solve numerically to $s \approx 0.348833$ , $x \approx 0.811652$ , $y \approx 0.712861$ , and $z \approx 0.467599$ .

Now applying some trigonometry to $\triangle PQR$ that is labeled below:

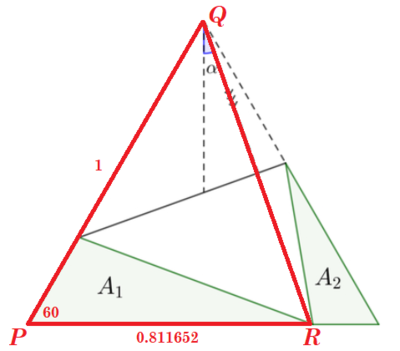
By the law of cosines, $QR = \sqrt{PQ^2 + PR^2 - 2 \cdot PQ \cdot PR \cdot \cos 60°} \approx \sqrt{1^2 + 0.811652^2 - 2 \cdot 1 \cdot 0.811652 \cdot \frac{1}{2}} \approx 0.920395$ .

And also by the law of cosines, $PR = \sqrt{PQ^2 + QR^2 - 2 \cdot PQ \cdot QR \cdot \cos \angle Q}$ , or $0.811652 \approx \sqrt{1^2 + 0.920395^2 - 2 \cdot 1 \cdot 0.920395 \cdot \cos (30° + \alpha)}$ , which solves to $\alpha \approx 0.345436$ .

Therefore, $\lfloor 10^5 \alpha \rfloor = \lfloor 10^5 \cdot 0.345436 \rfloor = \boxed{34543}$ .