Origami Logic Part 1
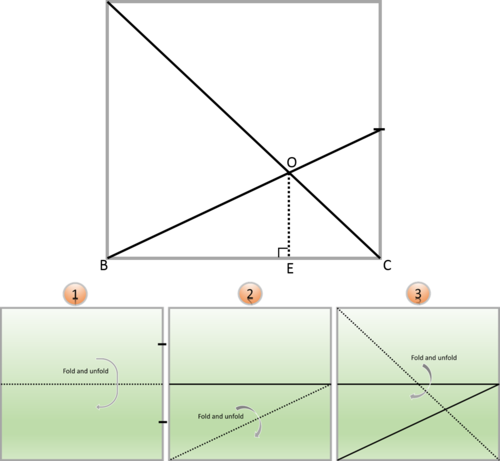 Stated in the picture is how to divide a paper into equal pieces.
What is the ratio of the length BE to length EC?
Key in your answers in the form:
Stated in the picture is how to divide a paper into equal pieces.
What is the ratio of the length BE to length EC?
Key in your answers in the form:
The answer is 3.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Consider this image: https://docs.google.com/document/d/1CVOOfHrMjD81taOO6fp_f-Y3AdEeIZfCzv4cEVtYEuw/edit
W e c a n f i n d θ 1 a n d θ 2 t a n θ 1 = 2 x x = 0 . 5 θ 1 = t a n − 1 0 . 5 θ 2 = 1 8 0 − 4 5 − θ 1 = 1 3 5 − t a n − 1 0 . 5 U s i n g t h e S i n e R u l e , w e c a n f i n d y 2 S i n e r u l e : s i n θ 2 2 x = s i n θ 1 y y = s i n θ 2 2 x s i n θ 1 W e c a n f i n d y 2 t o o , u s i n g P y t h a g o r a s t h e o r e m . y 1 = 8 x 2 S o , i f y o u t r y : y 2 y 1 = 3