Orthogonal Circles
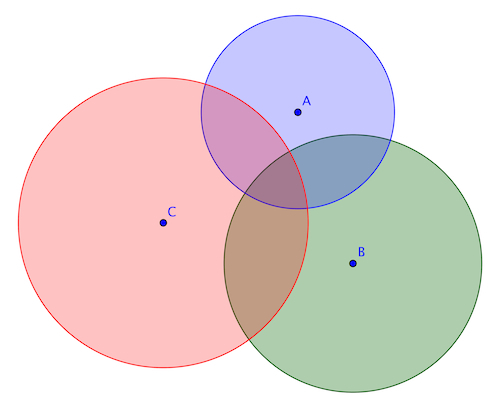Three mutually orthogonal circles, centered at points
A
,
B
, and
C
, have radii of 6, 8, and 9 respectively. Find the area of
Δ
A
B
C
.
Two circles are orthogonal to each other if a) they intersect in two points, and b) the two lines tangent to the two circles at each intersection point are perpendicular. So three or more circles are mutually orthogonal if every pair of them are orthogonal to each other.
The answer is 51.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
Well there is a way to find the area without working with any radicals.
We will use the nice length 1 0 to our advantage. Say that B C = 1 0 , A B = 1 1 7 , A C = 1 4 5 .
Drop the perpendicular from A onto B C at D . By Pythagorean theorem we get: A C 2 − A B 2 = 2 8 = C D 2 − B D 2 = ( C D + B D ) ( C D − B D ) = 1 0 ( C D − B D ) ⇒ C D − B D = 5 1 4 Since C D + B D = 1 0 we can solve for either C D or B D which means we can use pythagorean theorem to find the height and yeah...
Log in to reply
Congratulations. You think out of the box. Thanks.
√(6²+8²)= 10.0 √(6²+9²)= 10. 817 √(8²+9²)= 12. 042 s=((10.0+ 10. 817+12. 042)/2)= 16. 430 A=√( 16. 430( 16. 430- 10.0)( 16. 430- 10. 817)( 16. 430- 12. 042))= 51. 010 Integer=51
The nice thing about bashy solutions is that you often get to the answer quicker. Tight, refined, short elegant solutions take all day to craft---usually after you've already solved it first by bashing.
Log in to reply
And with Wolfram Alpha, it's even faster.
Not saying I used wolfram alpha to calculate Heron's Formula. Repeated difference of squares will solve it relatively quickly.
I drew a trapezium using the diameter of A to the intersections of the other 2 circles and the line BC. Then it is just a matter of calculating the area of the trapezium and the 2 triangles around ABC (it is easy with the diagram...)
Same Solution. It is pretty easy if you analyze the figure and problem very carefully.
Heron's formula
it's the same solution that I find
Did the same way.
Bashy solution alert:
Connect A and B with the outer intersection of the two corresponding circles as shown.
We see that since the circles are mutually orthogonal, the angles that are marked are right angles. Thus, by using the Pythagorean Theorem, we see that the sides of △ A B C are 1 0 , 1 1 7 , 1 4 5 .
By Heron's Formula, we see that [ A B C ] = ( 2 1 0 + 1 1 7 + 1 4 5 ) ( 2 1 0 + 1 1 7 − 1 4 5 ) ( 2 1 0 − 1 1 7 + 1 4 5 ) ( 2 − 1 0 + 1 1 7 + 1 4 5 ) which miraculously simplifies into 5 1 .
Definitely a better solution, but I don't see it.