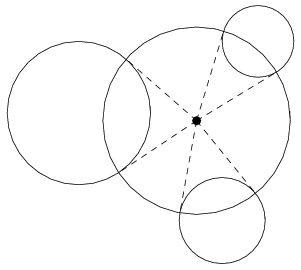
Orthogonal Circles

Geometry Level 5

( x − 153 ) 2 + y 2 = 18 5 2 ( x + 153 ) 2 + y 2 = 18 5 2 x 2 + ( y − 1380 ) 2 = 19 6 2 \large{\begin{aligned} (x-153)^2+y^2&=&185^2 \\ (x+153)^2+y^2&=&185^2 \\ x^2+(y-1380)^2&=&196^2 \\ \end{aligned} }

Above shows the equations for 3 circles.

Two circles are orthogonal to each other if where they intersect meets at right angles. The circle that is orthogonal to all three of the circles above can be written as:

x 2 + ( y − k ) 2 = r 2 . x^2+(y-k)^2=r^2.

Find k + r k+r .


The answer is 1352.

View wiki
Garrett Clarke by Garrett Clarke , 25, USA

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Chew-Seong Cheong
Jul 13, 2015

Two circles x 2 + y 2 + 2 g x + 2 f y + c = 0 x^2 + y^2 + 2gx + 2fy + c = 0 and x 2 + y 2 + 2 g ′ x + 2 f ′ y + c ′ = 0 x^2 + y^2 + 2g'x + 2f'y + c' = 0 are orthogonal if 2 g g ′ + 2 f f ′ = c + c ′ 2gg' + 2ff' = c + c' (see Orthogonal Circles ).

Now we have:

{ ( x − 153 ) 2 + y 2 = 18 5 2 ⇒ x 2 + y 2 − 306 x − 10816 = 0 . . . ( 1 ) ( x + 153 ) 2 + y 2 = 18 5 2 ⇒ x 2 + y 2 + 306 x − 10816 = 0 . . . ( 2 ) x 2 + ( y − 1380 ) 2 = 19 6 2 ⇒ x 2 + y 2 − 2760 y + 1865984 = 0 . . . ( 3 ) x 2 + ( y − k ) 2 = r 2 ⇒ x 2 + y 2 − 2 k y + k 2 − r 2 = 0 . . . ( 4 ) \begin{cases} (x-153)^2 + y^2 = 185^2 & \Rightarrow x^2 + y^2 -306x -10816 = 0 &...(1) \\ (x+153)^2 + y^2 = 185^2 & \Rightarrow x^2 + y^2 +306x -10816 = 0 &...(2) \\ x^2 + (y-1380)^2 = 196^2 & \Rightarrow x^2 + y^2 -2760y + 1865984 = 0 &...(3) \\ x^2 + (y - k)^2 = r^2 & \Rightarrow x^2 + y^2 -2ky + k^2-r^2 = 0 &...(4) \end{cases}

Considering 2 g g ′ + 2 f f ′ = c + c ′ 2gg' + 2ff' = c + c' , we have

{ ( 1 ) & ( 4 ) : 0 = k 2 − r 2 − 10816 ⇒ k 2 − r 2 = 10816 . . . ( 5 ) ( 3 ) & ( 4 ) : 2760 k = k 2 − r 2 + 1865984 = 10816 + 1865984 ⇒ k = 680 ( 5 ) : k 2 − r 2 = 10816 ⇒ r 2 = 68 0 2 − 10816 ⇒ r = 672 \begin{cases} (1)\&(4): & 0 = k^2 - r^2 - 10816 \quad \Rightarrow \color{#3D99F6} {k^2 - r^2 = 10816} \quad ...(5) \\ (3)\&(4): & 2760k = \color{#3D99F6}{k^2 - r^2} + 1865984 = \color{#3D99F6}{10816} + 1865984 \quad \Rightarrow \color{#D61F06}{k = 680} \\ (5): & \color{#D61F06}{k^2} - r^2 = 10816 \quad \Rightarrow r^2 = \color{#D61F06}{680^2} - 10816 \quad \Rightarrow r = 672 \end{cases}

⇒ k + r = 680 + 672 = 1352 \Rightarrow k + r = 680 + 672 = \boxed{1352}

1 Helpful 0 Interesting 0 Brilliant 0 Confused

Moderator note:

What is special about this center?

Hint: Power of a point.

I believe you meant that r = 672 r=672 , not r = 627 r=627 . Great solution though!

Garrett Clarke - 5 years, 11 months ago

Log in to reply

Thanks. I have changed it.

Chew-Seong Cheong - 5 years, 11 months ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...