Oscillating Ring!
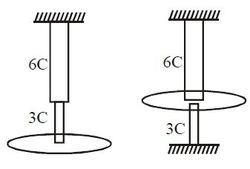 A disc of mass
and radius
is fastened with two rods of torsional constant
and
as shown. Time period of small oscillation of disc about it's axis in the left case and right case are respectively
and
.
A disc of mass
and radius
is fastened with two rods of torsional constant
and
as shown. Time period of small oscillation of disc about it's axis in the left case and right case are respectively
and
.
What is the value of is?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
The equivalent single torsional constant at LEFT is C 1 = 3 C 1 + 6 C 1 1 = 2 C . a t R I G H T i s C 2 = 3 C + 6 C = 9 C . T 1 2 ∗ C 1 = T 2 2 ∗ C 2 . ∴ T 2 2 T 1 2 = 2 9 .