Overlapped Squares Troubles

$BD^2=1^2+1^2 \Rightarrow BD=\sqrt{2}$

$ED=BD-1=\sqrt{2}-1$

$EF=\sqrt{2}-1$

Shaded area is $|\triangle BCD|-|\triangle EDF|$ .

$|\triangle BCD|=\frac{1\times1}{2}=\frac{1}{2}$

$|\triangle EDF|=\frac{EF \times ED}{2}=\frac{(\sqrt{2}-1)^2}{2}=\frac{3-2\sqrt{2}}{2}$

$|\triangle BCD|-|\triangle EDF|=\frac{1}{2}-\frac{3-2\sqrt{2}}{2}=\boxed{\sqrt{2}-1}$ .

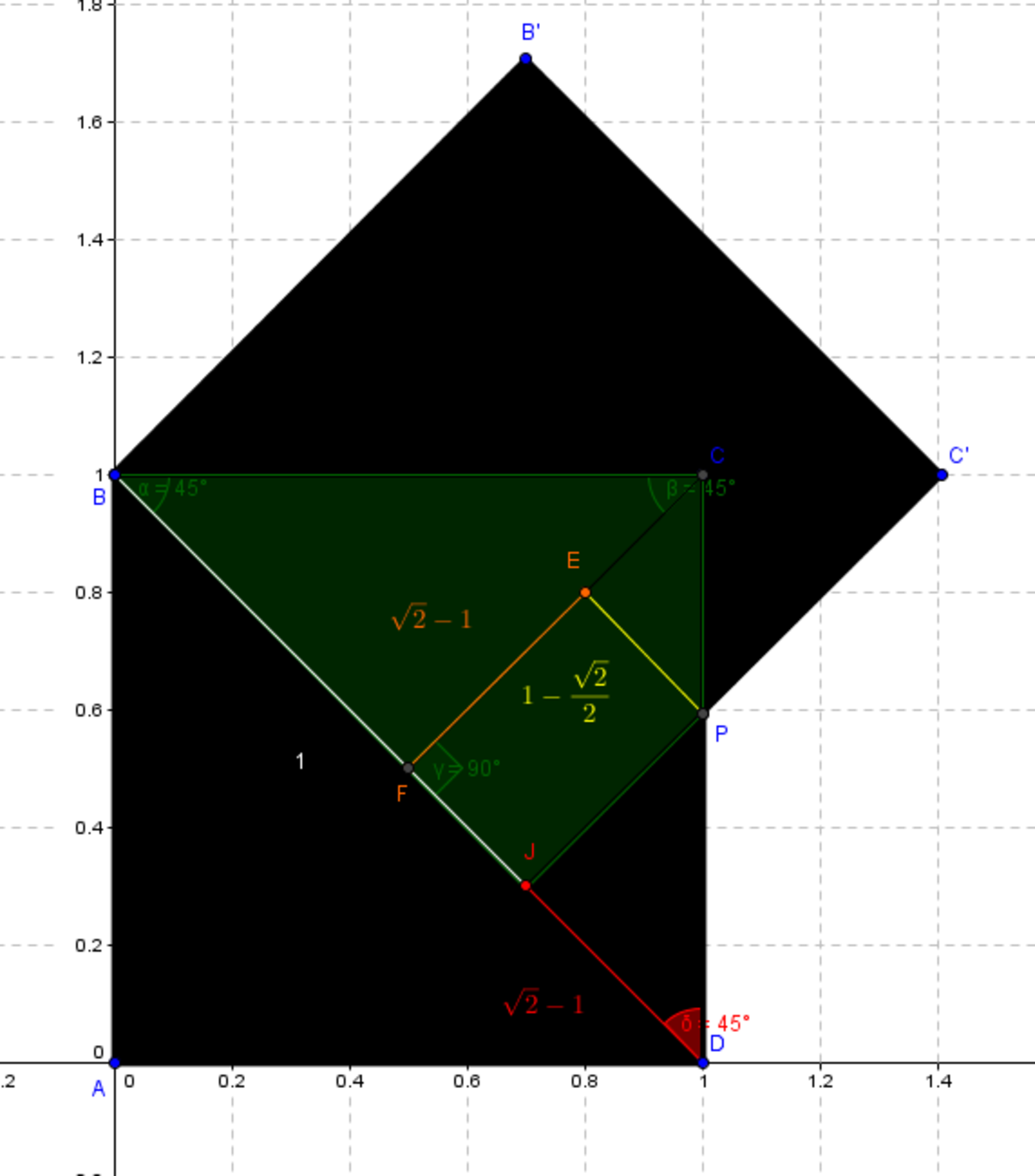
$\text{1. Plot segments BD and AC} \\\text{2. BD and AC intersect at point F} \\\text{3. BD=}\space\sqrt{2} \\\text{4. BF=}\space\dfrac{\sqrt{2}}{2} \\\text{5. FD =}\space\dfrac{\sqrt{2}}{2} \\\text{6. CF =}\space\dfrac{\sqrt{2}}{2} \\\text{7. BJ = 1} \\\text{8. JD =}\space \sqrt{2} - 1 \\\text{9. FJ =}\space \dfrac{\sqrt{2}}{2} - \left(\sqrt{2} - 1\right) = 1 - \dfrac{\sqrt{2}}{2} \\\text{10. Plot EP perpendicular to AC } \\\text{11.} EP \cong FJ \\\text{12.} EP = 1 - \dfrac{\sqrt{2}}{2} \\\text{13}. JP \perp BD \\\text{14.}JP \cong JD \\\text{15.} JP = \sqrt{2} - 1 \\\text{16.} EC \cong EP$

17) Area of Shaded Region = Area of $\triangle{FBC}$ + Area of $\triangle ECP +$ Area of ▱ EFJP 18) Area of Shaded Region = $\dfrac{1}{4} + \left(\dfrac{3}{4} - \dfrac{\sqrt{2}}{2}\right) + \left(\dfrac{3\sqrt{2}}{2} - 2\right) = \sqrt{2} - 1$

There's an intuitive guess with this problem due to the poor multiple choice options. I am quite lazy, so that's how I solved it quickly.

We know that the area of the shaded area must be less than $\frac {1}{2}$ .

$\frac{\sqrt{2}}{2} > \frac{1}{2}$ , thus not the answer.

$\sqrt{3} - \sqrt{2}$ , $\sqrt{3}$ seems impossible to get from the squares, therefore not a plausible answer.

$\frac{\sqrt{2} + 1}{2} > \frac{1}{2}$ , thus not the answer.

$\sqrt{2} + 1 > \frac{1}{2}$ , thus not the answer.

$\sqrt{2} - 1 < \frac{1}{2}$ , the only one that is plausible, thus the answer.

(1/2) -(1/2) (square root of 2 - 1)^2 =

(square root of 2) - 1