Parallelogramatic

Geometry Level 2

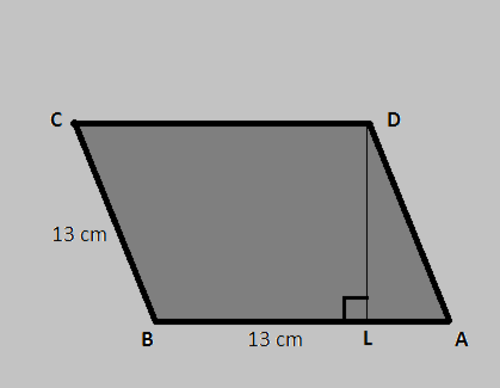
In the given figure, ABCD is a parallelogram. If the area of the parallelogram is 156 sq cm, find the length of AL.


The answer is 5.

Arpan Ray by Arpan Ray , 17, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Arpan Ray
Sep 29, 2016

Area of the parallelogram = 156 sq cm

[Area of a normal parallelogram = b a s e base X h e i g h t height ]

Base = 13 cm

Height (DL) = A r e a B a s e \frac{Area}{Base} = 156 13 \frac{156}{13} = 12 cm

DLA is a right- angle triangle.

Hypotenuse (DA) = 13cm

Altitude (DL) = 12 cm

Base (AL) = ??

{According to Pythagoras Theorem}

= ( A l t i t u d e ) (Altitude) 2 \frac{2}{} + ( B a s e ) (Base) 2 \frac{2}{} = ( H y p o t e n u s e ) (Hypotenuse) 2 \frac{2}{} [Solving it]

= ( 12 ) (12) 2 \frac{2}{} + ( A L ) (AL) 2 \frac{2}{} = ( 13 ) (13) 2 \frac{2}{}

= 144 + 144 + ( A L ) (AL) 2 \frac{2}{} = 169 = 169 [Shifting 144 from L.H.S. to R.H.S.]

= ( A L ) (AL) 2 \frac{2}{} = 169 − 144 = 169 - 144

= ( A L ) (AL) 2 \frac{2}{} = 25 = 25 [Square root on both sides]

= √ √ ( A L ) (AL) 2 \frac{2}{} = √ 25 = √25

= √ √ A L AL X A L AL = √ = √ 5 5 X 5 5

= A L = 5 c m AL = 5 cm = A n s Ans \

0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...