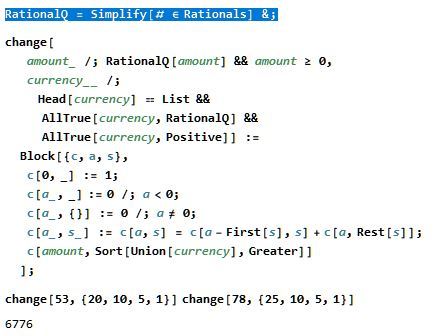
Paying with cash - part III
At a gas station I had to pay $53.78 with cash for gas, whiskey and sandwich. With plenty of pennies, nickels, dimes, quarters and paper money ($1 bill, $5 bills, $10 bills, $20 bills) in my pockets I can pay in any possible way $53 with paper bills, 78 cents with coins. In how many different ways can I pay with the exact amount of money?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions

6
=
2
6
∗
3
2
=
1
8
∗
1
6
;
3
0
2
4
=
2
4
∗
3
3
∗
7
=
5
6
∗
5
4
;
6
7
7
6
=
2
3
∗
7
∗
1
1
2
=
5
6
∗
1
2
1
;
7
8
9
0
=
2
∗
3
∗
5
∗
2
6
3
.
3
$
o
f
5
3
$
a
n
d
3
c
e
n
t
s
o
f
7
8
c
e
n
t
s
w
i
l
l
b
e
i
n
c
l
u
d
e
d
i
n
a
l
l
p
a
y
m
e
n
t
s
,
s
o
s
u
f
f
i
c
e
t
o
l
o
o
k
a
t
5
0
$
a
n
d
7
5
c
e
n
t
s
.
D
i
v
i
d
e
5
3
$
p
a
y
m
e
n
t
s
i
n
t
o
t
h
r
e
e
g
r
o
u
p
s
A
,
B
,
C
,
a
n
d
7
8
c
e
n
t
s
i
n
t
o
f
o
u
r
g
r
o
u
p
s
W
,
X
,
Y
,
Z
.
m
g
r
o
u
p
i
s
t
h
e
m
a
x
i
m
u
m
p
o
s
s
i
b
l
e
5
$
b
i
l
l
s
o
r
n
i
c
k
e
l
s
i
n
t
h
e
g
i
v
e
n
g
r
o
u
p
.
T
h
i
s
i
s
w
h
e
n
t
h
e
p
a
y
m
e
n
t
h
a
s
n
o
1
0
$
i
n
5
3
$
o
r
n
o
d
i
m
e
i
n
7
8
c
e
n
t
s
.
A
.
.
.
.
.
.
.
I
t
c
o
n
t
a
i
n
s
n
o
2
0
$
b
i
l
l
s
:
−
N
e
e
d
5
3
$
∴
c
o
n
t
r
i
b
u
t
i
o
n
b
y
1
0
$
b
i
l
l
s
+
5
$
b
i
l
l
s
s
h
o
u
l
d
b
e
f
r
o
m
0
$
t
o
5
0
$
a
n
d
t
h
e
s
h
o
r
t
f
a
l
l
f
r
o
m
5
3
$
i
s
s
u
p
p
l
i
e
d
b
y
1
$
b
i
l
l
s
.
t
t
h
e
n
u
m
b
e
r
o
f
1
0
$
b
i
l
l
s
w
o
u
l
d
b
e
f
r
o
m
0
t
o
5
.
C
o
r
r
e
s
p
o
n
d
i
n
g
f
t
h
e
n
u
m
b
e
r
o
f
5
$
b
i
l
l
s
w
o
u
l
d
b
e
f
r
o
m
0
t
o
(
1
0
−
2
t
)
.
m
A
=
1
0
.
B
.
.
.
.
.
.
.
I
t
c
o
n
t
a
i
n
s
o
n
e
2
0
$
b
i
l
l
s
:
−
N
e
e
d
3
3
$
∴
c
o
n
t
r
i
b
u
t
i
o
n
b
y
1
0
$
b
i
l
l
s
+
5
$
b
i
l
l
s
s
h
o
u
l
d
b
e
f
r
o
m
0
$
t
o
3
0
$
a
n
d
t
h
e
s
h
o
r
t
f
a
l
l
f
r
o
m
3
3
$
i
s
s
u
p
p
l
i
e
d
b
y
1
$
b
i
l
l
s
.
t
w
o
u
l
d
b
e
f
r
o
m
0
t
o
3
.
C
o
r
r
e
s
p
o
n
d
i
n
g
f
w
o
u
l
d
b
e
f
r
o
m
0
t
o
(
6
−
2
t
)
.
m
B
=
6
.
C
.
.
.
.
.
.
.
I
t
c
o
n
t
a
i
n
s
t
w
o
2
0
$
b
i
l
l
s
:
−
N
e
e
d
1
3
$
∴
C
o
n
t
r
i
b
u
t
i
o
n
b
y
1
0
$
b
i
l
l
s
+
5
$
b
i
l
l
s
s
h
o
u
l
d
b
e
f
r
o
m
0
$
t
o
1
0
$
a
n
d
t
h
e
s
h
o
r
t
f
a
l
l
f
r
o
m
1
3
$
i
s
s
u
p
p
l
i
e
d
b
y
1
$
b
i
l
l
s
.
t
w
o
u
l
d
b
e
f
r
o
m
0
t
o
1
.
C
o
r
r
e
s
p
o
n
d
i
n
g
f
w
o
u
l
d
b
e
f
r
o
m
0
t
o
(
2
−
2
t
)
.
m
C
=
2
.
W
.
.
.
.
.
.
.
I
t
c
o
n
t
a
i
n
s
n
o
Q
u
a
r
t
e
r
:
−
N
e
e
d
7
8
c
e
n
t
s
∴
C
o
n
t
r
i
b
u
t
i
o
n
b
y
d
i
m
e
s
+
n
i
c
k
e
l
s
s
h
o
u
l
d
b
e
f
r
o
m
0
t
o
7
5
.
a
n
d
t
h
e
s
h
o
r
t
f
a
l
l
f
r
o
m
7
8
c
e
n
t
s
i
s
s
u
p
p
l
i
e
d
b
y
p
e
n
n
i
e
s
.
t
t
h
e
n
u
m
b
e
r
o
f
d
i
m
e
s
w
o
u
l
d
b
e
f
r
o
m
0
t
o
7
.
C
o
r
r
e
s
p
o
n
d
i
n
g
f
t
h
e
n
u
m
b
e
r
o
f
n
i
c
k
e
l
s
w
o
u
l
d
b
e
f
r
o
m
0
t
o
(
1
5
−
2
t
)
.
m
A
=
1
5
.
X
.
.
.
.
.
.
.
I
t
c
o
n
t
a
i
n
s
o
n
e
Q
u
a
r
t
e
r
:
−
N
e
e
d
5
3
c
e
n
t
s
∴
C
o
n
t
r
i
b
u
t
i
o
n
b
y
d
i
m
e
s
+
n
i
c
k
e
l
s
s
h
o
u
l
d
b
e
f
r
o
m
0
t
o
5
0
.
a
n
d
t
h
e
s
h
o
r
t
f
a
l
l
f
r
o
m
7
8
c
e
n
t
s
i
s
s
u
p
p
l
i
e
d
b
y
p
e
n
n
i
e
s
.
t
w
o
u
l
d
b
e
f
r
o
m
0
t
o
5
.
C
o
r
r
e
s
p
o
n
d
i
n
g
f
w
o
u
l
d
b
e
f
r
o
m
0
t
o
(
1
0
−
2
t
)
.
m
A
=
1
0
.
Y
.
.
.
.
.
.
.
I
t
c
o
n
t
a
i
n
s
t
w
o
Q
u
a
r
t
e
r
:
−
N
e
e
d
2
8
c
e
n
t
s
∴
C
o
n
t
r
i
b
u
t
i
o
n
b
y
d
i
m
e
s
+
n
i
c
k
e
l
s
s
h
o
u
l
d
b
e
f
r
o
m
0
t
o
2
5
.
a
n
d
t
h
e
s
h
o
r
t
f
a
l
l
f
r
o
m
7
8
c
e
n
t
s
i
s
s
u
p
p
l
i
e
d
b
y
p
e
n
n
i
e
s
.
t
w
o
u
l
d
b
e
f
r
o
m
0
t
o
2
.
C
o
r
r
e
s
p
o
n
d
i
n
g
f
w
o
u
l
d
b
e
f
r
o
m
0
t
o
(
5
−
2
t
)
.
m
A
=
5
.
Z
.
.
.
.
.
.
.
I
t
c
o
n
t
a
i
n
s
t
h
r
e
e
Q
u
a
r
t
e
r
:
−
N
e
e
d
3
c
e
n
t
s
∴
T
h
e
r
e
a
r
e
n
o
d
i
m
e
s
o
r
n
i
c
k
e
l
s
b
u
t
t
h
r
e
e
p
e
n
n
i
e
s
.
m
A
=
1
.
N
t
h
e
n
u
m
b
e
r
o
f
w
a
y
s
w
e
c
a
n
p
a
y
,
d
e
p
e
n
d
s
o
n
m
g
r
o
u
p
.
F
o
r
e
v
e
n
m
g
r
o
u
p
,
N
=
(
2
m
g
r
o
u
p
+
1
)
2
.
F
o
r
o
d
d
m
g
r
o
u
p
,
N
=
(
2
m
g
r
o
u
p
+
1
)
2
+
2
m
g
r
o
u
p
+
1
N
A
=
(
2
1
0
+
1
)
2
=
3
6
N
B
=
(
2
6
+
1
)
2
=
1
6
.
N
C
=
(
2
2
+
1
)
2
=
4
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
N
5
3
=
3
6
+
1
6
+
4
=
5
6
.
N
W
=
(
2
1
5
+
1
)
2
+
2
1
5
+
1
=
6
4
+
8
=
7
2
N
X
=
(
2
1
0
+
1
)
2
=
3
6
.
N
Y
=
(
2
5
+
1
)
2
+
2
5
+
1
=
9
+
3
=
1
2
N
Z
=
1
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
N
7
8
=
7
2
+
3
6
+
1
2
+
1
=
1
2
1
.
N
5
6
∗
1
2
1
=
6
7
7
6
B
e
l
o
w
f
i
n
d
d
e
t
a
i
l
e
d
e
x
p
l
a
n
a
t
i
o
n
.
I
f
I
g
e
t
t
i
m
e
I
w
i
l
l
m
a
k
e
i
t
o
r
d
e
r
l
y
6
=
2
6
∗
3
2
=
1
8
∗
1
6
;
3
0
2
4
=
2
4
∗
3
3
∗
7
=
5
6
∗
5
4
;
6
7
7
6
=
2
3
∗
7
∗
1
1
2
=
5
6
∗
1
2
1
;
7
8
9
0
=
2
∗
3
∗
5
∗
2
6
3
.
L
e
t
p
⟹
p
e
n
n
i
e
s
.
.
.
.
.
.
.
.
.
.
n
⟹
n
i
c
k
e
l
s
.
.
.
.
.
.
.
.
.
.
d
⟹
d
i
m
s
.
.
.
.
.
.
.
.
.
q
⟹
Q
u
a
r
t
e
r
s
.
V
a
r
i
o
u
s
c
o
m
b
i
n
a
t
i
o
n
s
o
f
2
0
$
,
1
0
$
,
a
n
d
5
$
b
i
l
l
s
c
a
n
a
d
d
u
p
f
r
o
m
5
$
t
o
5
0
$
i
n
m
u
l
t
i
p
l
e
s
o
f
5
$
.
p
l
u
s
p
1
$
b
i
l
l
c
a
n
g
i
v
e
a
t
o
t
a
l
o
f
5
3
$
.
∴
i
t
i
s
s
u
f
f
i
c
i
e
n
t
t
o
f
i
x
2
0
$
,
1
0
$
a
n
d
5
$
b
i
l
l
s
.
n
.
m
m
e
a
n
s
n
o
f
m
$
.
D
i
v
i
d
e
0
$
t
o
5
0
$
i
n
t
o
t
h
r
e
e
g
r
o
u
p
s
A
,
B
,
C
.
A
0
.
2
0
+
t
.
1
0
+
f
.
5
+
p
.
1
.
C
o
n
t
r
i
b
u
t
i
o
n
o
f
1
0
a
n
d
5
$
b
i
l
l
s
i
s
0
$
t
o
5
0
$
i
n
m
u
l
t
i
p
l
e
s
o
f
5
$
.
t
t
h
e
r
a
n
g
e
o
f
1
0
$
b
i
l
l
s
i
s
t
=
0
t
o
5
.
f
t
h
e
r
a
n
g
e
o
f
5
$
b
i
l
l
s
i
s
f
=
0
t
o
(
1
0
−
2
t
)
t
h
a
t
i
s
0
.
5
t
o
(
1
0
−
2
t
)
.
5
p
w
o
u
l
d
c
o
n
t
r
i
b
u
t
e
t
o
t
h
e
s
h
o
r
t
f
a
l
l
f
r
o
m
5
3
$
a
s
s
h
o
w
n
i
n
t
a
b
l
e
f
o
r
A
i
n
d
e
t
a
i
l
.
B
1
.
2
0
+
t
.
1
0
+
f
.
5
+
p
.
1
.
C
o
n
t
r
i
b
u
t
i
o
n
o
f
1
0
a
n
d
5
$
b
i
l
l
s
i
s
0
$
t
o
3
0
$
i
n
m
u
l
t
i
p
l
e
s
o
f
5
$
.
t
=
0
t
o
3
.
f
=
0
t
o
(
6
−
2
t
)
p
w
o
u
l
d
c
o
n
t
r
i
b
u
t
e
t
o
t
h
e
s
h
o
r
t
f
a
l
l
f
r
o
m
3
3
$
a
s
i
n
t
a
b
l
e
f
o
r
B
.
C
2
.
2
0
+
t
.
1
0
+
f
.
5
p
+
p
.
1
.
C
o
n
t
r
i
b
u
t
i
o
n
o
f
1
0
a
n
d
5
$
b
i
l
l
s
i
s
$
.
0
$
t
o
1
0
$
i
n
m
u
l
t
i
p
l
e
s
o
f
5
$
.
t
=
0
t
o
1
.
f
=
0
t
o
(
2
−
2
t
)
p
w
o
u
l
d
c
o
n
t
r
i
b
u
t
e
t
o
t
h
e
s
h
o
r
t
f
a
l
l
f
r
o
m
1
3
$
a
s
i
n
t
a
b
l
e
f
o
r
C
.
G
r
o
u
p
A
B
C
N
u
m
b
e
r
o
f
2
0
$
+
0
1
2
t
=
N
u
m
b
e
r
o
f
1
0
$
+
0
1
2
3
4
5
0
1
2
3
0
1
f
=
N
u
m
b
e
r
o
f
5
$
+
0
1
2
3
4
5
6
7
8
9
1
0
0
1
2
3
4
5
6
7
8
0
1
2
3
4
5
6
0
1
2
3
4
0
1
2
0
0
1
2
3
4
5
6
0
1
2
3
4
0
1
2
0
0
1
2
0
N
u
m
b
e
r
o
f
1
$
5
3
4
8
4
3
3
8
3
3
2
8
2
3
1
8
1
3
8
3
4
3
3
8
3
3
2
8
2
3
1
8
1
3
8
3
3
3
2
8
2
3
1
8
1
3
8
3
2
3
1
8
1
3
8
3
1
3
8
3
3
3
3
2
8
2
3
1
8
1
3
8
3
2
3
1
8
1
3
8
3
1
3
8
3
3
1
3
8
3
3
W
a
y
s
1
2
3
4
5
6
7
8
9
1
0
1
1
1
2
3
4
5
6
7
8
9
1
2
3
4
5
6
7
1
2
3
4
5
1
2
3
1
1
2
3
4
5
6
7
1
2
3
4
5
1
2
3
1
1
2
3
1
A
=
1
1
+
9
+
7
+
5
+
3
+
1
=
3
6
=
(
2
1
0
+
1
)
2
.
B
=
7
+
5
+
3
+
1
=
1
6
=
(
2
6
+
1
)
2
.
C
=
3
+
1
=
4
=
(
2
2
+
1
)
2
.
N
o
t
e
:
I
f
i
n
a
g
r
o
u
p
,
m
t
h
e
m
a
x
i
m
u
m
5
$
b
i
l
l
(
o
r
n
i
c
k
e
l
s
)
c
a
n
c
o
n
t
r
i
b
u
t
e
i
s
e
v
e
n
,
t
h
e
n
t
h
e
n
u
m
b
e
r
o
f
w
a
y
s
=
(
2
m
+
1
)
2
.
I
f
i
n
a
g
r
o
u
p
,
m
t
h
e
m
a
x
i
m
u
m
5
$
b
i
l
l
(
o
r
n
i
c
k
e
l
s
)
c
a
n
c
o
n
t
r
i
b
u
t
e
i
s
o
d
d
,
t
h
e
n
t
h
e
n
u
m
b
e
r
o
f
w
a
y
s
=
(
2
m
+
1
)
2
+
2
m
+
1
=
4
1
∗
(
m
2
+
4
m
+
3
)
.
3
6
+
1
6
+
4
=
5
6
⟹
w
e
h
a
v
e
5
6
w
a
y
s
t
o
g
e
t
5
3
$
.
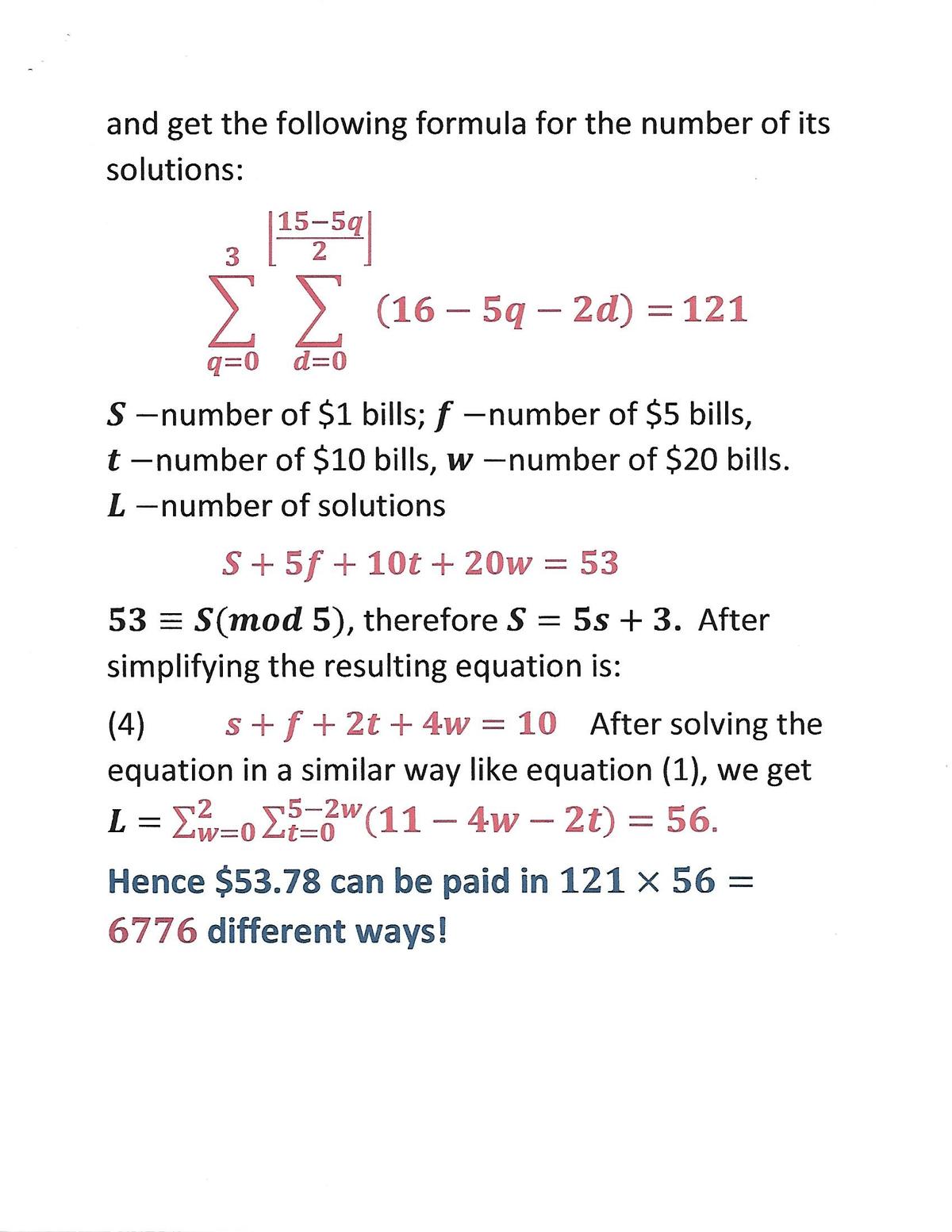
Total ways to get 53.78 $ ={ ways we get for 53 $= 56}*{ways we get .78 $.}
It is easy to see that
.
7
8
$
w
a
y
s
a
r
e
m
o
r
e
t
h
a
n
w
a
y
s
o
f
5
3
$
.
5 7 6 a n d 7 8 9 0 a r e n o t d i v i s i b l e b y 5 6 . S o c a n n o t b e t h e s o l u t i o n . 5 6 3 0 2 4 = 5 4 < 5 6 . S o c a n n o t b e t h e s o l u t i o n . I f t h e s o l u t i o n i s i n t h e g i v e n o p t i o n s 6 7 7 6 m u s t b e t h e s o l u t i o n . H o w e v e r o n t h e s a m e b a s e s b e l o w i s s h o r t p r o o f o f 1 2 1 w a y s w e c a n g e t 0 . 7 8 c e n t s .
L e t p ⟹ p e n n i e s . . . . . . . . . . n ⟹ n i c k e l s . . . . . . . . . . d ⟹ d i m s . . . . . . . . . q ⟹ Q u a r t e r s . G r o u p W n o Q u a r t e r . . . . . . . . . . t . d + f . n = 0 t o 7 5 c e n t s . + n e c e s s a r y n i c k e l s = 7 8 c e n t s . G r o u p X o n e Q u a r t e r . . . . . . . . t . d + f . n = 0 t o 5 0 c e n t s . + n e c e s s a r y n i c k e l s = 7 8 c e n t s . G r o u p Y t w o Q u a r t e r . . . . . . . . t . d + f . n = 0 t o 2 5 c e n t s . + n e c e s s a r y n i c k e l s = 7 8 c e n t s . G r o u p Z t h r e e Q u a r t e r . . . . . . t . d + f . n = 0 c e n t + 3 n i c k e l s = 7 8 c e n t s . G r o u p W X Y Z N u m b e r o f q 0 1 2 3 t = N u m b e r o f d 0 1 2 3 4 5 6 7 0 1 2 3 4 5 0 1 2 0 f = N u m b e r o f n 0 . n t o 1 5 . n 0 . n t o 1 3 . n 0 . n t o 1 1 . n 0 . n t o 9 . n 0 . n t o 7 . n 0 . n t o 5 . n 0 . n t o 3 . n 0 . n t o 1 . n 0 . n t o 1 0 . n 0 . n t o 8 . n 0 . n t o 6 . n 0 . n t o 4 . n 0 . n t o 2 . n 0 . n 0 . n t o 5 . n 0 . n t o 3 . n 0 . n t o 1 . n 0 . n W a y s 1 6 1 4 1 2 1 0 8 6 4 2 7 2 1 1 9 7 5 3 1 3 6 6 4 2 1 2 1
W + X + Y + Z = 7 2 + 3 6 + 1 2 + 1 = 1 2 1 5 6 ∗ 1 2 1 = 6 7 7 6 .
Details shown in table ABC could be shortened as in WXYZ and 1 $ column can be eliminated.
L
e
t
n
.
m
m
e
a
n
s
n
o
f
m
$
a
n
d
n
.
c
o
i
n
m
e
a
n
s
n
o
f
g
i
v
e
n
c
o
i
n
.
S
i
n
c
e
3
$
o
f
5
3
$
w
i
l
l
b
e
i
n
c
l
u
d
e
d
i
n
a
l
l
p
a
y
m
e
n
t
s
,
s
u
f
f
i
c
e
t
o
l
o
o
k
a
t
t
h
e
w
a
y
s
t
o
p
a
y
5
0
$
.
S
i
m
i
l
a
r
l
y
s
u
f
f
i
c
e
t
o
c
o
n
s
i
d
e
r
o
n
l
y
7
5
c
e
n
t
s
,
s
i
n
c
e
a
l
l
p
a
y
m
e
n
t
s
w
i
l
l
h
a
v
e
3
p
e
n
n
i
e
s
i
n
i
t
.
D
i
v
i
d
e
5
3
$
p
a
y
m
e
n
t
s
i
n
t
o
t
h
r
e
e
g
r
o
u
p
s
A
,
B
,
C
,
a
n
d
7
8
c
e
n
t
s
i
n
t
o
f
o
u
r
g
r
o
u
p
s
W
,
X
,
Y
,
Z
.
m
g
r
o
u
p
i
s
t
h
e
n
u
m
b
e
r
o
f
5
$
b
i
l
l
s
w
h
e
n
i
n
t
h
e
g
r
o
u
p
,
c
o
n
t
r
i
b
u
t
i
o
n
o
f
2
0
l
s
+
m
g
r
o
u
p
.
5
+
3
.
1
=
5
3
$
.
m
g
r
o
u
p
i
s
t
h
e
n
u
m
b
e
r
o
f
n
i
c
k
e
l
s
w
h
e
n
i
n
t
h
e
g
r
o
u
p
,
c
o
n
t
r
i
b
u
t
i
o
n
o
f
Q
u
a
r
t
e
r
s
+
m
g
r
o
u
p
.
5
+
3
.
1
=
7
8
c
e
n
t
s
.
A
.
.
.
.
.
I
t
c
o
n
t
a
i
n
s
n
o
2
0
$
b
i
l
l
s
:
−
.
.
.
.
∴
0
$
≤
(
t
.
1
0
+
f
.
5
)
≤
5
0
$
i
n
m
u
l
t
i
p
l
e
s
o
f
5
$
+
1
$
b
i
l
l
s
=
5
3
$
.
0
≤
t
≤
5
i
s
t
h
e
r
a
n
g
e
o
f
1
0
$
b
i
l
l
s
.
0
≤
f
≤
1
0
i
s
t
h
e
r
a
n
g
e
o
f
5
$
b
i
l
l
s
.
m
A
=
1
0
.
B
.
.
.
.
.
I
t
c
o
n
t
a
i
n
s
o
n
e
2
0
$
b
i
l
l
.
∴
0
$
≤
(
t
.
1
0
+
f
5
)
≤
3
0
$
i
n
m
u
l
t
i
p
l
e
s
o
f
5
$
+
1
$
b
i
l
l
s
=
5
3
$
.
0
≤
t
≤
3
i
s
t
h
e
r
a
n
g
e
o
f
1
0
$
b
i
l
l
s
.
0
≤
f
≤
6
i
s
t
h
e
r
a
n
g
e
o
f
5
$
b
i
l
l
s
.
m
B
=
6
.
C
.
.
.
.
I
t
c
o
n
t
a
i
n
s
t
w
o
2
0
$
b
i
l
l
s
:
∴
0
$
≤
(
t
.
1
0
+
f
5
)
≤
1
0
$
i
n
m
u
l
t
i
p
l
e
s
o
f
5
$
,
+
1
$
b
i
l
l
s
=
5
3
$
.
0
≤
t
≤
1
i
s
t
h
e
r
a
n
g
e
o
f
1
0
$
b
i
l
l
s
.
0
≤
f
≤
2
i
s
t
h
e
r
a
n
g
e
o
f
5
$
b
i
l
l
s
.
m
C
=
2
.
W
.
.
.
.
.
I
t
c
o
n
t
a
i
n
s
n
o
Q
u
a
r
t
e
r
s
:
−
∴
0
c
e
n
t
≤
(
t
.
d
i
m
e
+
f
.
n
i
c
k
e
l
)
≤
7
5
c
e
n
t
i
n
m
u
l
t
i
p
l
e
s
o
f
5
c
e
n
t
s
+
p
e
n
n
i
e
s
=
7
8
c
e
n
t
s
.
0
≤
t
≤
7
i
s
t
h
e
r
a
n
g
e
o
f
d
i
m
e
s
.
0
≤
f
≤
1
5
i
s
t
h
e
r
a
n
g
e
o
f
n
i
c
k
e
l
s
.
m
W
=
1
5
.
X
.
.
.
.
.
I
t
c
o
n
t
a
i
n
s
o
n
e
Q
u
a
r
t
e
r
:
−
∴
0
c
e
n
t
≤
(
t
.
d
i
m
e
+
f
.
n
i
c
k
e
l
)
≤
5
0
c
e
n
t
i
n
m
u
l
t
i
p
l
e
s
o
f
5
c
e
n
t
s
+
p
e
n
n
i
e
s
=
7
8
c
e
n
t
s
.
0
≤
t
≤
5
i
s
t
h
e
r
a
n
g
e
o
f
d
i
m
e
s
.
0
≤
f
≤
1
0
i
s
t
h
e
r
a
n
g
e
o
f
n
i
c
k
e
l
s
.
m
X
=
1
0
.
Y
.
.
.
.
.
I
t
c
o
n
t
a
i
n
s
t
w
o
Q
u
a
r
t
e
r
s
:
−
∴
0
c
e
n
t
≤
(
t
.
d
i
m
e
+
f
.
n
i
c
k
e
l
)
≤
2
5
c
e
n
t
i
n
m
u
l
t
i
p
l
e
s
o
f
5
c
e
n
t
s
+
p
e
n
n
i
e
s
=
7
8
c
e
n
t
s
.
0
≤
t
≤
2
i
s
t
h
e
r
a
n
g
e
o
f
d
i
m
e
s
.
0
≤
f
≤
5
i
s
t
h
e
r
a
n
g
e
o
f
n
i
c
k
e
l
s
.
m
Y
=
1
5
.
Z
.
.
.
.
.
I
t
c
o
n
t
a
i
n
s
t
h
r
e
e
Q
u
a
r
t
e
r
s
:
−
S
o
o
n
l
y
o
n
e
w
a
y
o
f
p
a
y
m
e
n
t
,
3
.
Q
u
a
r
t
e
r
+
3
.
p
e
n
n
i
e
s
.
m
Z
=
1
.
N
t
h
e
n
u
m
b
e
r
o
f
w
a
y
s
w
e
c
a
n
p
a
y
,
d
e
p
e
n
d
s
o
n
m
g
r
o
u
p
.
F
o
r
e
v
e
n
m
g
r
o
u
p
,
N
=
(
2
m
g
r
o
u
p
+
1
)
2
.
F
o
r
o
d
d
m
g
r
o
u
p
,
N
=
(
2
m
g
r
o
u
p
+
1
)
2
+
2
m
g
r
o
u
p
+
1
N
A
=
(
2
1
0
+
1
)
2
=
3
6
N
B
=
(
2
6
+
1
)
2
=
1
6
.
N
C
=
(
2
2
+
1
)
2
=
4
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
N
5
3
=
3
6
+
1
6
+
4
=
5
6
.
N
W
=
(
2
1
5
+
1
)
2
+
2
1
5
+
1
=
6
4
+
8
=
7
2
N
X
=
(
2
1
0
+
1
)
2
=
3
6
.
N
Y
=
(
2
5
+
1
)
2
+
2
5
+
1
=
9
+
3
=
1
2
N
Z
=
1
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
N
7
8
=
7
2
+
3
6
+
1
2
+
1
=
1
2
1
.
N
5
6
∗
1
2
1
=
6
7
7
6