Pentagons and circles
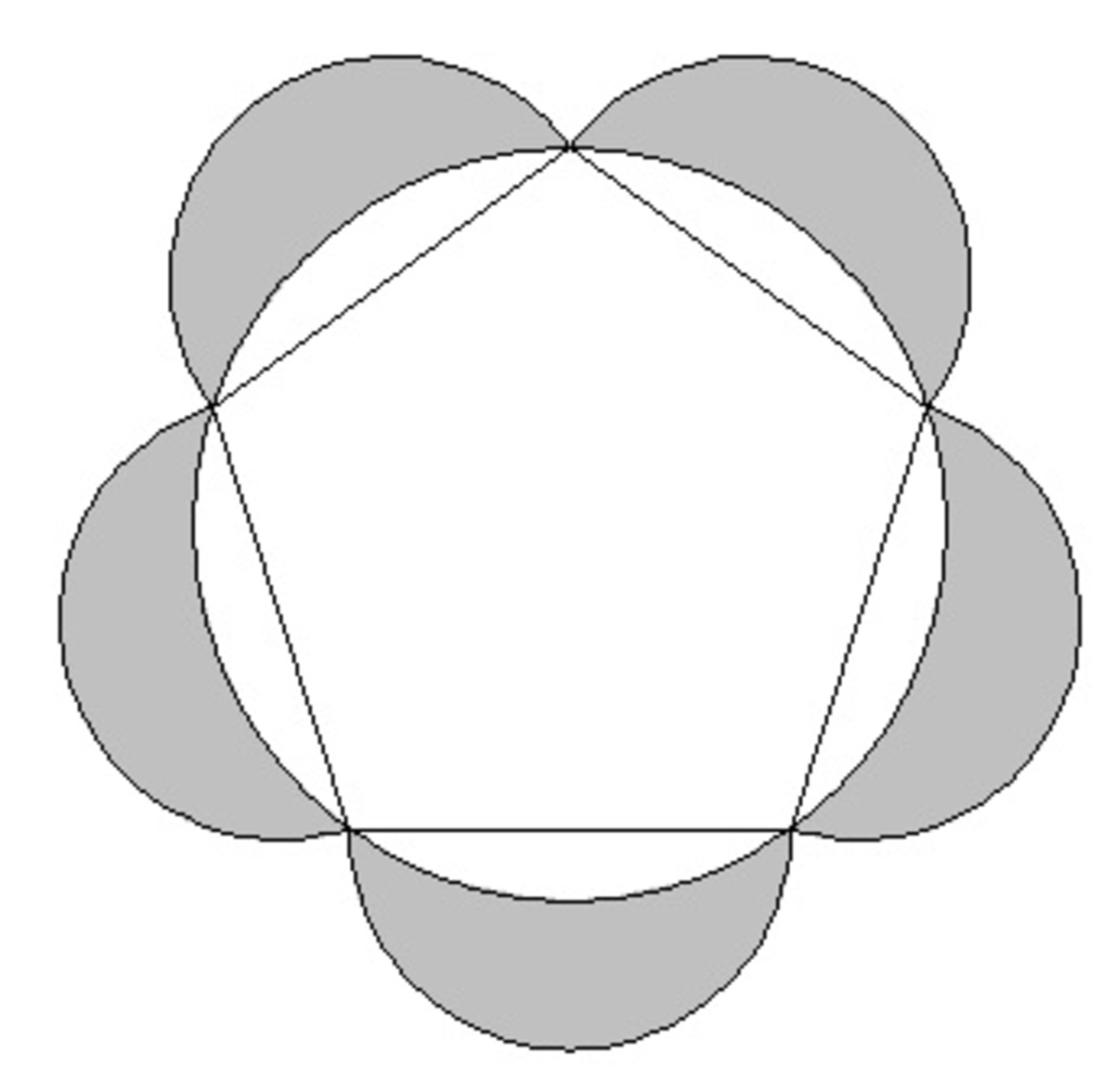 A regular pentagon is inscribed in a circle with diameter
. Five semicirlces are then constructed with their diameters on each of the sides of the pentagon. Which of the following is the most approximate area of the shaded portion? Use
.
A regular pentagon is inscribed in a circle with diameter
. Five semicirlces are then constructed with their diameters on each of the sides of the pentagon. Which of the following is the most approximate area of the shaded portion? Use
.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let s be the area of one lune and x be the length of one side of the pentagon.
A s e c t o r = 3 6 0 θ π r 2 = 3 6 0 7 2 ( 7 2 2 ) ( 1 0 2 ) = 6 2 . 8 5 7
A s e g m e n t = A s e c t o r − A t r i a n g l e = 6 2 . 8 5 7 − 2 1 ( 1 0 2 ) ( sin 7 2 ) = 1 5 . 3 0 4
By cosine law, x 2 = 1 0 2 + 1 0 2 − 2 ( 1 0 ) ( 1 0 ) ( cos 7 2 ) , from here x = 1 1 . 7 5 6
s = a r e a o f s m a l l s e m i c r c l e − A s e g m e n t = 2 1 ( 7 2 2 ) ( 2 1 1 . 7 5 6 ) 2 − 1 5 . 3 0 4 = 3 8 . 9 9
Finally,
5 s = 5 ( 3 8 . 9 9 ) ≈ 1 9 5