Pentagonal Puzzle with Pythagorus
Geometry
Level
pending
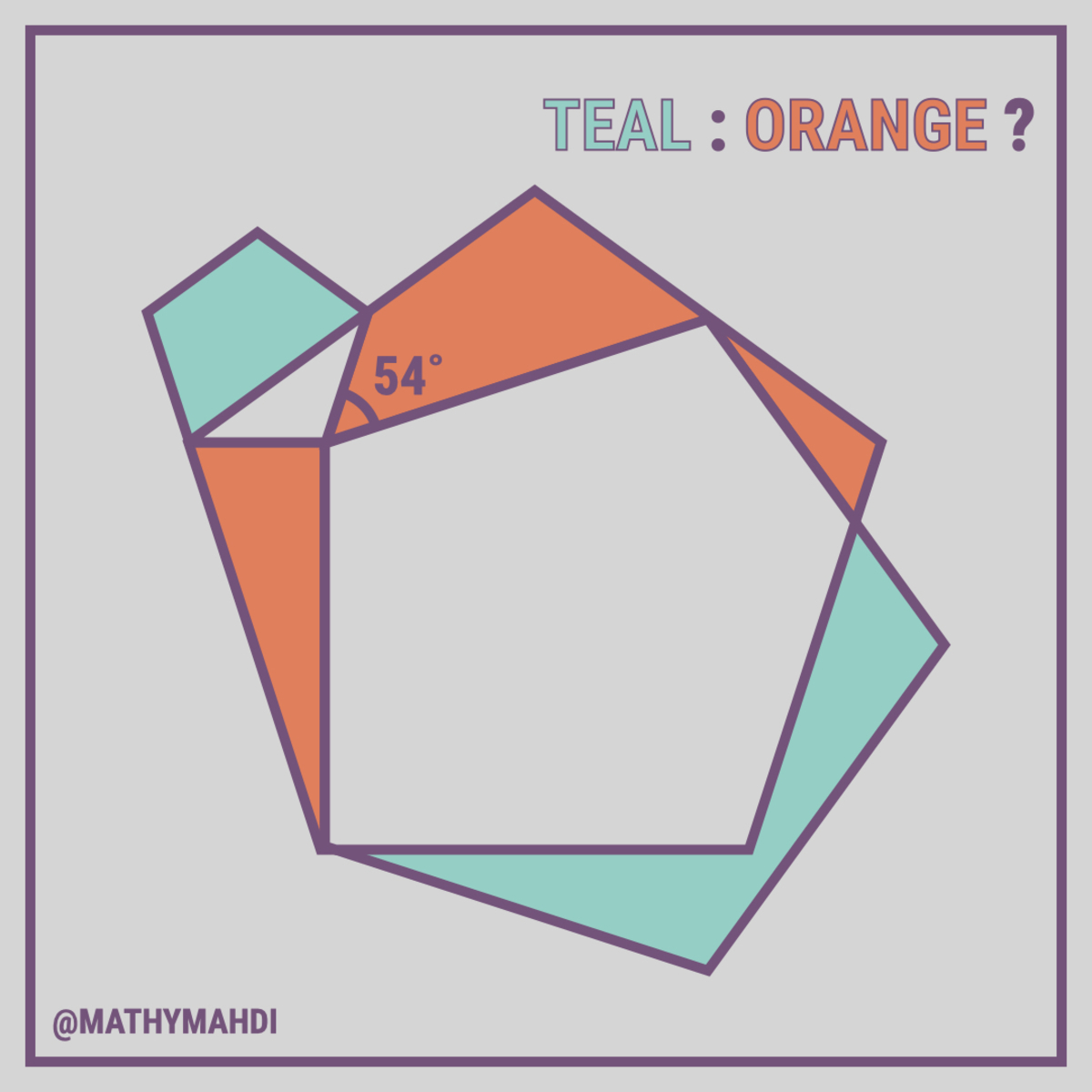
3 regular pentagons overlap as such. The two teal pentagons intersect at an angle of 54˚. What is the ratio of the sum of Teal regions to the sum of Orange regions?
The answer is 1.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Label the regions in the diagram as follows:
At the vertex of the 5 4 ° angle, the two regular polygon angles are 1 0 8 ° (from regions G 1 and G 2 ), which makes the missing angle on O 1 equal to 3 6 0 ° − 5 4 ° − 2 ⋅ 1 0 4 ° = 9 0 ° , so that O 1 is a right triangle.
Each of the three different regular pentagons also share one side of right triangle O 1 , and by the Pythagorean Theorem, these three sides have the relation a 2 + b 2 = c 2 , and since the ratio of the areas of similar polygons is equal to the ratio of the square of its sides, the area of the small pentagon plus the area of the medium pentagon is equal to the area of the big pentagon, or:
( T 1 + G 1 ) + ( G 2 + T 2 ) = ( O 1 + O 2 + O 3 + G 1 + G 2 )
which solves to:
T 1 + T 2 = O 1 + O 2 + O 3
In other words, the sum of the areas of the teal regions is equal to the sum of the areas of the orange regions, which makes the ratio of their areas equal to 1 .